A simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
3mg
4mg
5mg
6mg
The correct Answer is:D
To solve the problem of finding the tension in the string at the lowest point of a simple pendulum given the minimum horizontal speed necessary for it to move in a circular path, we can follow these steps:
1. Identify the parameters:
- Length of the pendulum: l
- Mass of the bob: m
- Gravitational acceleration: g
2. Determine the minimum speed:
The minimum horizontal speed v required for the bob to move in a circular path is given by the formula:
v=√gl
This is the speed necessary to maintain circular motion at the top of the swing.
3. Draw the free body diagram:
At the lowest point of the swing, the forces acting on the bob are:
- The weight of the bob acting downwards: mg
- The tension in the string acting upwards: T
4. Apply Newton's second law:
At the lowest point, the net force acting on the bob provides the centripetal force required for circular motion. Therefore, we can write:
T−mg=mv2l
where mv2l is the centripetal force.
5. Substitute the expression for v:
Substitute v=√gl into the centripetal force equation:
T−mg=m(√gl)2l
Simplifying this gives:
T−mg=mgll=mg
6. Solve for tension T:
Rearranging the equation gives:
T=mg+mg=2mg
7. Final result:
The tension in the string at the lowest point of the bob is:
T=2mg
- Length of the pendulum: l
- Mass of the bob: m
- Gravitational acceleration: g
The minimum horizontal speed v required for the bob to move in a circular path is given by the formula:
v=√gl
This is the speed necessary to maintain circular motion at the top of the swing.
At the lowest point of the swing, the forces acting on the bob are:
- The weight of the bob acting downwards: mg
- The tension in the string acting upwards: T
At the lowest point, the net force acting on the bob provides the centripetal force required for circular motion. Therefore, we can write:
T−mg=mv2l
where mv2l is the centripetal force.
Substitute v=√gl into the centripetal force equation:
T−mg=m(√gl)2l
Simplifying this gives:
T−mg=mgll=mg
Rearranging the equation gives:
T=mg+mg=2mg
The tension in the string at the lowest point of the bob is:
T=2mg
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 1 Subjective
13 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 Single Correct
18 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Assertion And Reason
28 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
The string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionA simple pendulum of length l and bob of mass m is displaced from its equilibrium position O to a position P so that hight of P above O is h . If is then released. What is the tension in the string when the bob passes through the through the equilibrium position O ? Neglect friction. v is the velocity of the bob at O
View SolutionA simple pendulum of length L and mass (bob) M is oscillating in a plane about a vertical line between angular limit −ϕ and +ϕ. For an angular displacement θ(|θ|<ϕ), the tension in the string and the velocity of the bob are T and V respectively. The following relations hold good under the above conditions:
View SolutionThe bob of a simple pendulum of length 1 m has mass 100 g and a speed of 1.4 m/s at the lowest point in its path. Find the tension in the stirng at this instant.
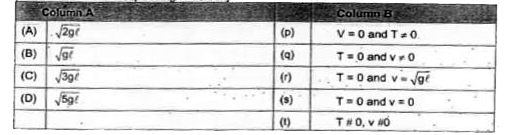
View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
View SolutionA simple pendulm has a length L and a bob of mass M. The bob is vibrating with amplitude a .What is the maximum tension in the string?
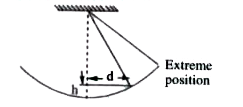
View SolutionA simple pendulum of length l and mass m free to oscillate in vertical plane. A nail is located at a distance d=l−a vertically below the point of suspension of a simple pendulum. The pendulum bob is released from the position where the string makes an angle of 90∘ from vertical. Discuss the motion of the bob if (a) l=2a and (b) l=2.5a.
View SolutionA simple pendulum of length 1 oscillates aboμt the mean position as shown in the figure. If the total energy of the pendulum is E, the velocity of the pendulum bob of mass m at point P is:
View SolutionA simple pendulum of length l and mass m is suspended in a car that is moving with constant speed v around a circle of radius r. Find the period of oscillation and equilibrium position of the pendulum.
View Solution