Four charges +q,−q,+q and −q are placed in order on the four consecutive corners of a square of side a. The work done in interchanging the positions of any two neighboring charges of the opposite sign is
q24πε0a(−4+√2)
q24πε0a(4+2√2)
q24πε0a(4−2√2)
q24πε0a(4+√2)
The correct Answer is:C
To find the work done in interchanging the positions of any two neighboring charges of opposite signs in the given configuration, we will calculate the initial and final potential energy of the system and then determine the work done based on the change in potential energy.
1. Identify the Initial Configuration:
The charges are arranged at the corners of a square:
- Corner 1: +q
- Corner 2: -q
- Corner 3: +q
- Corner 4: -q
2. Calculate the Initial Potential Energy (U_initial):
- The potential energy between two charges q1 and q2 separated by a distance r is given by:
U=kq1q2r
- For neighboring charges (e.g., +q and -q), the distance is a.
- For diagonal charges (e.g., +q and +q), the distance is a√2.
- The contributions to the initial potential energy are:
- For the pairs of neighboring charges:
Uneighboring=−kq2a−kq2a−kq2a−kq2a=−4kq2a
- For the pairs of diagonal charges:
Udiagonal=kq2a√2+kq2a√2=2kq2a√2
- Therefore, the total initial potential energy is:
Uinitial=−4kq2a+2kq2a√2
3. Identify the Final Configuration After Interchanging Charges:
After interchanging the positions of two neighboring charges of opposite signs, the new arrangement will be:
- Corner 1: +q
- Corner 2: +q
- Corner 3: -q
- Corner 4: -q
4. Calculate the Final Potential Energy (U_final):
- The contributions to the final potential energy are:
- For the pairs of neighboring charges (now both +q):
Uneighboring=0(since they are like charges)
- For the pairs of neighboring charges (now both -q):
Uneighboring=0(since they are like charges)
- For the pairs of diagonal charges (one +q and one -q):
Udiagonal=−kq2a√2−kq2a√2=−2kq2a√2
- Therefore, the total final potential energy is:
Ufinal=0+0−2kq2a√2=−2kq2a√2
5. Calculate the Work Done (W):
The work done by the external agent is equal to the change in potential energy:
W=Ufinal−Uinitial
Substituting the values:
W=(−2kq2a√2)−(−4kq2a+2kq2a√2)
Simplifying this expression:
W=−2kq2a√2+4kq2a−2kq2a√2=4kq2a−4kq2a√2
Factoring out common terms:
W=kq2a(4−2√2)
Final Answer:
The work done in interchanging the positions of any two neighboring charges of opposite sign is:
W=kq2a(4−2√2)
The charges are arranged at the corners of a square:
- Corner 1: +q
- Corner 2: -q
- Corner 3: +q
- Corner 4: -q
- The potential energy between two charges q1 and q2 separated by a distance r is given by:
U=kq1q2r
- For neighboring charges (e.g., +q and -q), the distance is a.
- For diagonal charges (e.g., +q and +q), the distance is a√2.
- The contributions to the initial potential energy are:
- For the pairs of neighboring charges:
Uneighboring=−kq2a−kq2a−kq2a−kq2a=−4kq2a
- For the pairs of diagonal charges:
Udiagonal=kq2a√2+kq2a√2=2kq2a√2
- Therefore, the total initial potential energy is:
Uinitial=−4kq2a+2kq2a√2
After interchanging the positions of two neighboring charges of opposite signs, the new arrangement will be:
- Corner 1: +q
- Corner 2: +q
- Corner 3: -q
- Corner 4: -q
- The contributions to the final potential energy are:
- For the pairs of neighboring charges (now both +q):
Uneighboring=0(since they are like charges)
- For the pairs of neighboring charges (now both -q):
Uneighboring=0(since they are like charges)
- For the pairs of diagonal charges (one +q and one -q):
Udiagonal=−kq2a√2−kq2a√2=−2kq2a√2
- Therefore, the total final potential energy is:
Ufinal=0+0−2kq2a√2=−2kq2a√2
The work done by the external agent is equal to the change in potential energy:
W=Ufinal−Uinitial
Substituting the values:
W=(−2kq2a√2)−(−4kq2a+2kq2a√2)
Simplifying this expression:
W=−2kq2a√2+4kq2a−2kq2a√2=4kq2a−4kq2a√2
Factoring out common terms:
W=kq2a(4−2√2)
The work done in interchanging the positions of any two neighboring charges of opposite sign is:
W=kq2a(4−2√2)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Knowledge Check
Four equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
AzeroB√2q2πε0aC√2qπε0aDq2πε0a
Three charges, each equal to q, are placed at the three. corners of a square of side a . Find the electric field at. the fourth corner.
View SolutionThree charges, each equal to q, are placed at the three. corners of a square of side a . Find the electric field at. the fourth corner.
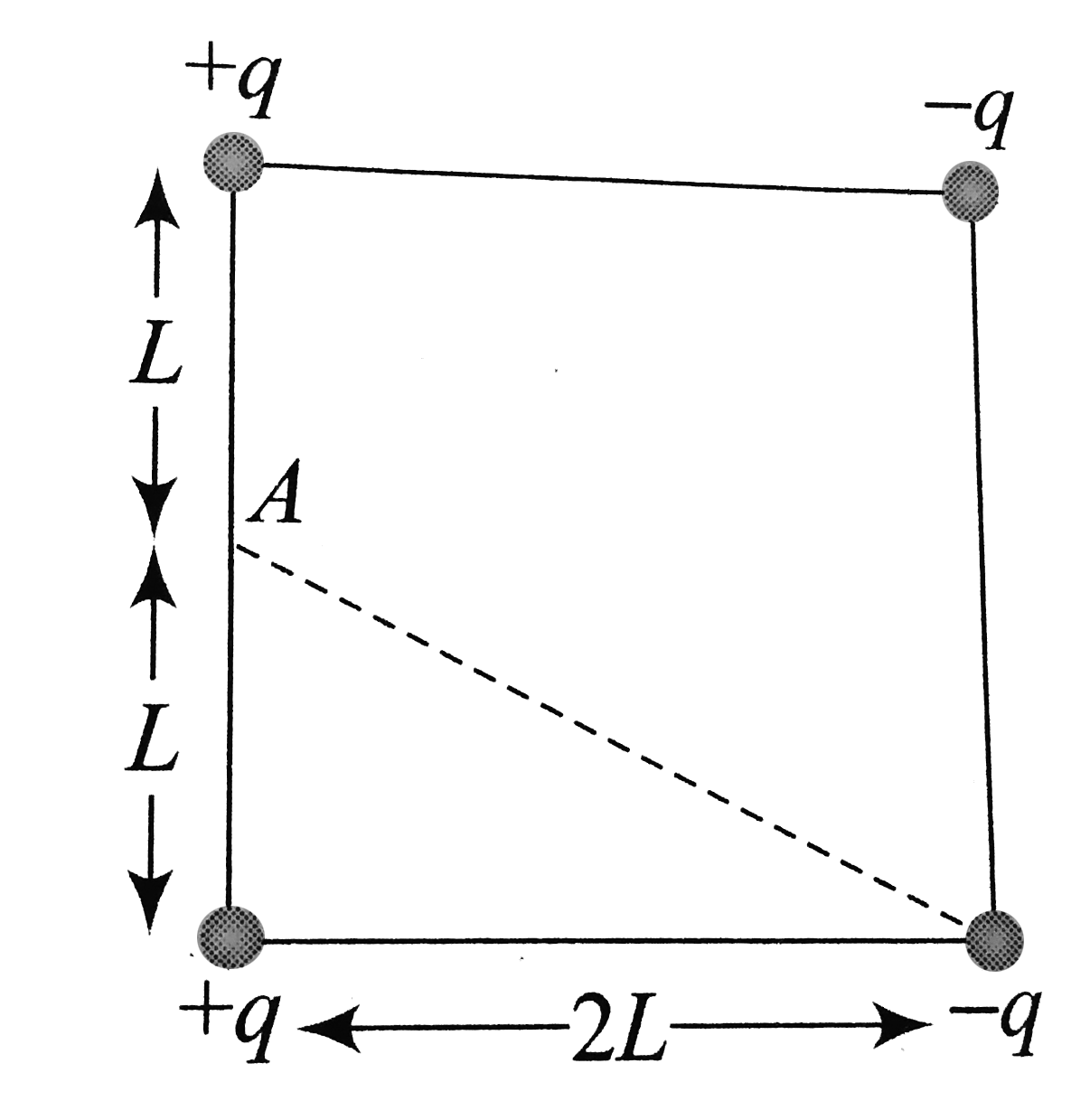
View SolutionFour electric charges +q,+q,−q and −q are placed at the corners of a square of side 2L (see figure). The electric potential at point A, mid-way between the two charges +q and +q, is
View SolutionFour charges +q,+q,−q,and−q are placed, respectively, at the corners A,B,C,andD of a square of side a, arranged in the given order. EandF are the midpoints of sides BCandCD, respectively, O is the center of square.
The work done in carrying a charge e from O to F is.
View SolutionFour charges +q,+q,−q,and−q are placed, respectively, at the corners A,B,C,andD of a square of side a, arranged in the given order. EandF are the midpoints of sides BCandCD, respectively, O is the center of square.
The work done in carrying a charge e from O to E is.
View SolutionFour charges +q,+q,−q,and−q are placed, respectively, at the corners A,B,C,andD of a square of side a, arranged in the given order. EandF are the midpoints of sides BCandCD, respectively, O is the center of square.
The electric potential at O is.
View SolutionFour charges +q,+q,−q,and−q are placed, respectively, at the corners A,B,C,andD of a square of side a, arranged in the given order. If O is the center of square.
The electric field at O is.
View Solution
