Two condensers and each having slabs of dielectric constant are connected in series. When they are connected across supply, potential difference across is and that across B is . If the dielectric in the condenser of smaller capacitance is replaced by one for which , what will be the values of potential difference across them?
To solve the problem step by step, we will analyze the capacitors A and B connected in series and how the change in dielectric affects the potential difference across them.
Step 1: Understand the relationship between capacitance and dielectric constant
The capacitance C of a capacitor with a dielectric is given by:
C=K⋅C0
where K is the dielectric constant and C0 is the capacitance without the dielectric.
Step 2: Set up the relationship for capacitors in series
For capacitors in series, the total voltage V is the sum of the voltages across each capacitor:
V=VA+VB
Given that V=230V, VA=130V, and VB=100V.
Step 3: Determine the capacitance ratio
From the voltage across the capacitors, we can establish the ratio of their capacitances:
VAVB=CBCA
Substituting the known values:
130100=CBCA⟹CACB=100130=1013
Step 4: Replace the dielectric in the capacitor with smaller capacitance
Assume CA has the smaller capacitance. When the dielectric constant of CA is changed from K=2 to K=5, the new capacitance C′A can be calculated as:
C′A=KnewKold⋅CA=52⋅CA=2.5⋅CA
Step 5: Update the capacitance ratio
Now, substituting C′A into the capacitance ratio:
C′ACB=2.5⋅CACB
Using the previous ratio CACB=1013:
C′ACB=2.5⋅1013⋅CBCB=2513
Step 6: Find the new voltage across each capacitor
Using the new capacitance ratio, we can find the new voltage across each capacitor:
V′AV′B=CBC′A=1325
Let V′A=x and V′B=y. Then:
xy=1325⟹25x=13y
Also, since V′A+V′B=230:
x+y=230
Step 7: Solve the equations
From 25x=13y, we can express y in terms of x:
y=2513x
Substituting into the total voltage equation:
x+2513x=230
Combining terms:
3813x=230⟹x=230⋅1338=78.68V
Now, substituting back to find y:
y=230−78.68=151.32V
Final Answer
The new potential differences across capacitors A and B are:
- V′A=78.68V
- V′B=151.32V
---
The capacitance C of a capacitor with a dielectric is given by:
C=K⋅C0
where K is the dielectric constant and C0 is the capacitance without the dielectric.
For capacitors in series, the total voltage V is the sum of the voltages across each capacitor:
V=VA+VB
Given that V=230V, VA=130V, and VB=100V.
From the voltage across the capacitors, we can establish the ratio of their capacitances:
VAVB=CBCA
Substituting the known values:
130100=CBCA⟹CACB=100130=1013
Assume CA has the smaller capacitance. When the dielectric constant of CA is changed from K=2 to K=5, the new capacitance C′A can be calculated as:
C′A=KnewKold⋅CA=52⋅CA=2.5⋅CA
Now, substituting C′A into the capacitance ratio:
C′ACB=2.5⋅CACB
Using the previous ratio CACB=1013:
C′ACB=2.5⋅1013⋅CBCB=2513
Using the new capacitance ratio, we can find the new voltage across each capacitor:
V′AV′B=CBC′A=1325
Let V′A=x and V′B=y. Then:
xy=1325⟹25x=13y
Also, since V′A+V′B=230:
x+y=230
From 25x=13y, we can express y in terms of x:
y=2513x
Substituting into the total voltage equation:
x+2513x=230
Combining terms:
3813x=230⟹x=230⋅1338=78.68V
Now, substituting back to find y:
y=230−78.68=151.32V
The new potential differences across capacitors A and B are:
- V′A=78.68V
- V′B=151.32V
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE1videos
CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE1videos
CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY42videos
ATOMS
- BOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective11videos
COMMUNICATION SYSTEM
Similar Questions
Potential difference across AB , i.e., VA−VB is
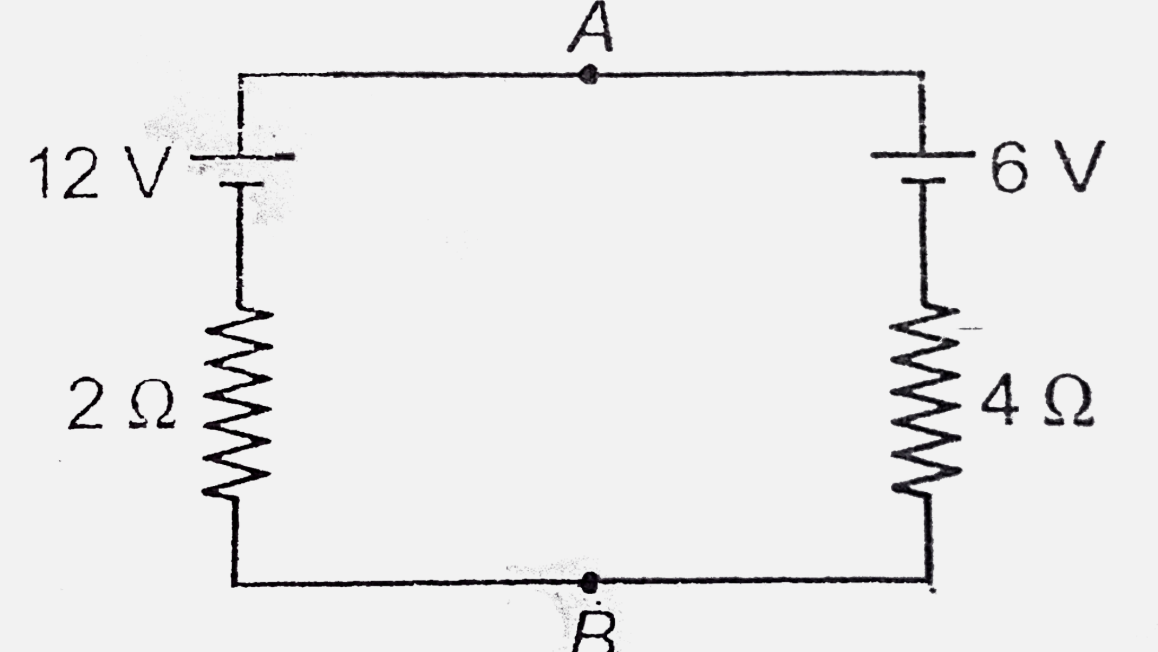 View Solution
View SolutionA 220 V AC supply is connected between points A and B . What will be the potential difference V across the capacitor ?
 View Solution
View SolutionA 220 V AC supply is connected between points A and B . What will be the potential difference V across the capacitor ?
 View Solution
View SolutionA 220 V AC supply is connected between points A and B . What will be the potential difference V across the capacitor ?
 View Solution
View SolutionTwo capacitances of capacity C1and C2 are connected in series and potential difference V is applied across it. Then the potential difference across C1 will be
View SolutionA 220 V A supply is connected between points A and B in figure. What will be the potential difference V across the capacitor?
View SolutionA resistor and a capacitor are connected in series with an a.c. source. If the potential drop across the capacitor is 5 V and that across resistor is 12 V, applied voltage is
View SolutionTwo electric bulbs one, if 200 V-40 W and other 200 V-100 W are connected in series to a 200 V line, then the potential drop across
View SolutionThree capacitors 3μF,6μF and 6μF are connected in series to source of 120 V. The potential difference in volt, across the 3μF capacitor will be
View SolutionTwo, capacitors A and B are connected in series across a 100V supply and it is observed that the potential difference across them are 60V and 40V. A capacitor of 2pF capacitance is now connected in parallel with A and the potential difference across B rises to 90V. Determine the capacitance of A and B
View Solution

