Question

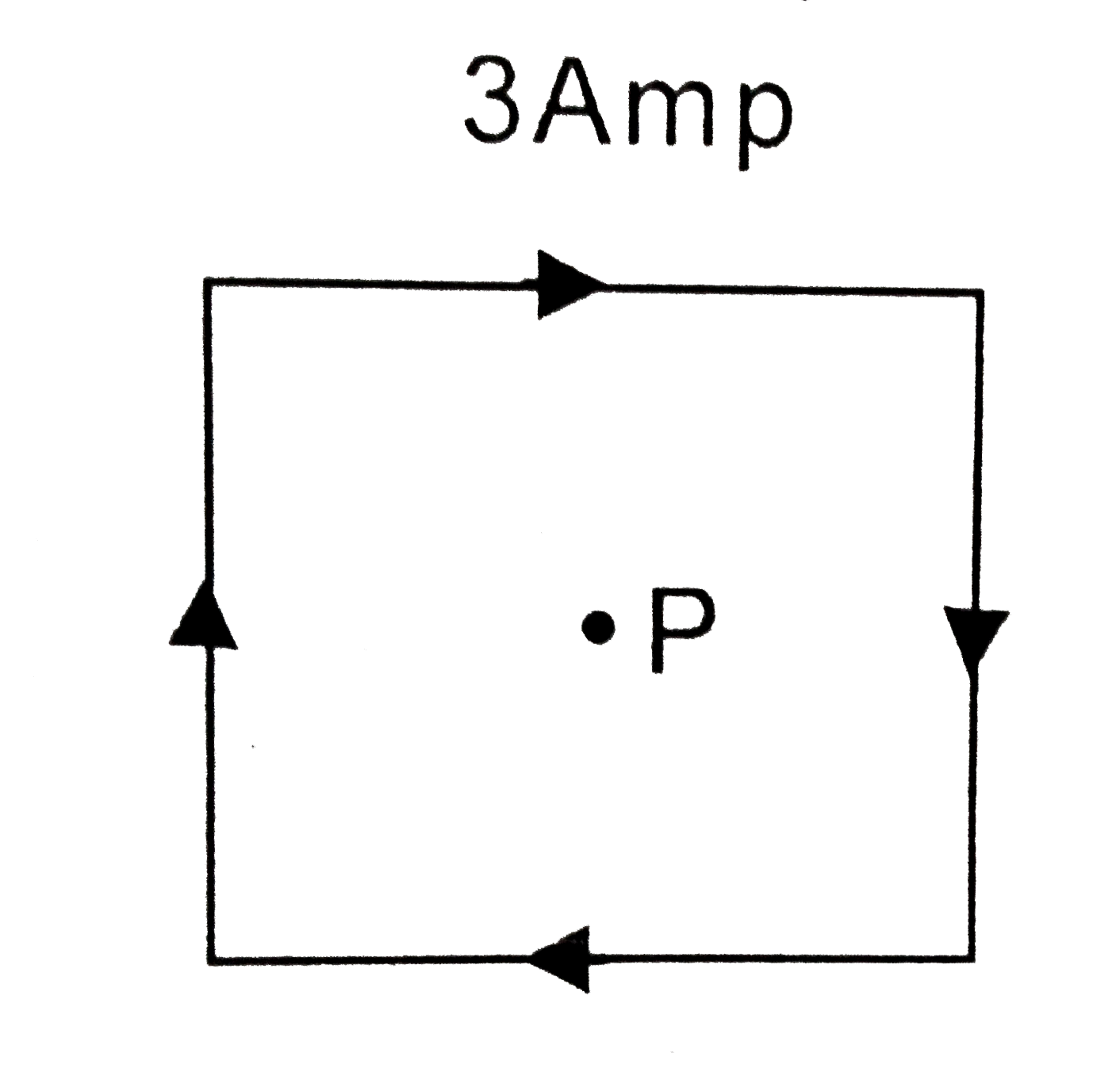
A square loop of side $6cm$ carries a current of $30A$. Calculate the magnitude of magnetic field $B$ at a point $P$ lying on the axis of the loop and a distance$\sqrt{7}$ cm from centre of the loop.

Answer

A::B::D

Explanation

To solve the problem, we will calculate the magnetic field $$B$$ at point $$P$$ due to a square loop carrying a current. The steps are as follows:

Step 1: Understand the Geometry
We have a square loop with a side length of \( 6 \, \text{cm} \) and a current of \( 30 \, \text{A} \). The point \( P \) is located on the axis of the loop at a distance of \( \sqrt{7} \, \text{cm} \) from the center of the loop.

Step 2: Calculate the Distance \( R \)
The distance \( R \) from a corner of the square loop to point \( P \) can be calculated using the Pythagorean theorem. The distance from the center of the loop to the corner is \( \frac{6}{2} = 3 \, \text{cm} \) (half the side length). Therefore, the total distance \( R \) is given by:

\( R = \sqrt{(3 \, \text{cm})^2 + (\sqrt{7} \, \text{cm})^2} \)

Calculating this:

\( R = \sqrt{9 + 7} = \sqrt{16} = 4 \, \text{cm} \)

Step 3: Calculate the Magnetic Field \( B \) for One Side
The magnetic field \( B \) at point \( P \) due to one side of the square loop can be calculated using the formula:

\( B = \frac{\mu_0 I}{4\pi R} \cdot 2 \sin \alpha \)

Where:
- \( \mu_0 = 4\pi \times 10^{-7} \, \text{T m/A} \) (permeability of free space)
- \( I = 30 \, \text{A} \)
- \( R = 4 \, \text{cm} = 4 \times 10^{-2} \, \text{m} \)

To find \( \sin \alpha \), we note that in our triangle formed, \( \sin \alpha = \frac{3}{5} \) (opposite over hypotenuse).

Now substituting the values:

\( B = \frac{(4\pi \times 10^{-7}) \cdot 30}{4\pi \cdot (4 \times 10^{-2})} \cdot 2 \cdot \frac{3}{5} \)

Simplifying this:

\( B = \frac{30 \times 10^{-7}}{4 \times 10^{-2}} \cdot 2 \cdot \frac{3}{5} \)

Calculating \( B \):

\( B = \frac{30 \times 10^{-7}}{0.04} \cdot \frac{6}{5} = \frac{30 \times 10^{-7} \cdot 6}{0.2} = 9 \times 10^{-5} \, \text{T} \)

Step 4: Calculate the Total Magnetic Field
Since there are four sides in the square loop, the total magnetic field \( B_{\text{total}} \) is:

\( B_{\text{total}} = 4B \sin \theta \)

Where \( \theta \) is the angle at point \( P \). In this case, \( \sin \theta = \frac{3}{4} \).

Substituting the values:

\( B_{\text{total}} = 4 \cdot (9 \times 10^{-5}) \cdot \frac{3}{4} \)

Calculating this gives:

\( B_{\text{total}} = 3 \times 10^{-4} \, \text{T} = 2.7 \times 10^{-4} \, \text{T} \)

Final Answer
The magnitude of the magnetic field \( B \) at point \( P \) is:

\( B = 2.7 \times 10^{-4} \, \text{T} \)

---