A man of height 2.0m is standing on a level road because of temprature variation the refractive index of air is varying as μ=√1+ay, where y is height from road. If a=2.0×10−6m−1. Then, find distant point that he can see on the road.
The correct Answer is:A, B
To solve the problem step by step, we will analyze the situation using the given information about the refractive index and the geometry involved.
Step 1: Understanding the Refractive Index Variation
The refractive index of air is given by:
μ=√1+ay
where a=2.0×10−6m−1 and y is the height from the road.
Step 2: Setting Up the Geometry
We have a man of height h=2.0m standing on a level road. We need to find the maximum distance x that he can see on the road. The light rays coming from a distant point on the road will bend due to the varying refractive index.
Step 3: Applying Snell's Law
According to Snell's law:
μ0sinθ=μsin90∘
Here, μ0=1 (for air) and sin90∘=1. Thus,
sinθ=1μ
Substituting the expression for μ:
sinθ=1√1+ay
Step 4: Relating tanθ and Geometry
Using basic trigonometric functions, we have:
tanθ=dxdy
From the previous step:
tanθ=1√1+ay
Thus, we can equate:
dxdy=1√1+ay
Step 5: Rearranging and Integrating
Rearranging gives us:
dx=dy√1+ay
Now we will integrate both sides. The limits for y will be from 0 to 2m (the height of the man), and we want to find x when y=2:
∫x0dx=∫20dy√1+ay
Step 6: Solving the Integral
The integral on the right can be solved using a substitution. Let u=1+ay, then du=ady or dy=dua. The limits change accordingly:
- When y=0, u=1
- When y=2, u=1+2a
Thus, the integral becomes:
x=∫1+2a11√udua=1a(2√u)1+2a1
Calculating this gives:
x=2a(√1+2a−1)
Step 7: Substituting the Value of a
Now, substituting a=2.0×10−6:
x=22.0×10−6(√1+4.0×10−6−1)
Calculating √1+4.0×10−6≈1+2.0×10−6 (using the binomial approximation for small values):
x≈22.0×10−6(2.0×10−6)=2000m
Final Result
The maximum distance that the man can see on the road is:
2000m
The refractive index of air is given by:
μ=√1+ay
where a=2.0×10−6m−1 and y is the height from the road.
We have a man of height h=2.0m standing on a level road. We need to find the maximum distance x that he can see on the road. The light rays coming from a distant point on the road will bend due to the varying refractive index.
According to Snell's law:
μ0sinθ=μsin90∘
Here, μ0=1 (for air) and sin90∘=1. Thus,
sinθ=1μ
Substituting the expression for μ:
sinθ=1√1+ay
Using basic trigonometric functions, we have:
tanθ=dxdy
From the previous step:
tanθ=1√1+ay
Thus, we can equate:
dxdy=1√1+ay
Rearranging gives us:
dx=dy√1+ay
Now we will integrate both sides. The limits for y will be from 0 to 2m (the height of the man), and we want to find x when y=2:
∫x0dx=∫20dy√1+ay
The integral on the right can be solved using a substitution. Let u=1+ay, then du=ady or dy=dua. The limits change accordingly:
- When y=0, u=1
- When y=2, u=1+2a
x=∫1+2a11√udua=1a(2√u)1+2a1
Calculating this gives:
x=2a(√1+2a−1)
Now, substituting a=2.0×10−6:
x=22.0×10−6(√1+4.0×10−6−1)
Calculating √1+4.0×10−6≈1+2.0×10−6 (using the binomial approximation for small values):
x≈22.0×10−6(2.0×10−6)=2000m
The maximum distance that the man can see on the road is:
2000m
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 2 Single Correct
41 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Single Correct Option
3 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 1 Subjective
29 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
A man of height 2 m stands on a straight road on a hot day. The vertical temperature in the air results in a variation of refractive index with height y as μ=μ0√(1+ay) where μ0 is the refractive index of air near the road and a=2×10−6/m. What is the actual length of the road, man is able to see
View SolutionA man of height 1.47 m stands on a straight road on a hot day. The vertical temperature in the air results in a variation of refractive index with height y as μ=μ0√(1+ay) where μ0 is the refractive index of air near the road a =1.5×10−6/m. What is the apparent length of the road man is able to sec?
View SolutionDue to a vertical temperature gradient in the atmosphere, the index of refraction varies. Suppose index of refraction varies as n=n0√1+ay, where n0 is the index of refraction at the surface and a=2.0×10−6m−1 . A person of height h=2.0m stands on a level surface. Beyond what distance will he not see the runway?

View SolutionA ray of light is incident on a glass slab at grazing incidence. The refractive index of the material of the slab is given by μ=√(1+y) . If the thickness of the slab is d=2m, determing the equation of the trajectory of the ray inside the slab and the coordinates of the point where the ray exits from the slab. Take the origin to be at the point of entry of the ray.
View SolutionA ball falls on the ground fro a height of 2.0 m and rebounds up to a height of 1.5 m. Find the coefficient of restitution.
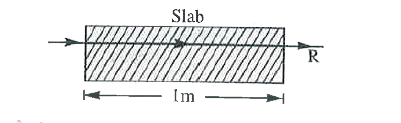
View SolutionThe figure shows a transparent slab of length m placed in air whose refractive index in x direction varies as μ=1+x2(0<x<1). The optical path length of ray R will be
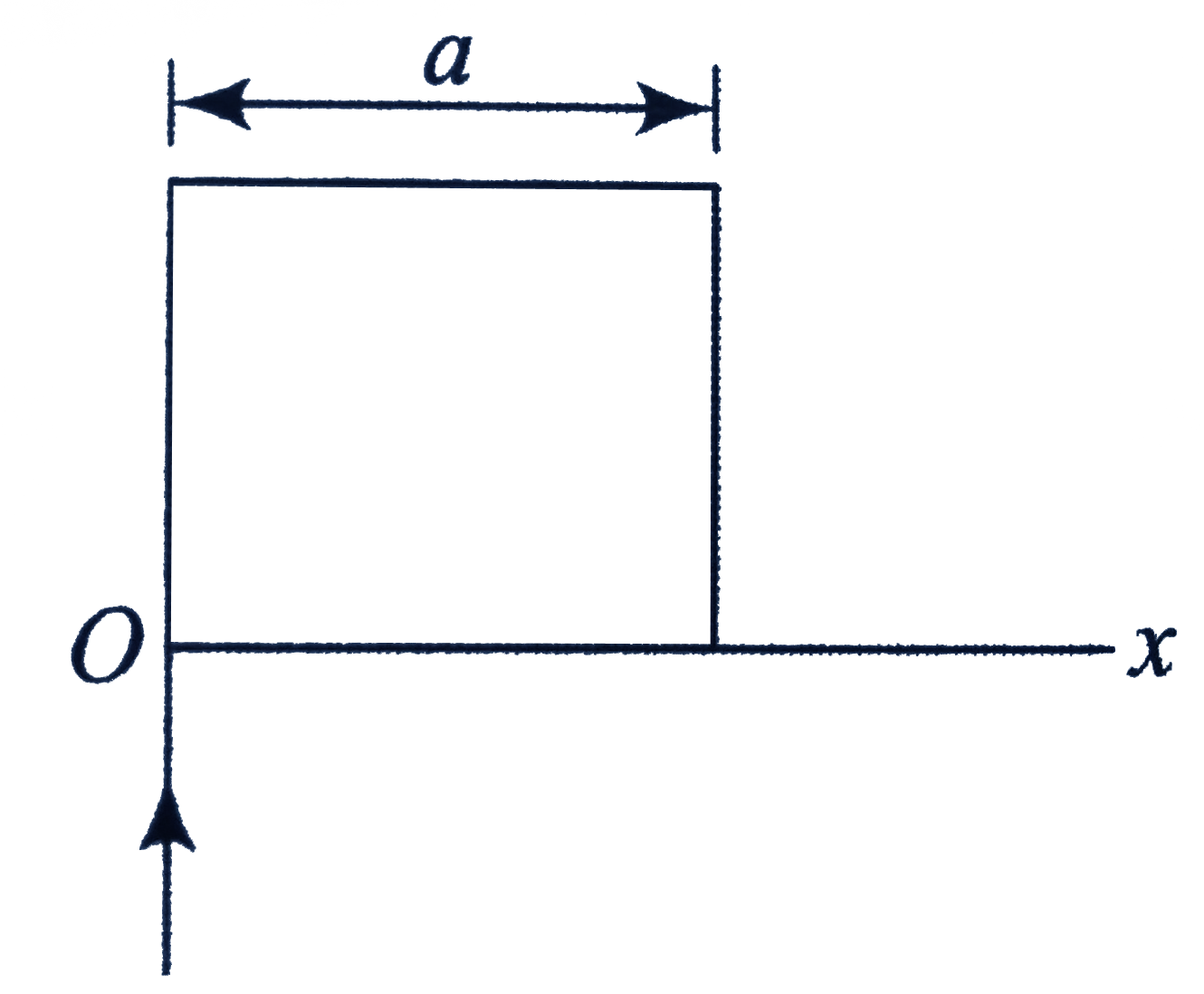
View SolutionThe refractive index of an anisotropic medium varies as μ=μ0√(x+1) , where 0≤x≤a. A ray of light is incident at the origin just along y-axis (shown in figure). Find the equation of ray in the medium .
View SolutionA person standing on a road has to hold his umbrella at 600 with the verticcal to keep the rain away. He throws the umbrella an starts running at 20ms−1. He finds that rain drops are hitting his head vertically. Find the speed of the rain drops with respect to (a) the road (b) the moving person.
View SolutionA ray of light travelling in air is inciden at angle of inciden 30∘ on one surface of slab in which refractive index varies with y. The light travels along the curve y=4x2 ( y and x are in meter) in the slab. Find out the refractive index of the slab at y=1/2m in the slab.

View SolutionFor traffic moving at 60km/h if the radius of the curve is 0.1km what is the correct angle of banking of the road Given g=10m/s2 .
View Solution
