A ray of light is refracted through a sphere whose material has a refractive index in such a way that it passes through the extremities of two radii which make an angle with each other. Prove that if is the deviation of the ray caused by its passage through the sphere, then
The correct Answer is:A
To solve the problem, we need to prove that:
cos(β−α2)=μcos(β2)
where:
- α is the angle of deviation,
- β is the angle between the two radii,
- μ is the refractive index of the sphere.
1. Understanding the Geometry:
- Consider a ray of light passing through a sphere. The ray is incident at point A and refracted at point B, making an angle β with the radius at point A and point B.
- The angles of incidence and refraction at the first surface are i and r respectively.
2. Using the Angle of Deviation:
- The angle of deviation α can be expressed as:
α=i+e−2r
where e is the angle of emergence.
3. Relating Angles:
- From the geometry of the sphere, we know that:
β+2r=180∘
This implies:
r=90∘−β2
4. Substituting for r:
- Substitute r into the deviation formula:
α=i+e−2(90∘−β2)
Simplifying this gives:
α=i+e−180∘+β
Rearranging yields:
β−α=i+e−180∘
5. Finding the Average of i and e:
- The average of the angles of incidence and emergence can be expressed as:
i+e2=90∘−β−α2
6. Using Snell's Law:
- Applying Snell's Law at the first surface:
μ1sini=μ2sinr
Given μ1=1 (air) and μ2=μ, we have:
sini=μsinr
Since r=90∘−β2, we can write:
sinr=cos(β2)
Thus:
sini=μcos(β2)
7. Expressing sini:
- We can also express sini in terms of α:
sini=sin(90∘−β−α2)=cos(β−α2)
8. Equating the Two Expressions for sini:
- We have:
cos(β−α2)=μcos(β2)
9. Final Result:
- Thus, we arrive at the desired proof:
cos(β−α2)=μcos(β2)
- α is the angle of deviation,
- β is the angle between the two radii,
- μ is the refractive index of the sphere.
- Consider a ray of light passing through a sphere. The ray is incident at point A and refracted at point B, making an angle β with the radius at point A and point B.
- The angles of incidence and refraction at the first surface are i and r respectively.
- The angle of deviation α can be expressed as:
α=i+e−2r
where e is the angle of emergence.
- From the geometry of the sphere, we know that:
β+2r=180∘
This implies:
r=90∘−β2
- Substitute r into the deviation formula:
α=i+e−2(90∘−β2)
Simplifying this gives:
α=i+e−180∘+β
Rearranging yields:
β−α=i+e−180∘
- The average of the angles of incidence and emergence can be expressed as:
i+e2=90∘−β−α2
- Applying Snell's Law at the first surface:
μ1sini=μ2sinr
Given μ1=1 (air) and μ2=μ, we have:
sini=μsinr
Since r=90∘−β2, we can write:
sinr=cos(β2)
Thus:
sini=μcos(β2)
- We can also express sini in terms of α:
sini=sin(90∘−β−α2)=cos(β−α2)
- We have:
cos(β−α2)=μcos(β2)
- Thus, we arrive at the desired proof:
cos(β−α2)=μcos(β2)
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 2 Single Correct
41 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Single Correct Option
3 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 1 Subjective
29 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
A ray of light falls on the surface of a spherical glass paper weight making an angle α with the normal and is refracted in the medium at an angle β. The angle of deviation of the emergent ray from the direction of the incident ray is :
View SolutionA ray of light falls on the surface of a spherical glass paper weight making an angle α with the normal and is refracted in the medium at an angle β. The angle of deviation of the emergent ray from the direction of the incident ray is :
View Solution
Knowledge Check
A ray of light passes through an equilateral prism (refractive index 1.5) such that angle of incidence is equal to angle of emergence and the latter is equal to 3/4th of the angle of prism. Calculate the angle of deviation.
A60∘B30∘C45∘D120∘
The refractive index of a prism is μ. Find the maximum angle of the prism for which a ray incident on it will be transmitted through other face without total internal reflection.
View SolutionA ray of light passing through a prism having refractive index √2 suffers minimum deviation. It is found that the angle of incidence is double the angle of refraction within the prism. What is the angle of prism?
View SolutionIf αandβ are two points on the hyperbola x2a2−y2b2=1 and the chord joining these two points passes through the focus (ae, 0) then ecosα−β2=
View SolutionDefine refractive index of a transparent medium. A ray of light passes through a triangular prism. Plot a graph showing the variation of angle of deviation with the angle of incidence.
View SolutionA horizontal ray of light is incident on a solid glass sphere of radius R and refractive index μ . What is net deviation of the beam when it emerged from the other side of the sphere?
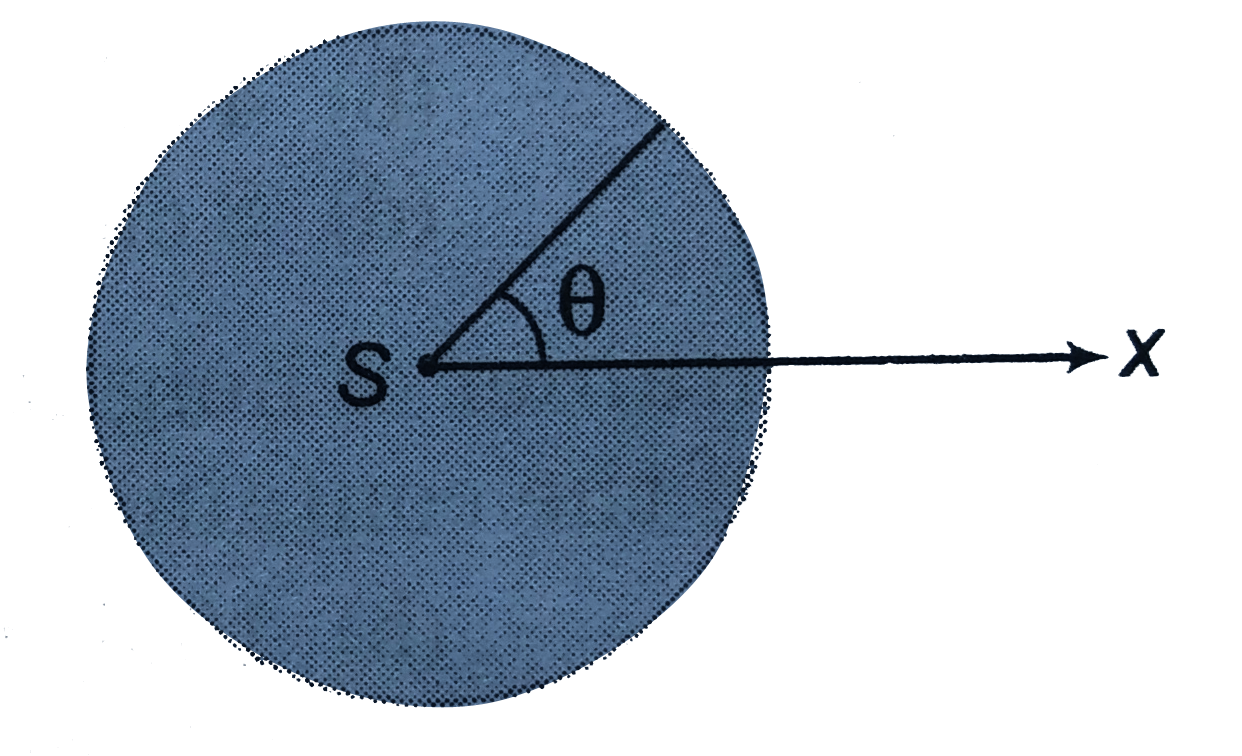
View SolutionA light source S is placed at the centre of a glass sphere of radius R and refractive index μ. The maximum angle θ with the x-axis (as shown in the figure) an incident light ray can make without suffering total internal reflection is
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
View Solution
