A cuboidal piece of wood has dimensions a, b and c. its relatively density is d. it is floating in a large body of water such that side a is vertical. It is pushed down a bit and released. The time period of SHM executed by it is
2π√abcg
2π√gda
2π√bcdg
2π√dag
The correct Answer is:D
To find the time period of the simple harmonic motion (SHM) executed by the cuboidal piece of wood when it is pushed down and released, we can follow these steps:
1. Understand the System:
- We have a cuboidal block of wood with dimensions a, b, and c.
- The relative density of the wood is d.
- The block is floating in water with side a vertical.
2. Establish the Equilibrium Condition:
- When the block is floating, the weight of the block is balanced by the buoyant force.
- The weight of the block is given by mg, where m is the mass of the block.
- The buoyant force is given by Buoyant Force=ρl⋅Vd⋅g, where ρl is the density of the liquid (water), Vd is the volume of water displaced, and g is the acceleration due to gravity.
3. Volume and Weight Relationships:
- The volume of the block V is V=a⋅b⋅c.
- The mass of the block can be expressed as m=ρs⋅V, where ρs is the density of the solid wood.
4. Displacement and Buoyant Force:
- When the block is pushed down by a distance x and released, the new displaced volume of water is Vd=b⋅c⋅x.
- The buoyant force when displaced is Buoyant Force=ρl⋅(b⋅c⋅x)⋅g.
5. Setting Up the Equation:
- At equilibrium, the weight of the block equals the buoyant force:
ρs⋅(a⋅b⋅c)⋅g=ρl⋅(b⋅c⋅h)⋅g
- This simplifies to:
ρs⋅a=ρl⋅h
- Here, h is the height of the submerged part of the block.
6. Finding the Time Period:
- The restoring force when displaced by x is proportional to the displacement x:
F=−ρl⋅(b⋅c)⋅g⋅x
- The effective spring constant k can be identified as:
k=ρl⋅(b⋅c)⋅g
- The mass m of the block is:
m=ρs⋅(a⋅b⋅c)
- The time period T of SHM is given by:
T=2π√mk
- Substituting the values:
T=2π√ρs⋅(a⋅b⋅c)ρl⋅(b⋅c)⋅g
- Simplifying gives:
T=2π√d⋅ag
- where d=ρsρl is the relative density.
Final Answer:
The time period of SHM executed by the cuboidal piece of wood is:
T=2π√d⋅ag
- We have a cuboidal block of wood with dimensions a, b, and c.
- The relative density of the wood is d.
- The block is floating in water with side a vertical.
- When the block is floating, the weight of the block is balanced by the buoyant force.
- The weight of the block is given by mg, where m is the mass of the block.
- The buoyant force is given by Buoyant Force=ρl⋅Vd⋅g, where ρl is the density of the liquid (water), Vd is the volume of water displaced, and g is the acceleration due to gravity.
- The volume of the block V is V=a⋅b⋅c.
- The mass of the block can be expressed as m=ρs⋅V, where ρs is the density of the solid wood.
- When the block is pushed down by a distance x and released, the new displaced volume of water is Vd=b⋅c⋅x.
- The buoyant force when displaced is Buoyant Force=ρl⋅(b⋅c⋅x)⋅g.
- At equilibrium, the weight of the block equals the buoyant force:
ρs⋅(a⋅b⋅c)⋅g=ρl⋅(b⋅c⋅h)⋅g
- This simplifies to:
ρs⋅a=ρl⋅h
- Here, h is the height of the submerged part of the block.
- The restoring force when displaced by x is proportional to the displacement x:
F=−ρl⋅(b⋅c)⋅g⋅x
- The effective spring constant k can be identified as:
k=ρl⋅(b⋅c)⋅g
- The mass m of the block is:
m=ρs⋅(a⋅b⋅c)
- The time period T of SHM is given by:
T=2π√mk
- Substituting the values:
T=2π√ρs⋅(a⋅b⋅c)ρl⋅(b⋅c)⋅g
- Simplifying gives:
T=2π√d⋅ag
- where d=ρsρl is the relative density.
The time period of SHM executed by the cuboidal piece of wood is:
T=2π√d⋅ag
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Advanced
34 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - More than one option is correct
50 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.4
4 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A pole is floating in a liquid with 80 cm of its length immersed. It is pushed doun a certain distance and then released. Time period of vertical oscillation is
View SolutionA weighted glass tube is floated in a liquid with 20 cm of its length immersed. It is pushed down through a certain distance and then released. Compute the time period of its vibration.
View SolutionA cylindrical block of wood of mass m and area cross-section A is floating in water (density = ρ) when its axis vertical. When pressed a little and the released the block starts oscillating. The period oscillations is
View SolutionA brass cube of side a and density σis floating in mercury of density ρ. If the cube is displaced a bit vertically, it executes S.H.M. Its time period will be
View SolutionA cylindrical object of outer diameter 20 cm and mass 2 kg floats in water with its axis vertical. If it is slightly depressed and then released, find the time period of the resulting simple harmonic motion of the object.
View SolutionOne end of a 10 cm long silk thread is fixed to a large vertical surface of a charged non-conducting plate and the other end is fastened to a small ball of mass 10 g and charge of 4×10−6C. In equilibrium, thread makes an angle of 60o with the vertical.
(a) Find the tension in the string in equilibrium.
(b) Suppose the ball is slightly pushed aside and released. Find the time period of the small oscillations.
(Use σ=7.5×10−7Cm2)
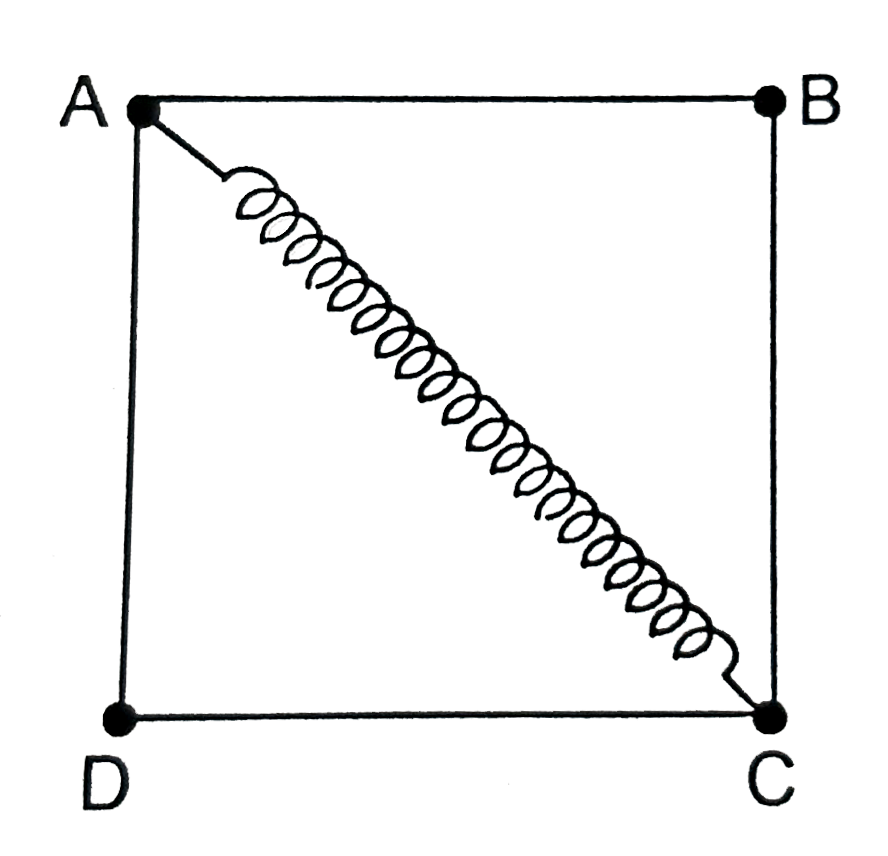
View SolutionA square hinged -structure (ABCD) is formed with four massless rods which lie on the smooth horizontal table. The hinge−D of structure is fixed with table and hinge A, hinge −B and hinge-C are free to move on the table. A point mass m is attached at hinge −B, and an ideal spring of stiffness k is connected between hinge −A and hinge-C as shown in the figure. System is in equilibrium. The mass m is slightly along line BD and released to perform SHM. The time period of SHM is π√nmk. Find n
View SolutionA cylindrical piece of cork of height 4.9 cm floats in water with its axis vertical and is made to execute slın. Calculate its time period of oscillation if its density is 0.2 gm/cc ?
View SolutionA piece of wood of uniform cross section and height 15 cm floats vertically with its height 10 cm in water and 12 cm in spirit. Find the density of (i) wood and (ii) spirit
View SolutionA wooden cube (density of wood 'd') of side 'l' flotes in a liquid of density 'ρ' with its upper and lower surfaces horizonta. If the cube is pushed slightly down and released, it performs simple harmonic motion of period 'T'. Then, 'T' is equal to :-
View Solution
