Assertion : If gravitational potential at some point is zero, then gravitational field strength at that point will also be zero.
Reason : Except at infinity gravitational potential due to a system of point masses at some finite distance can't be zero.
If both Assertin and Reason are correct and Reason is the correct explanation of Assertion
If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion
If Assertion is true but Reason is false
If Assertion is false but Reason is true
The correct Answer is:D
To solve the question, we need to analyze the assertion and the reason provided.
Step 1: Understanding the Assertion
The assertion states: "If gravitational potential at some point is zero, then gravitational field strength at that point will also be zero."
- Gravitational potential (V) is defined as the work done per unit mass in bringing a mass from infinity to that point.
- The gravitational field strength (E) is defined as the negative gradient of the gravitational potential, mathematically expressed as:
E=−dVdr
- Just because the gravitational potential is zero does not imply that the gravitational field strength is also zero. The field strength can still be non-zero if the potential changes with distance.
Conclusion for Assertion
Thus, the assertion is false.
Step 2: Understanding the Reason
The reason states: "Except at infinity, gravitational potential due to a system of point masses at some finite distance can't be zero."
- Gravitational potential due to point masses is always negative or zero at infinity.
- Since gravitational potential is a summation of contributions from all point masses, and since each contribution is negative (due to the attractive nature of gravity), the total potential cannot be zero at any finite distance.
Conclusion for Reason
Thus, the reason is true.
Final Conclusion
- The assertion is false, and the reason is true. Therefore, the correct answer is that the assertion does not follow from the reason.
---
The assertion states: "If gravitational potential at some point is zero, then gravitational field strength at that point will also be zero."
- The gravitational field strength (E) is defined as the negative gradient of the gravitational potential, mathematically expressed as:
E=−dVdr
Thus, the assertion is false.
The reason states: "Except at infinity, gravitational potential due to a system of point masses at some finite distance can't be zero."
- Since gravitational potential is a summation of contributions from all point masses, and since each contribution is negative (due to the attractive nature of gravity), the total potential cannot be zero at any finite distance.
Thus, the reason is true.
- The assertion is false, and the reason is true. Therefore, the correct answer is that the assertion does not follow from the reason.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (A) Chapter Exercises68videos
GRAVITATION
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE10videos
KINEMATICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The gravitational field intensity at a point 10,000km from the centre of the earth is 4.8Nkg−1. The gravitational potential at that point is
A−4.8×107Jkg−1B−2.4×107Jkg−1C4.8×106Jkg−1D3.6×106Jkg−1 - Question 2 - Select One
In the given circuit the potential at point B is zero, the potential at points A and D will be
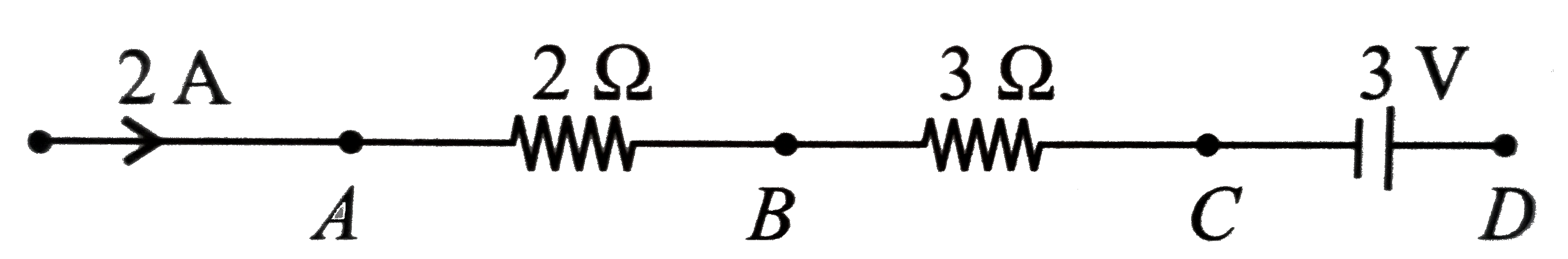 AVA=4V,VD=9VBVA=3V,VD=4VCVA=9V,VD=3VDVA=4V,VD=3V
AVA=4V,VD=9VBVA=3V,VD=4VCVA=9V,VD=3VDVA=4V,VD=3V
Find the potential V at P in the gravitational field of fixed point mass M.
View SolutionThe gravitational force on a body of mass 1.5 kg situated at a point is 45 M . The gravitational field intensity at that point is .
View SolutionAssertion : One two sides of a point mass, gravitational field strength is same at same distance,
Reason : As we move away from a point mass, value of gravitational potential decreases.View SolutionLet V and E denote the gravitational potential and gravitational field at a point. It is possile to have
View SolutionThe gravitational potential at a point outside the solid sphere of radius "R" and at a distance of "r" will be :
View SolutionIn some region, the gravitational field is zero. The gravitational potential in this region
View Solution

