The energy stored in a capacitor of capacitance 100μF is 50 J. Its potential difference is
50 V
500 v
1000 V
1500 V
The correct Answer is:C
To find the potential difference across a capacitor given its capacitance and the energy stored, we can use the formula for the energy stored in a capacitor:
U=12CV2
Where:
- U is the energy stored in the capacitor (in joules),
- C is the capacitance (in farads),
- V is the potential difference (in volts).
1. Identify the given values:
- Energy stored, U=50J
- Capacitance, C=100μF=100×10−6F=10−4F
2. Substitute the values into the energy formula:
50=12×(100×10−6)×V2
3. Multiply both sides by 2 to eliminate the fraction:
100=(100×10−6)×V2
4. Divide both sides by 100×10−6:
V2=100100×10−6=10010−4=100×104=106
5. Take the square root of both sides to solve for V:
V=√106=1000V
Final Answer:
The potential difference across the capacitor is 1000V.
---
- U is the energy stored in the capacitor (in joules),
- C is the capacitance (in farads),
- V is the potential difference (in volts).
- Energy stored, U=50J
- Capacitance, C=100μF=100×10−6F=10−4F
50=12×(100×10−6)×V2
100=(100×10−6)×V2
V2=100100×10−6=10010−4=100×104=106
V=√106=1000V
The potential difference across the capacitor is 1000V.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (A) Chapter exercises
227 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.4
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
Knowledge Check
A capacitor or capacitance C1 is charge to a potential V and then connected in parallel to an uncharged capacitor of capacitance C2. The fianl potential difference across each capacitor will be
AC1VC1+C2BC2VC1+C2C1+C2C1D1−C2C1
A 100μF capacitor is charged to a potential difference of 24V. It is connected to an uncharged capacitor of capacitance 20μF What will be the new potential difference across the 100μF capacitor?
View SolutionThe energy stored in a capacitor of capacity C and potential V is given by
View SolutionThe electrostatic energy stored in a parallel-plate capacitor of capacitance 2μF is 10mJ. If the separation between the plates of the capacitor is 1mm, the elctric field inside the capacitor is 10XN/C. The value of X is_____.
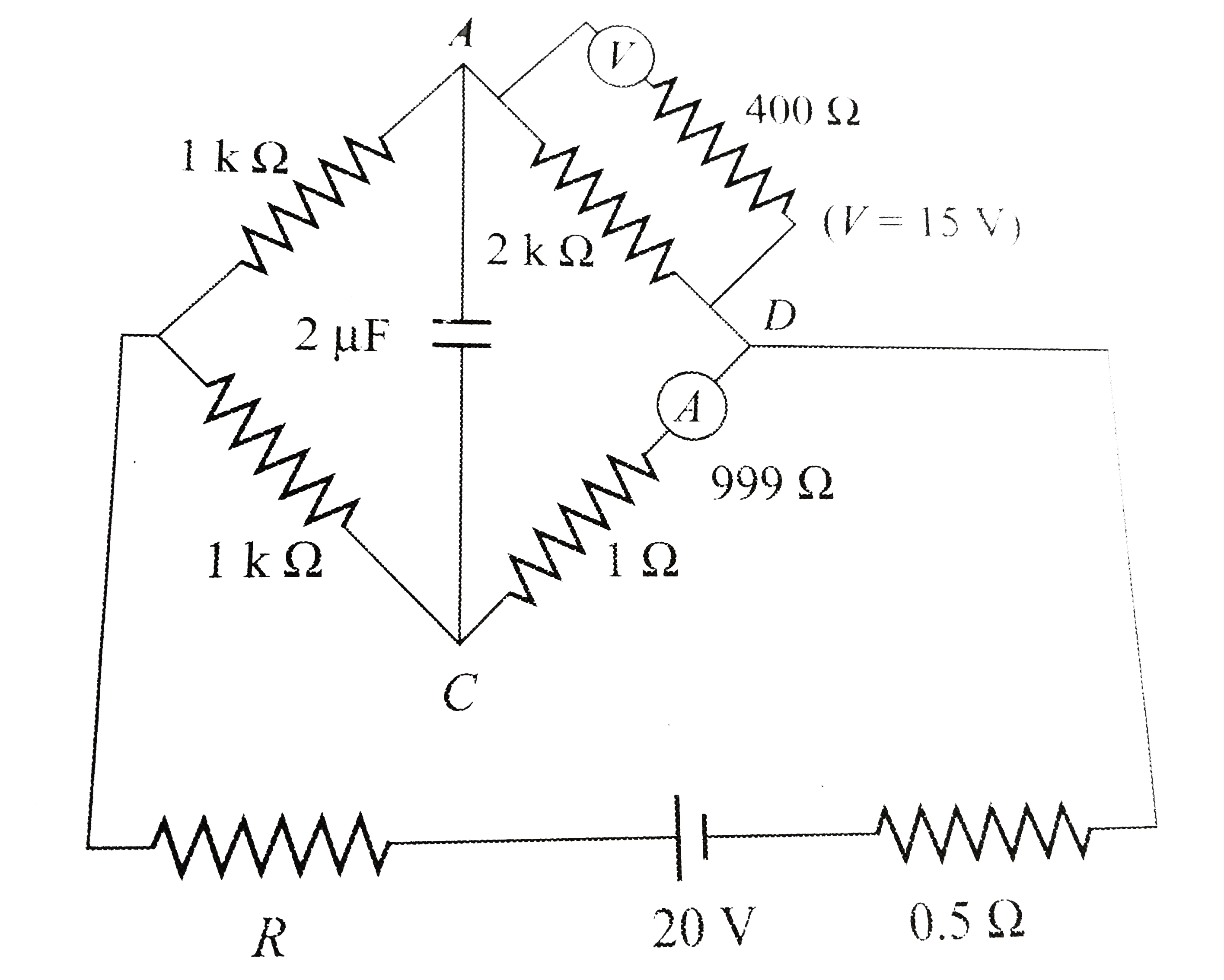
View SolutionCalculate the energy stored in the capacitor of capacitance 2μf. The voltmeter gives a reading of 15 V and the ammeter A reads 15mA .
View SolutionThe capacitor of capacitance 4μF and 6μF are connected in series. A potential difference of 500 volts applied to the outer plates of the two capacitor system. Then the charge on each capacitor is numerically
View SolutionA capacitor of capacitance C1 is charged to a potential V1 while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other .
(a) Find the total energy stored in the two capacitors before they are connected.
(b) Find the total energy stored in the parallel combination of the two capacitors.
(c ) Explain the reason for the difference of energy in parallel combination in comparison to the total energy before they are connected.
View SolutionTwo capacitor of capacitance 2μFand3μF respectively are charged to potential difference 20 V each. Now the capacitors are connected such that the positively chargd plate of one capacitory is connected to the negatively charged plate of the other. after the current in the circuit has become negligible, the potential difference across the 2μF capacitor is ____V.
View Solution

