A capacitor of capacitance value 1μF is charged to 30 V and the battery is then disconnected. If it is connected across a 2μF capacotor, then the energy lost by the system is
300μJ
450μJ
225μJ
150μF
The correct Answer is:A
To solve the problem step by step, we will calculate the initial energy stored in the capacitor, the final energy after connecting the two capacitors, and then determine the energy lost by the system.
Step 1: Calculate the Initial Energy Stored in the Capacitor
The formula for the energy stored in a capacitor is given by:
U=12CV2
Where:
- U is the energy,
- C is the capacitance,
- V is the voltage.
Given:
- C=1μF=1×10−6F
- V=30V
Substituting the values:
U=12×(1×10−6)×(30)2
U=12×(1×10−6)×900
U=900×10−62=450μJ
Step 2: Determine the Charge on the Initial Capacitor
The charge Q on the capacitor can be calculated using the formula:
Q=C×V
Substituting the values:
Q=(1×10−6)×30=30μC
Step 3: Connect the Capacitors and Find the Final Voltage
When the charged capacitor is connected to a 2μF capacitor, the total capacitance Ctotal is:
Ctotal=C1+C2=1μF+2μF=3μF
The total charge Q remains the same (conservation of charge):
Q=30μC
The final voltage Vf across the capacitors can be found using:
Vf=QCtotal=30μC3μF=10V
Step 4: Calculate the Final Energy Stored in the System
Now, we can calculate the final energy stored in the system using the new voltage and total capacitance:
Uf=12CtotalV2f
Substituting the values:
Uf=12×(3×10−6)×(10)2
Uf=12×(3×10−6)×100
Uf=300×10−62=150μJ
Step 5: Calculate the Energy Lost by the System
The energy lost ΔU can be calculated as:
ΔU=Uinitial−Ufinal
Substituting the values:
ΔU=450μJ−150μJ=300μJ
Final Answer
The energy lost by the system is 300μJ.
---
- U is the energy,
- C is the capacitance,
- V is the voltage.
- C=1μF=1×10−6F
- V=30V
U=12×(1×10−6)×900
U=900×10−62=450μJ
Uf=12×(3×10−6)×100
Uf=300×10−62=150μJ
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (A) Chapter exercises
227 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.4
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
A capacitor of capacitance 10μF is charged to a potential 50V with a battery. The battery is now disconnected and an additional charge 200μC is given to the positive plate of the capacitor. The potential difference across the capacitor will be.
View SolutionAn ideal capacitor of capacitance 0.2μF is charged to a potential difference of 10V. The charging battery is than disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5mH. The current at a time when the potential difference across the capacitor is 5V is :
View SolutionA 4μF capacitor is charged by a 200 V battery. It is then disconnected from the supply and is connected to another uncharged 2μF capacitor. During the process loss of energy (in J) s
View SolutionA capacitor of capacitance C is charged to a potential difference V0. The charged battery is disconnected and the capacitor is connected to a capacitor of unknown capacitance Cx. The potential difference across the combination is V. The value of Cx should be
View SolutionA capacitor of capacity C1=1μF is charged to a potential of 100 V. The charging battery is then removed and it is connected to another capacitor of capacity C2=2μF. One plate of C2 is earthed as shown in figure. The charges on C1andC2 in steady state will be

View SolutionA capacitor of capacitance 4μF is charged to 80V and another capacitor of capacitance 6μF is charged to 30V are connected to each other using zero resistance wires such that the positive plate of one capacitor is connected to the positive plate of the other. The energy lost by the 4μF capacitor in the process in X∗10−4J. Find the value of X.
View SolutionA capacitor of capacitance 4μF is charged to 80V and another capacitor of capacitance 6μF is charged to 30V are connected to each other using zero resistance wires such that the positive plate of one capacitor is connected to the positive plate of the other. The energy lost by the 4μF capacitor in the process in X∗10−4J. Find the value of X.
View SolutionA capacitor is charged by connecting a battery across its plates. It stores energy U. Now the battery is disconnected and another identical capacior is connected across it, then the energy stores by both capacitors of the system will be
View SolutionTwo capacitor each of capacitance C=1μF each charged to potential 100 V and 50 V respectively and connected across a uncharged capacitor 'C' = 1μF as shown in the figure if switch 'S' is closed then charge in the uncharged capacitor at equilibrium will be
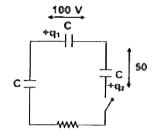
View SolutionA 4μF capacitor is charged by a 200 V supply. It is then disconnected from the supply and is connected to another uncharged 2μF capacitor. How much electrostatic energy of the first capacitor is dissipated in the form of heat and electromagnetic radiation ?
View Solution
