Question

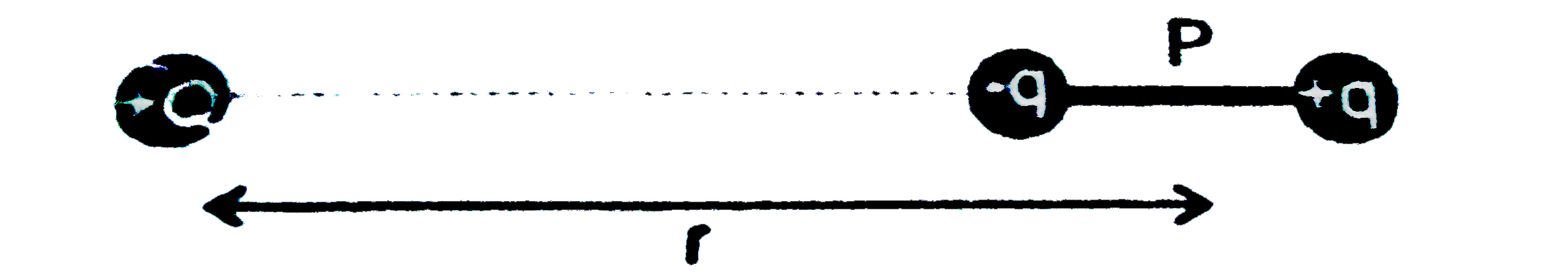
Two equal charges ${\displaystyle q}$ of opposite sign separated by a distance 2a constitute an electric dipole of dipole moment ${\displaystyle p}$. If P is a point at a distance r from the centre of the dipole and the line joining the centre of the dipole to this point makes an angle ${\displaystyle \theta }$ with the axis of the dipole, then then potential at P is given by ${\displaystyle (r>>2a)}$ where (p = 2 qa)

Options

A. `V=(p cos theta)/(4 piepsilon_(0)r^(2))`
B. `V=(p cos theta)/(4 piepsilon_(0)r)`
C. `V=(p sin theta)/(2 piepsilon_(0)r)`
D. `V=(p cos theta)/(2 piepsilon_(0)r^(2))`

Answer

B

Explanation

To solve the problem, we need to find the electric potential $$V$$ at a point $$P$$ due to an electric dipole consisting of two equal and opposite charges $$+q$$ and $$-q$$ separated by a distance $$2a$$. The dipole moment $$p$$ is given by $$p=2qa$$.

1. Understanding the Dipole Configuration:
- We have two charges: \( +q \) and \( -q \) separated by a distance \( 2a \).
- The dipole moment \( p \) is defined as \( p = q \cdot d \), where \( d \) is the separation between the charges. Here, \( d = 2a \), so \( p = 2qa \).

2. Positioning the Dipole:
- Place the dipole along the x-axis with the positive charge at \( (-a, 0) \) and the negative charge at \( (a, 0) \).
- The center of the dipole is at the origin \( (0, 0) \).

3. Identifying the Point \( P \):
- The point \( P \) is at a distance \( r \) from the center of the dipole.
- The line joining the center of the dipole to point \( P \) makes an angle \( \theta \) with the dipole axis (x-axis).

4. Calculating the Electric Potential:
- The electric potential \( V \) due to a dipole at a point in space is given by the formula:
\( V = \frac{1}{4 \pi \epsilon_0} \cdot \frac{p \cos \theta}{r^2} \)
- Here, \( \epsilon_0 \) is the permittivity of free space.

5. Substituting the Dipole Moment:
- Substitute \( p = 2qa \) into the potential formula:
\( V = \frac{1}{4 \pi \epsilon_0} \cdot \frac{2qa \cos \theta}{r^2} \)

6. Final Expression:
- Thus, the electric potential \( V \) at point \( P \) is:
\( V = \frac{2qa \cos \theta}{4 \pi \epsilon_0 r^2} \)

Conclusion:
The potential at point \( P \) due to the dipole is given by:
\( V = \frac{p \cos \theta}{4 \pi \epsilon_0 r^2} \)
where \( p = 2qa \).