Two wires of the same material but of different diameters carry the same current i. If the ratio of their diameters is 2:1 , then the corresponding ratio of their mean drift velocities will be
4:1
1:1
1:2
1:4
The correct Answer is:D
To solve the problem, we need to find the ratio of the mean drift velocities of two wires made of the same material but having different diameters, given that they carry the same current.
1. Understand the relationship between current and drift velocity:
The current I in a wire is given by the formula:
I=n⋅e⋅A⋅vd
where:
- n = number of free electrons per unit volume (constant for the same material),
- e = charge of an electron (constant),
- A = cross-sectional area of the wire,
- vd = mean drift velocity of the charge carriers.
2. Identify the given information:
- The two wires carry the same current I.
- The ratio of their diameters is d1:d2=2:1.
3. Relate the cross-sectional area to diameter:
The cross-sectional area A of a wire is related to its diameter d by the formula:
A=πd24
Therefore, if we denote the diameters of the two wires as d1 and d2, their areas will be:
A1=πd214,A2=πd224
4. Express the drift velocity in terms of area:
Since the current I is the same for both wires, we can set up the following relationships:
I=n⋅e⋅A1⋅vd1=n⋅e⋅A2⋅vd2
From this, we can derive that:
vd1=In⋅e⋅A1,vd2=In⋅e⋅A2
5. Find the ratio of drift velocities:
Taking the ratio of the drift velocities:
vd1vd2=A2A1
6. Substituting the areas:
Using the areas derived from the diameters:
A1A2=πd214πd224=d21d22
Thus, we have:
vd1vd2=d22d21
7. Substituting the diameter ratio:
Given d1:d2=2:1, we can express this as:
d1=2d,d2=d
Therefore:
vd1vd2=(d)2(2d)2=d24d2=14
8. Final ratio of drift velocities:
This means:
vd1:vd2=1:4
Conclusion:
The ratio of the mean drift velocities of the two wires is 1:4.
The current I in a wire is given by the formula:
I=n⋅e⋅A⋅vd
where:
- n = number of free electrons per unit volume (constant for the same material),
- e = charge of an electron (constant),
- A = cross-sectional area of the wire,
- vd = mean drift velocity of the charge carriers.
- The two wires carry the same current I.
- The ratio of their diameters is d1:d2=2:1.
The cross-sectional area A of a wire is related to its diameter d by the formula:
A=πd24
Therefore, if we denote the diameters of the two wires as d1 and d2, their areas will be:
A1=πd214,A2=πd224
Since the current I is the same for both wires, we can set up the following relationships:
I=n⋅e⋅A1⋅vd1=n⋅e⋅A2⋅vd2
From this, we can derive that:
vd1=In⋅e⋅A1,vd2=In⋅e⋅A2
Taking the ratio of the drift velocities:
vd1vd2=A2A1
Using the areas derived from the diameters:
A1A2=πd214πd224=d21d22
Thus, we have:
vd1vd2=d22d21
Given d1:d2=2:1, we can express this as:
d1=2d,d2=d
Therefore:
vd1vd2=(d)2(2d)2=d24d2=14
This means:
vd1:vd2=1:4
The ratio of the mean drift velocities of the two wires is 1:4.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - B. Assertion and reason
18 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Match the columns
4 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Check point
70 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
Two wires of the same material and same mass are stretched by the same force. Their lengths are in the ratio 2:3. Their elongation are in the ratio
View SolutionTwo wires of the same material and length are stretched by the same force. Their masses are in the ratio 3:2. Their elongations are in the ratio
View SolutionTwo wires of the same length are shaped into a square and a circle. If they carry same current, ratio of the magnetic moment is
View SolutionIf the diameter of two different nuclei are in the ratio 2: 1, then calculate the ratio of their mass number
View SolutionTwo wire mode of the same material are subjected to force in the ratio of 1:2. Their lengths are in the ratio 8:1 and diameters in the raio 2:1. Find the ratio of their extension.
View SolutionTwo wires of same length are shaped into a square and a circle. If they carry same current, ratio of the magnetic moment is
View SolutionIn Fig. the circular and the straight parts of the wire are made of same material but have different diameters. The magnetic field at the centre is zero.
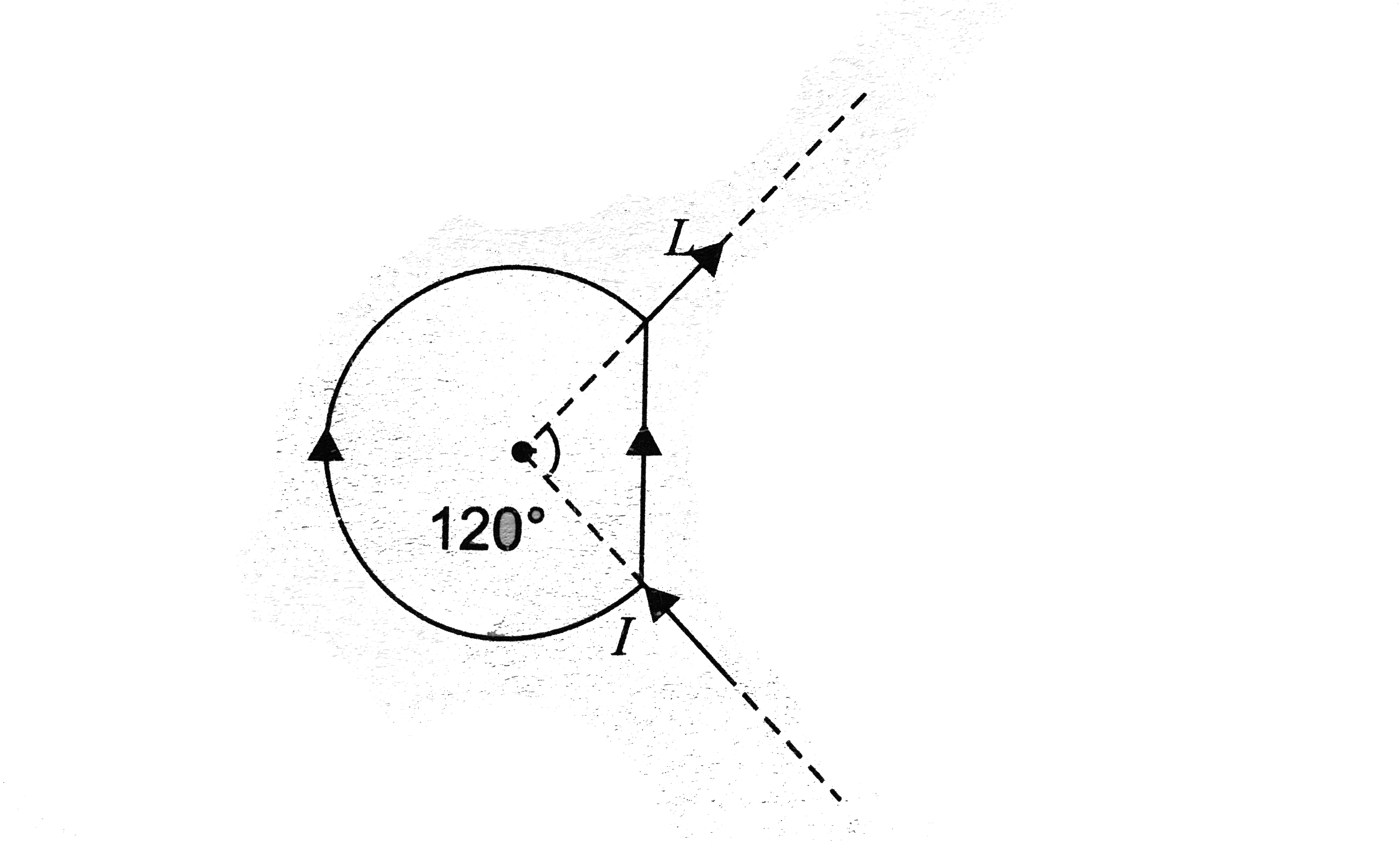
Ratio of the currents I1andI2 flowing through the circular and straight parts is
View SolutionIn Fig. the circular and the straight parts of the wire are made of same material but have different diameters. The magnetic field at the centre is zero.
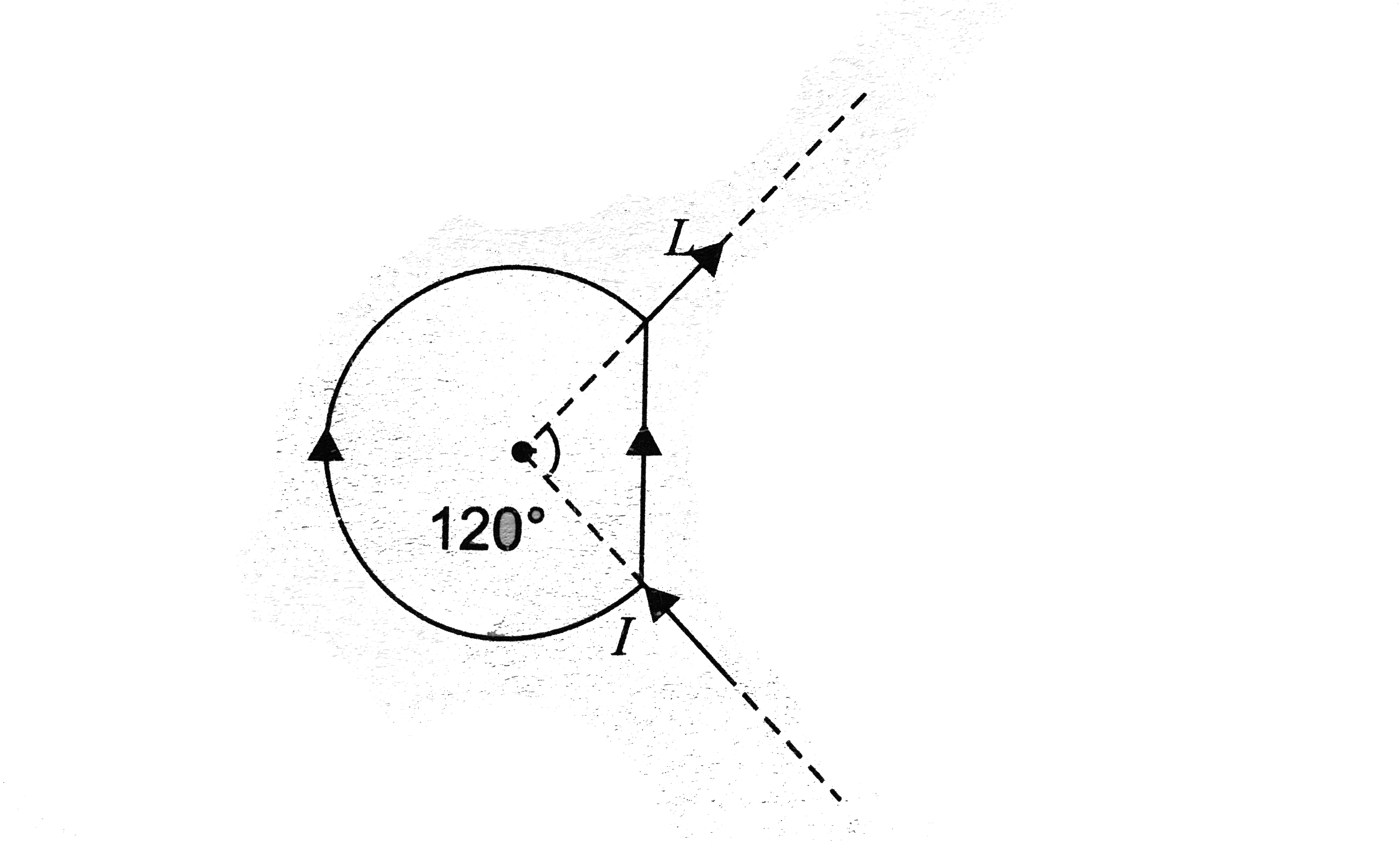
The ratio of the diameters of wires of circular and straight parts is
View SolutionIn Fig. the circular and the straight parts of the wire are made of same material but have different diameters. The magnetic field at the centre is zero.

The ratio of the diameters of wires of circular and straight parts is
View SolutionAn electric current is passed through a circuit containing two wires of the same material connected in parallel. If the lengths and radii of the wire are in the ratio 43 and 23, then the ratio of the currents passing through the wires will be
View Solution
