A metal ring is'melted and a solid sphere is made out of it. What happens to the M.I. about a vertical axis through the centre ?
To solve the problem of what happens to the moment of inertia (M.I.) about a vertical axis through the center when a metal ring is melted and formed into a solid sphere, we can follow these steps:
1. Understand the Moment of Inertia:
The moment of inertia (I) is a measure of an object's resistance to changes in its rotation. It depends on the mass distribution relative to the axis of rotation.
2. Moment of Inertia of a Metal Ring:
The moment of inertia of a thin ring about an axis perpendicular to its plane and through its center is given by the formula:
Iring=mr2
where m is the mass of the ring and r is the radius of the ring.
3. Moment of Inertia of a Solid Sphere:
The moment of inertia of a solid sphere about an axis through its center is given by:
Isphere=25mR2
where R is the radius of the sphere.
4. Mass Conservation:
When the metal ring is melted and formed into a solid sphere, the mass remains constant. Therefore, the mass of the ring m is equal to the mass of the sphere m.
5. Comparison of Radii:
When the ring is melted, the mass is redistributed. The radius of the sphere R will be smaller than the radius r of the ring, assuming the volume remains constant. The volume of the ring can be expressed as V=2πr2t (where t is the thickness), and the volume of the sphere is V=43πR3.
6. Effect on Moment of Inertia:
Since the mass is now closer to the axis of rotation (the center), the moment of inertia decreases. This is because the moment of inertia is proportional to the square of the distance from the axis of rotation.
7. Conclusion:
Therefore, when the metal ring is melted and formed into a solid sphere, the moment of inertia about a vertical axis through the center decreases.
Final Answer:
The moment of inertia about a vertical axis through the center decreases when a metal ring is melted and a solid sphere is made out of it.
The moment of inertia (I) is a measure of an object's resistance to changes in its rotation. It depends on the mass distribution relative to the axis of rotation.
The moment of inertia of a thin ring about an axis perpendicular to its plane and through its center is given by the formula:
Iring=mr2
where m is the mass of the ring and r is the radius of the ring.
The moment of inertia of a solid sphere about an axis through its center is given by:
Isphere=25mR2
where R is the radius of the sphere.
When the metal ring is melted and formed into a solid sphere, the mass remains constant. Therefore, the mass of the ring m is equal to the mass of the sphere m.
When the ring is melted, the mass is redistributed. The radius of the sphere R will be smaller than the radius r of the ring, assuming the volume remains constant. The volume of the ring can be expressed as V=2πr2t (where t is the thickness), and the volume of the sphere is V=43πR3.
Since the mass is now closer to the axis of rotation (the center), the moment of inertia decreases. This is because the moment of inertia is proportional to the square of the distance from the axis of rotation.
Therefore, when the metal ring is melted and formed into a solid sphere, the moment of inertia about a vertical axis through the center decreases.
The moment of inertia about a vertical axis through the center decreases when a metal ring is melted and a solid sphere is made out of it.
Topper's Solved these Questions
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (LONG ANSWER QUESTIONS)
14 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (SHORT ANSWER QUESTIONS)
35 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 1 (FROM BANKING OR RAILS AND ROADS)
13 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
50 Videos
Similar Questions
A ring of radius r made of wire of density ρ is rotated about a stationary vertical axis passing through its centre and perpendicular to the plane of the ring as shown in the figure. Determine the angular velocity (in rad/s) of ring at which the ring breaks. The wire breaks at tensile stress σ. Ignore gravity. Take σ/ρ=4 and r=1m.
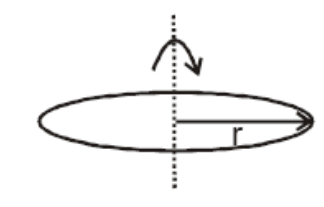
View SolutionA ring of radius r made of wire of density ρ is rotated about a stationary vertical axis passing through its centre and perpendicular to the plane of the ring as shown in the figure. Determine the angular velocity (in rad/s) of ring at which the ring breaks. The wire breaks at tensile stress σ. Ignore gravity. Take σ/ρ=4 and r=1m.

View SolutionThe moment of inertia of a flat angular ring is having mass M, inner radius a and outer radius b about the perpendicular axis through the centre is
View SolutionA circular disc of radius R and thickness R6 has moment of inertia about an axis passing through its centre and perpendicular to its plane. It is melted and recasted into a solid sphere. The moment of inertia of the sphere about its diameter as axis of rotation is
View SolutionA disc of radius r is removed from the centre of a disc of mass M and radius R. The M.I. About the axis through the centre and normal to the plane will be:
View SolutionConsider three bodies : a ring, a disc and a sphere. All the bodies have same mass and radius. All rotate about their axis through their respective centre of mass and perpendicular to the plane. What is the ratio of moment of inertia ?
View SolutionThe moment of inertia of a flat annular ring having mass M, inner radius a and outer radius b about the diametric axis through the centre is
View SolutionA ring of radius R having a linear charge density λ moves towards a solid imaginary sphere of radius R2, so that the centre of ring passes through the centre of sphere. The axis of the ring is perpendicular to the line joining the centres of the ring and the sphere. The maximum flux through the sphere in this process is
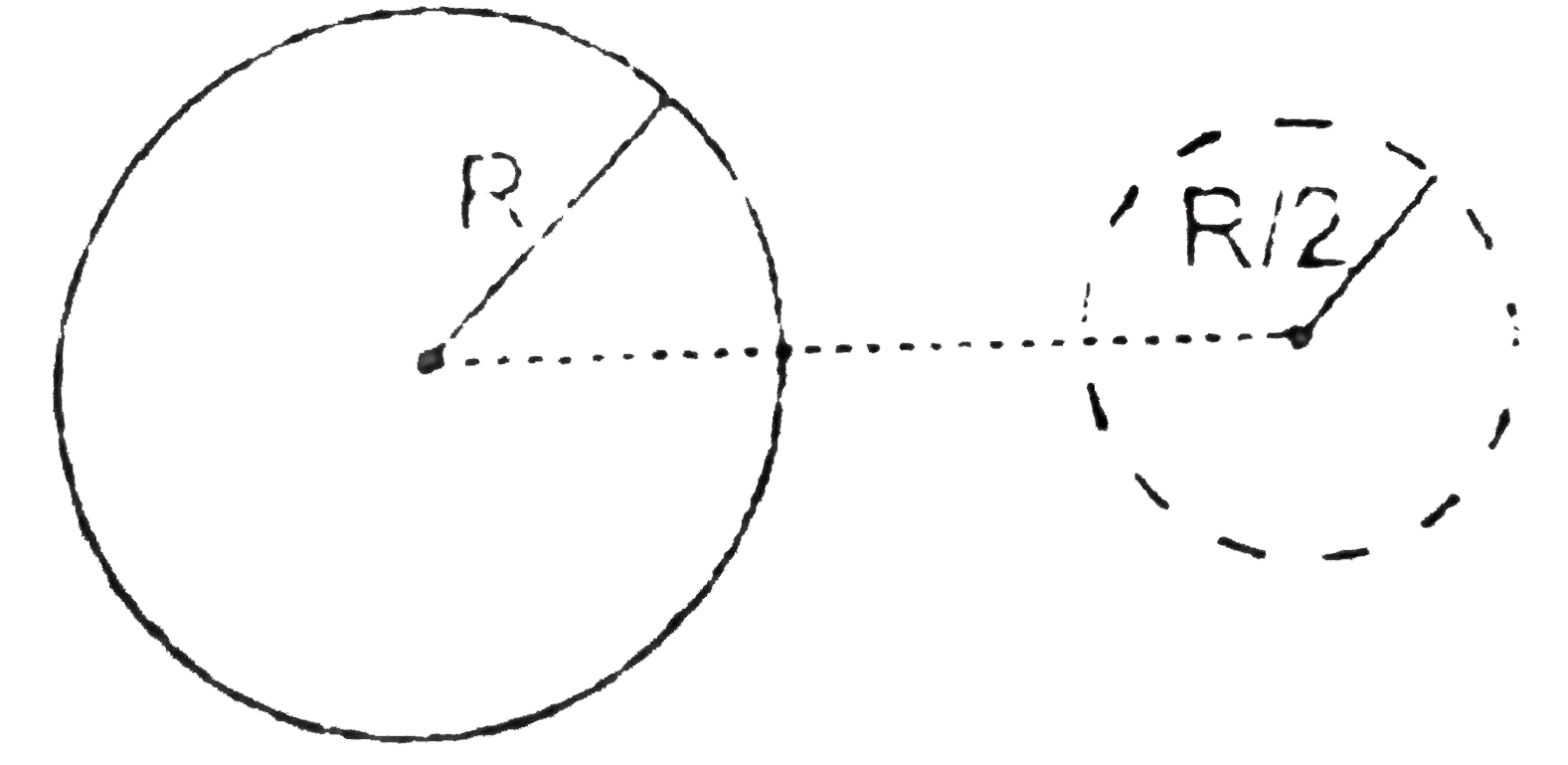
View SolutionA body has mass M and radius 2r. If the body assumes four shapes, solid cylinder, solid sphere, disc and ring of same radius, then the moment of inertia will be maximum about axis through centre of mass for:
View SolutionFrom a complete ring of mass M and radius R, a 30∘sector is removed. The moment of inertia of the incomplete ring about an axis passing through the centre of the ring and perpendicular to the plane of the ring is
 ,
,
View Solution
