A line segment AB is bisected at point P and through point P another line segment PQ, which is perpendicular to AB, is drawn. Show that : QA = QB.
To show that QA = QB given that a line segment AB is bisected at point P and through point P another line segment PQ, which is perpendicular to AB, is drawn, we can follow these steps:
1. Draw the Line Segment AB:
- Begin by drawing a line segment AB.
2. Locate the Midpoint P:
- Identify the midpoint P of the line segment AB. Since P bisects AB, we have AP = PB.
3. Draw the Perpendicular Line PQ:
- From point P, draw a line segment PQ such that it is perpendicular to AB. This means that angle QPA and angle QPB are both 90 degrees.
4. Identify the Triangles:
- Now, consider the two triangles: Triangle QAP and Triangle QBP.
5. Establish the Angles:
- Since PQ is perpendicular to AB, we have:
- ∠QPA = 90°
- ∠QPB = 90°
6. Establish the Equal Sides:
- We know that AP = PB because P is the midpoint of AB.
7. Identify the Common Side:
- The side PQ is common to both triangles QAP and QBP.
8. Apply the SAS Congruence Rule:
- We have:
- Angle QAP = Angle QBP (both are 90°)
- AP = PB (since P bisects AB)
- PQ is common to both triangles.
- Therefore, by the Side-Angle-Side (SAS) congruence criterion, Triangle QAP is congruent to Triangle QBP.
9. Conclude that QA = QB:
- Since the two triangles are congruent, the corresponding sides are equal. Thus, QA = QB.
Final Statement:
Therefore, we have shown that QA = QB.
---
- Begin by drawing a line segment AB.
- Identify the midpoint P of the line segment AB. Since P bisects AB, we have AP = PB.
- From point P, draw a line segment PQ such that it is perpendicular to AB. This means that angle QPA and angle QPB are both 90 degrees.
- Now, consider the two triangles: Triangle QAP and Triangle QBP.
- Since PQ is perpendicular to AB, we have:
- ∠QPA = 90°
- ∠QPB = 90°
- We know that AP = PB because P is the midpoint of AB.
- The side PQ is common to both triangles QAP and QBP.
- We have:
- Angle QAP = Angle QBP (both are 90°)
- AP = PB (since P bisects AB)
- PQ is common to both triangles.
- Therefore, by the Side-Angle-Side (SAS) congruence criterion, Triangle QAP is congruent to Triangle QBP.
- Since the two triangles are congruent, the corresponding sides are equal. Thus, QA = QB.
Therefore, we have shown that QA = QB.
Topper's Solved these Questions
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - EXERCISE 9(B)
20 Videos
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - QUESTIONS
8 Videos
- STATISTICS AND GRAPH WORK BOOK - ICSECHAPTER - STATISTICS AND GRAPH WORK EXERCISE - TOPIC - 2 (4 Marks Questions)
14 Videos
- TRIGONOMETRICAL RATIOS BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - EXERCISE 22(B)
50 Videos
Similar Questions
Draw a line segment AB = 6 cm. Take a point P above this line segment. Draw a perpendicular to AB through the point P.
View SolutionDraw a line segment AB=6.2 cm. mark a point P in AB such that BP=4 cm. through point P draw a perpendicular to AB.
View SolutionIn each of the following, draw a perpendicular through point P to the line segment AB:

 .
.
View SolutionAB is a given line segment with a point C on it . Draw a line segment perpendicular to AB at the point C.
View SolutionDraw a line segment AB=5.5 cm. mark a point P, such that PA=6cm and PB=4.8 cm. from the point P, draw a perpendicular to AB.
View SolutionDraw a perpendicular to a given line segment though a point K on the line segment .
View SolutionDraw a perpendicular to a given line segment though a point R on the line segment .
View SolutionDraw a line segment AB of lineth 10cm. Mark a point P on AB such that AP=4cm. Draw a line through P perpendicular to AB.
View SolutionDraw a line segment AB. Find a point P, 4 cm above the line segment, and draw a line segment parallel to AB through P.
View Solution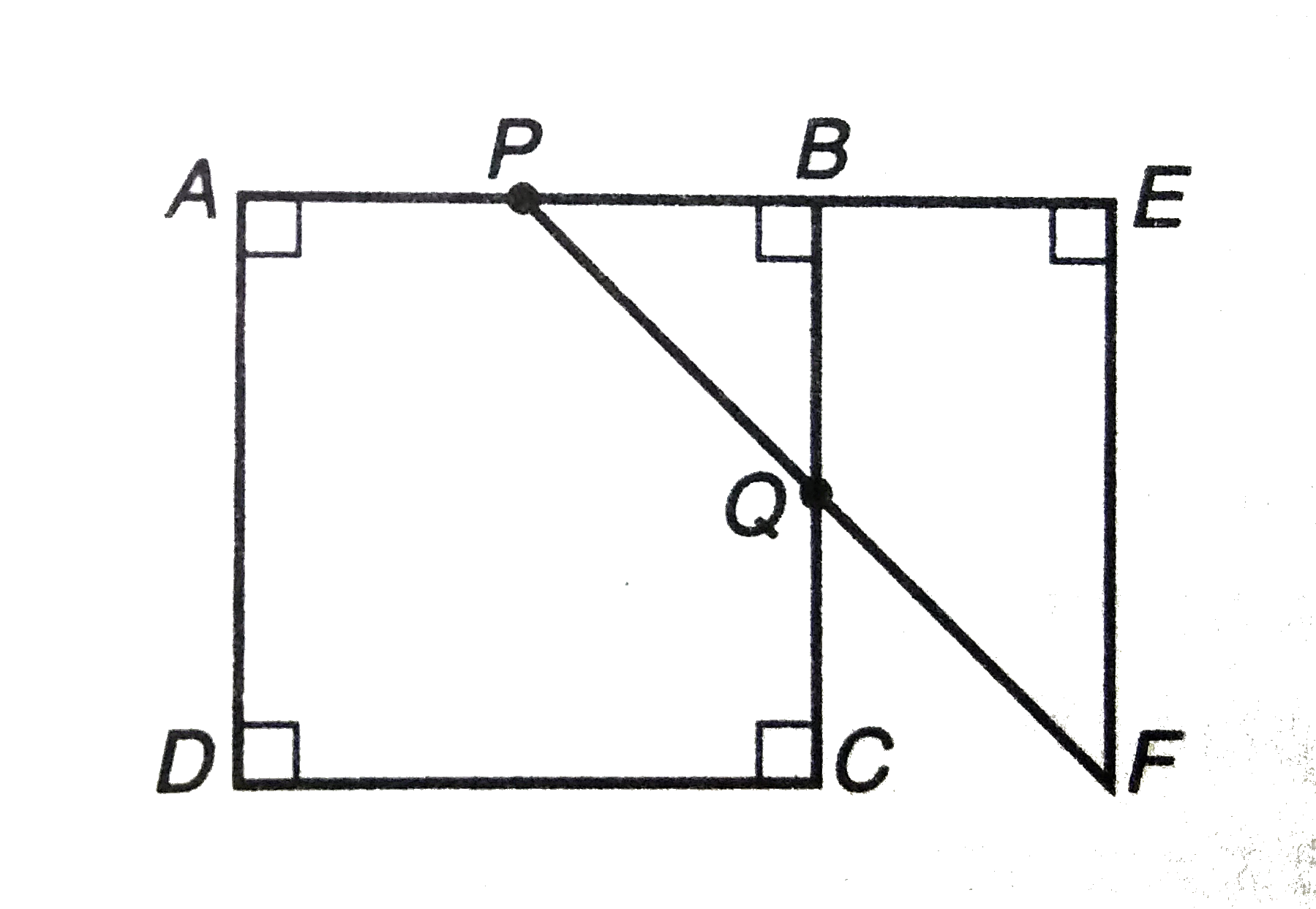
In the figure above, P and Q are the midpoints of sides AB and BC, respectively, of square ABCD. Line segment PB is extended by its own length to point E, and line segment PQ is extended to point F so that FE⊥PE. If the area of square ABCD is 9, what is the area of quadrilateral QBEF?
View Solution
