In the following figure, P and Q are the points of intersection of two circles with centres O and O'. If straight lines APB and CQD are parallel to OO', prove that

AB=CD
Step by step video & image solution for In the following figure, P and Q are the points of intersection of two circles with centres O and O'. If straight lines APB and CQD are parallel to OO', prove that AB=CD by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLEBOOK - ICSECHAPTER - CIRCLEEXERCISE - EXERCISE 17(C)
29 Videos
- CIRCLEBOOK - ICSECHAPTER - CIRCLEEXERCISE - EXERCISE 17(D)
12 Videos
- CIRCLEBOOK - ICSECHAPTER - CIRCLEEXERCISE - EXERCISE 17(A)
14 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - DISTANCE FORMULA
11 Videos
- CIRCLES BOOK - ICSECHAPTER - CIRCLES EXERCISE - TOPIC-2
25 Videos
Similar Questions
In the following figure, P and Q are the points of intersection of two circles with centres O and O'. If straight lines APB and CQD are parallel to OO', prove that
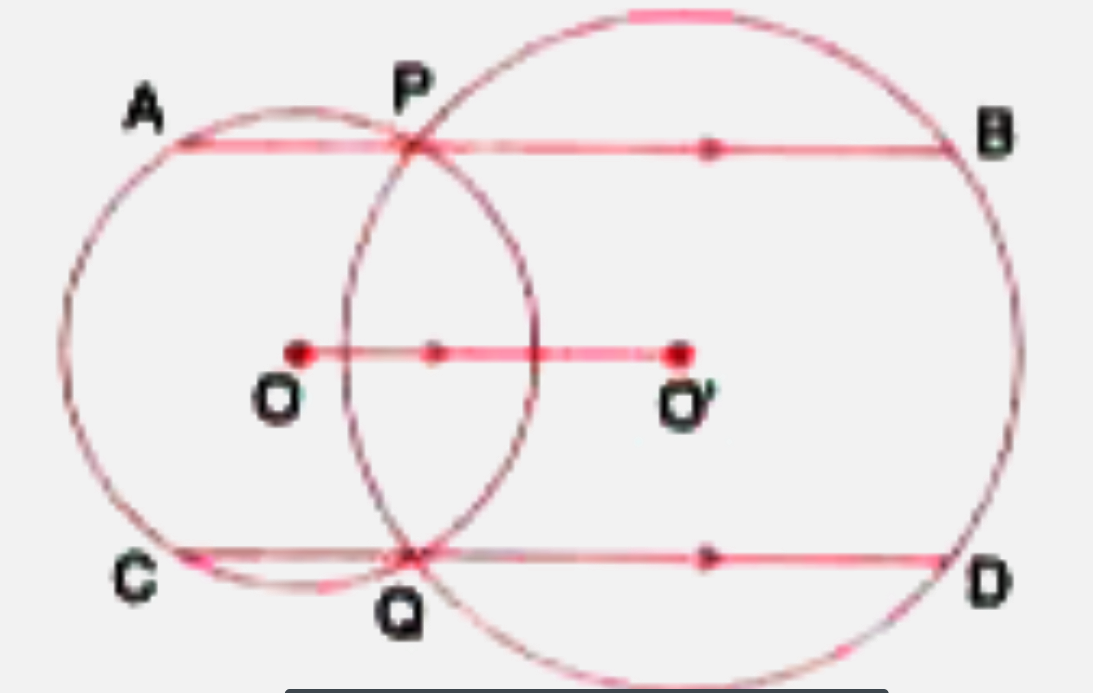
OO'=12AB=12CD
View SolutionIn Figure, O and O' are centres of two circles intersecting at B and C.ACD is a straight line, find x.
View SolutionIn Figure, P and Q are centres of two circles intersecting at B and C.ACD is a straight line. Then, ∠BQD=
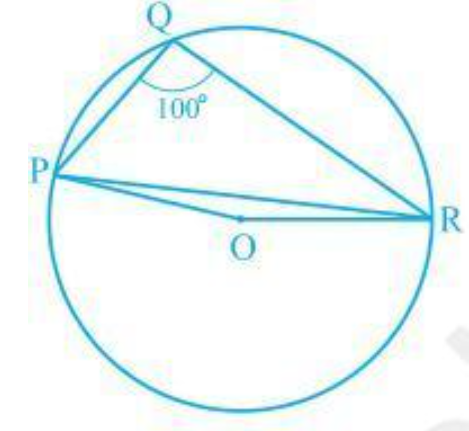
View Solution∠PQR=100∘, where P, Q and R are points on a circle with centre O. Find ∠OPR
View SolutionIn Figure, find m ∠PQB where O is the centre of the circle
View SolutionIf TP and TQ are the two tangents to a circle with centre O so that ∠POQ=110∘, then ∠PTQ is equal to
View SolutionAB is a diameter of the circle with centre O and chord CD is equal to radius OC (fig). AC and BD proudced meet at P. Prove that ∠CPD=60∘.

View SolutionIn Figure, ∠PQR=1000, where P, Q and R are points on a circle with centre. O. Find ∠OPR
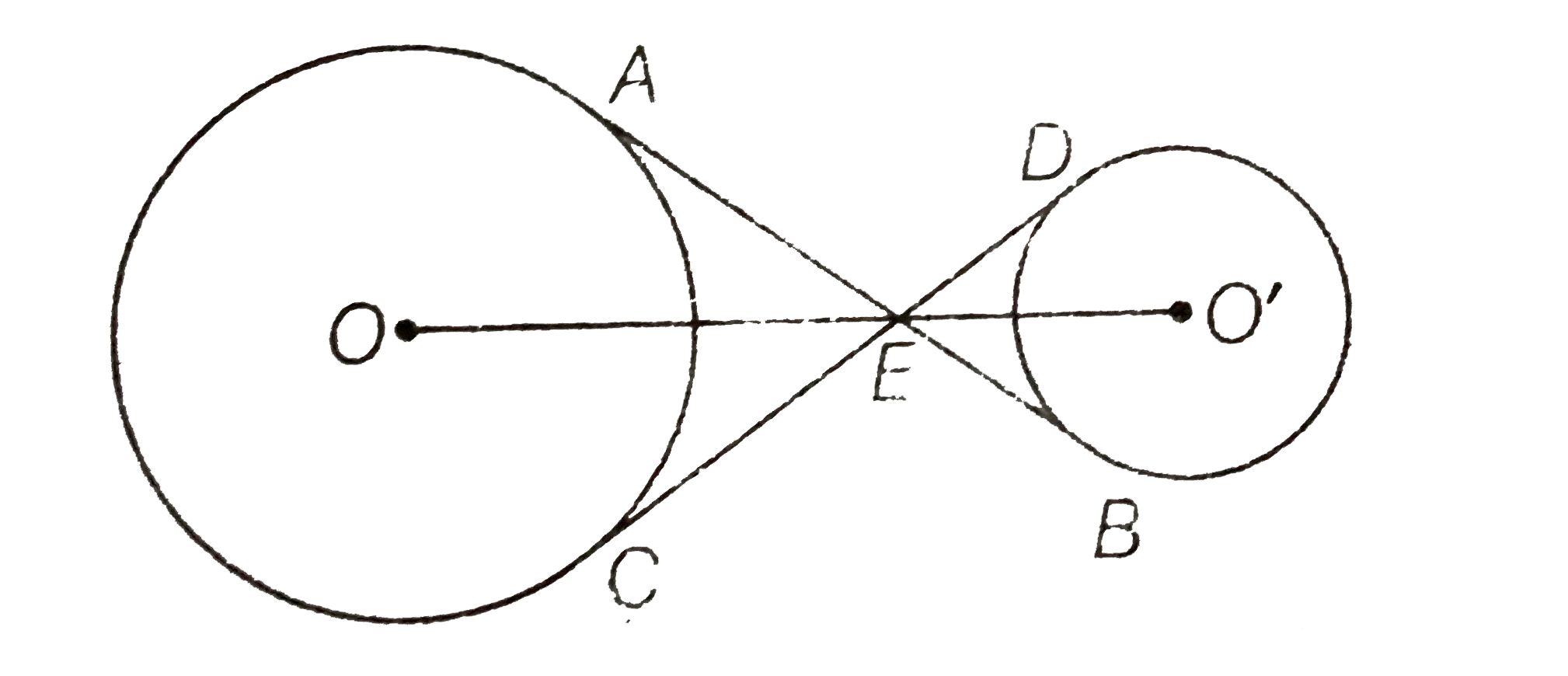
View SolutionIn a figure the common tangents, AB and CD to two circles with centers O and O' intersect at E. Prove that the points O, E and O' are collinear.
View SolutionM and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. Prove that :
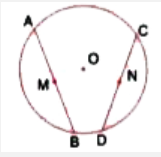
∠AMN=∠CNM.
View Solution
