Equation of the circumcircle of a triangle formed by the lines L1=0,L2=0andL3=0 can be written as L1L2+λL2L3+μL3L1=0, where λandμ are such that coefficient of x2 =coefficient of y2 and coefficient of xy=0.
L1L22+λL2L23+μL21=0 represents
λL1L4+μL2L3=0
λL1L3+μL2L4=0
λL1L2+μL3L4=0
λL21L3+μL22L4=0
The correct Answer is:C
To solve the problem, we need to analyze the given equations and derive the required representation step by step.
Step 1: Understand the Given Lines
The lines L1=0, L2=0, and L3=0 represent three lines in the plane. The intersection of these lines forms a triangle, and the circumcircle of this triangle can be expressed in terms of these lines.
Hint: Identify what the lines represent geometrically and how they interact.
Step 2: Write the Equation of the Circumcircle
The circumcircle of the triangle formed by these lines can be expressed as:
L1L2+λL2L3+μL3L1=0
where λ and μ are coefficients that need to satisfy certain conditions.
Hint: Recognize that the circumcircle equation is a quadratic in terms of x and y.
Step 3: Coefficient Conditions
The problem states that the coefficient of x2 must equal the coefficient of y2, and the coefficient of xy must be zero. This gives us two conditions to satisfy:
1. Coefficient of x2= Coefficient of y2
2. Coefficient of xy=0
Hint: Set up equations based on the coefficients of the terms in the circumcircle equation.
Step 4: Analyze the Given Expression
The expression L1L22+λL2L23+μL21=0 needs to be analyzed to determine the type of curve it represents.
Hint: Substitute the lines into the expression and simplify.
Step 5: Substitute Points
Let’s take a point (h,k) that satisfies L1(h,k)=0, L2(h,k)=0, and L3(h,k)=0. Substitute this point into the equation:
L1(h,k)L2(h,k)2+λL2(h,k)L3(h,k)2+μL1(h,k)2=0
Since each Li(h,k)=0, the entire expression equals zero.
Hint: Understand that substituting the point will help in confirming the nature of the curve.
Step 6: Conclusion
The expression L1L22+λL2L23+μL21=0 represents a curve that passes through the points of intersection of the lines L1=0, L2=0, and L3=0. This indicates that the curve is defined by the relationships between these lines.
Final Answer: The expression represents a curve passing through the point of intersection of the lines L1=0, L2=0, and L3=0.
The lines L1=0, L2=0, and L3=0 represent three lines in the plane. The intersection of these lines forms a triangle, and the circumcircle of this triangle can be expressed in terms of these lines.
The circumcircle of the triangle formed by these lines can be expressed as:
L1L2+λL2L3+μL3L1=0
where λ and μ are coefficients that need to satisfy certain conditions.
The problem states that the coefficient of x2 must equal the coefficient of y2, and the coefficient of xy must be zero. This gives us two conditions to satisfy:
1. Coefficient of x2= Coefficient of y2
2. Coefficient of xy=0
The expression L1L22+λL2L23+μL21=0 needs to be analyzed to determine the type of curve it represents.
Let’s take a point (h,k) that satisfies L1(h,k)=0, L2(h,k)=0, and L3(h,k)=0. Substitute this point into the equation:
L1(h,k)L2(h,k)2+λL2(h,k)L3(h,k)2+μL1(h,k)2=0
Since each Li(h,k)=0, the entire expression equals zero.
The expression L1L22+λL2L23+μL21=0 represents a curve that passes through the points of intersection of the lines L1=0, L2=0, and L3=0. This indicates that the curve is defined by the relationships between these lines.
Topper's Solved these Questions
- CIRCLE Book:ARIHANT MATHS ENGLISHChapter:CIRCLE Exercise:Exercise (Single Integer Answer Type Questions)
Explore 9 Videos
- CIRCLE Book:ARIHANT MATHS ENGLISHChapter:CIRCLE Exercise:Exercise (Statement I And Ii Type Questions)
Explore 7 Videos
- CIRCLE Book:ARIHANT MATHS ENGLISHChapter:CIRCLE Exercise:Exercise (More Than One Correct Option Type Questions)
Explore 15 Videos
- BIONOMIAL THEOREMBook:ARIHANT MATHS ENGLISHChapter:BIONOMIAL THEOREMExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 21 Videos
- COMPLEX NUMBERSBook:ARIHANT MATHS ENGLISHChapter:COMPLEX NUMBERSExercise:Complex Number Exercise 8
Explore 2 Videos
Similar Questions
Equation of the circumcircle of a triangle formed by the lines L1=0,L2=0andL3=0 can be written as L1L2+λL2L3+μL3L1=0, where λandμ are such that coefficient of x2 =coefficient of y2 and coefficient of xy=0.
If L1L2+λL2L3+μL3L1=0 is such that μ=0andλ is non-zero, then it represents
View SolutionConsider the lines given by
L1:x+3y−5=0
L2:3x−ky−1=0
L3:5x+2y−12=0
Match the following lists.

View SolutionThree sided of a triangle have equations L1≡y−mix=o;i=1,2and 3. Then L1L2+λL2L3+μL3L1=0 where λ≠0,μ≠0, is the equation of the circumcircle of the triangle if (a)1+λ+μ=m1m2+λm2m3+λm3m1 (b)m1(1+μ)+m2(1+λ)+m3(μ+λ)=0 (c)1m3+1m1+1m1=1+λ+μ (d)none of these
View SolutionLet the lines l1 and l2 be normals to y2=4x and tangents to x2=−12y (where l1andl2 are not x - axis). The absolute value of the difference of slopes of l1andl2 is
View SolutionYou are given three lenses L1,L2andL3 each of focal length 20 cm. An object is kept at 40 cm in front of L1, as shown. The final real image is formed at the focus 'I' of L3. Find the separations between L1,L2andL3.
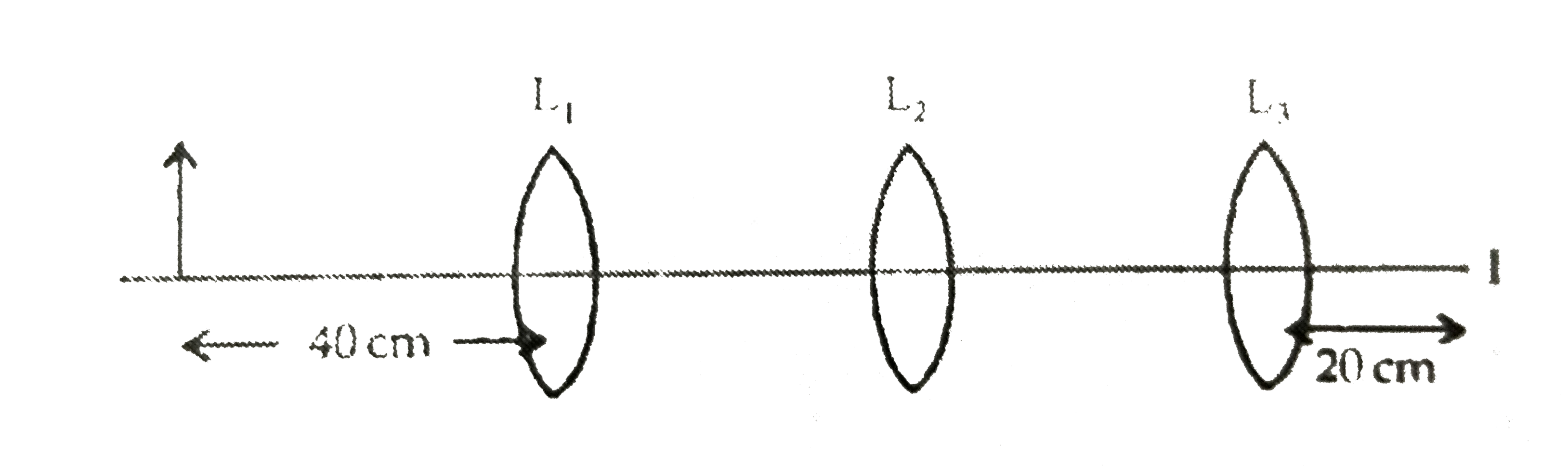
View SolutionYou are given three lenses L1,L2andL3 each of focal length 10 cm. An object is kept at 15 cm in front of L1, as shown. The final real image is formed at the focus 'T' of L3. Find the separations between L1,L2andL3.
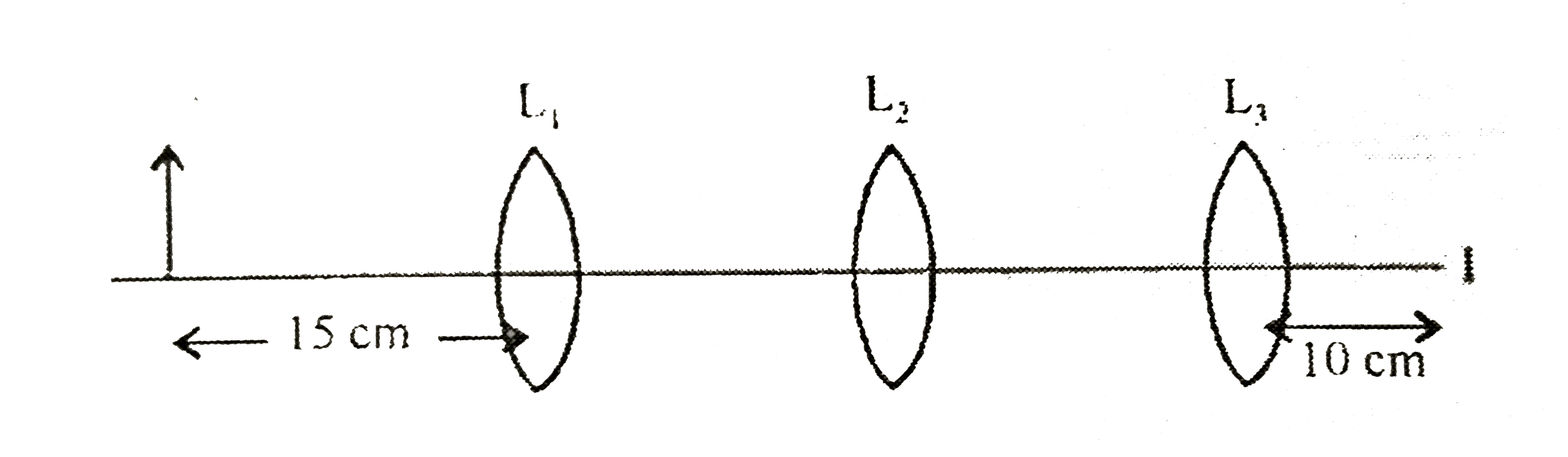
View SolutionABC is a right-angled triangle in which ∠B=90∘andBC=a. If n points L1,L2,…,Ln on AB is divided in n+1 equal parts and L1M1,L2M2,…,LnMn are line segments paralllel to BC and M1,M2,….,Mn are on AC, then the sum of the lengths of L1M1,L2M2,...,LnMn is
View SolutionConsider two intersecting (non-perpendicular lines L1=0andL2=0 and a point P1. Image of P1 and L1=0 is P2, image of P2 in L2=0 is P3 , image of P3 in L1 =0 is P4 and so on. Which of the following statements are incorrect ?
View SolutionFind the shortest distance between the lines l1andl2 given by
x−1−1=y3=z−22andx2=y−1−6=z−4
View SolutionThe combined equation of the lines L1 and L2 is 2x2+6xy+y2=0 and that lines L3 and L4 is 4x2+18xy+y2=0. If the angle between L1 and L4 be α, then the angle between L2 and L3 will be
View Solution