Equation of the circumcircle of a triangle formed by the lines L1=0,L2=0andL3=0 can be written as L1L2+λL2L3+μL3L1=0, where λandμ are such that coefficient of x2 =coefficient of y2 and coefficient of xy=0.
If L1L2+λL2L3+μL3L1=0 is such that μ=0andλ is non-zero, then it represents
a parabola
a pair of straight lines
a circle
an ellipse
The correct Answer is:B
To solve the problem, we need to analyze the given equation of the circumcircle of a triangle formed by the lines L1=0, L2=0, and L3=0. The equation can be expressed as:
L1L2+λL2L3+μL3L1=0
where λ and μ are coefficients that we need to determine under the conditions that the coefficient of x2 equals the coefficient of y2 and the coefficient of xy=0.
Step 1: Understanding the Lines
The lines L1=0, L2=0, and L3=0 represent three lines in the Cartesian plane. The intersection points of these lines will form the vertices of the triangle.
Step 2: Setting Up the Equation
The circumcircle of the triangle can be represented by the equation:
L1L2+λL2L3+μL3L1=0
Step 3: Analyzing the Coefficients
We know that:
- The coefficient of x2 is equal to the coefficient of y2.
- The coefficient of xy=0.
This means we need to ensure that the terms involving λ and μ satisfy these conditions.
Step 4: Substituting Values
Given that μ=0 and λ is non-zero, we can simplify the equation to:
L1L2+λL2L3=0
Step 5: Evaluating the Equation
Now, substituting L1,L2,L3 with their respective values (which are functions of x and y), we can analyze the behavior of the equation.
Step 6: Conclusion
Since L1,L2,L3 represent the lines that form the triangle, and the equation represents a curve, we can conclude that the equation represents a curve passing through the points of intersection of the lines L1=0, L2=0, and L3=0.
Final Answer
Thus, the final answer is that the equation represents a curve passing through the points of intersection of the lines L1=0, L2=0, and L3=0.
---
The lines L1=0, L2=0, and L3=0 represent three lines in the Cartesian plane. The intersection points of these lines will form the vertices of the triangle.
The circumcircle of the triangle can be represented by the equation:
We know that:
- The coefficient of x2 is equal to the coefficient of y2.
- The coefficient of xy=0.
Given that μ=0 and λ is non-zero, we can simplify the equation to:
Now, substituting L1,L2,L3 with their respective values (which are functions of x and y), we can analyze the behavior of the equation.
Since L1,L2,L3 represent the lines that form the triangle, and the equation represents a curve, we can conclude that the equation represents a curve passing through the points of intersection of the lines L1=0, L2=0, and L3=0.
Thus, the final answer is that the equation represents a curve passing through the points of intersection of the lines L1=0, L2=0, and L3=0.
Topper's Solved these Questions
- CIRCLE BOOK - ARIHANT MATHS ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise (Single Integer Answer Type Questions)
9 Videos
- CIRCLE BOOK - ARIHANT MATHS ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise (Statement I And Ii Type Questions)
7 Videos
- CIRCLE BOOK - ARIHANT MATHS ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise (More Than One Correct Option Type Questions)
15 Videos
- BIONOMIAL THEOREMBOOK - ARIHANT MATHS ENGLISHCHAPTER - BIONOMIAL THEOREMEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
- COMPLEX NUMBERSBOOK - ARIHANT MATHS ENGLISHCHAPTER - COMPLEX NUMBERSEXERCISE - Complex Number Exercise 8
2 Videos
Similar Questions
Equation of the circumcircle of a triangle formed by the lines L1=0,L2=0andL3=0 can be written as L1L2+λL2L3+μL3L1=0, where λandμ are such that coefficient of x2 =coefficient of y2 and coefficient of xy=0.
L1L22+λL2L23+μL21=0 represents
View SolutionThree sided of a triangle have equations L1≡y−mix=o;i=1,2and 3. Then L1L2+λL2L3+μL3L1=0 where λ≠0,μ≠0, is the equation of the circumcircle of the triangle if (a)1+λ+μ=m1m2+λm2m3+λm3m1 (b)m1(1+μ)+m2(1+λ)+m3(μ+λ)=0 (c)1m3+1m1+1m1=1+λ+μ (d)none of these
View SolutionConsider the lines given by
L1:x+3y−5=0
L2:3x−ky−1=0
L3:5x+2y−12=0
Match the following lists.

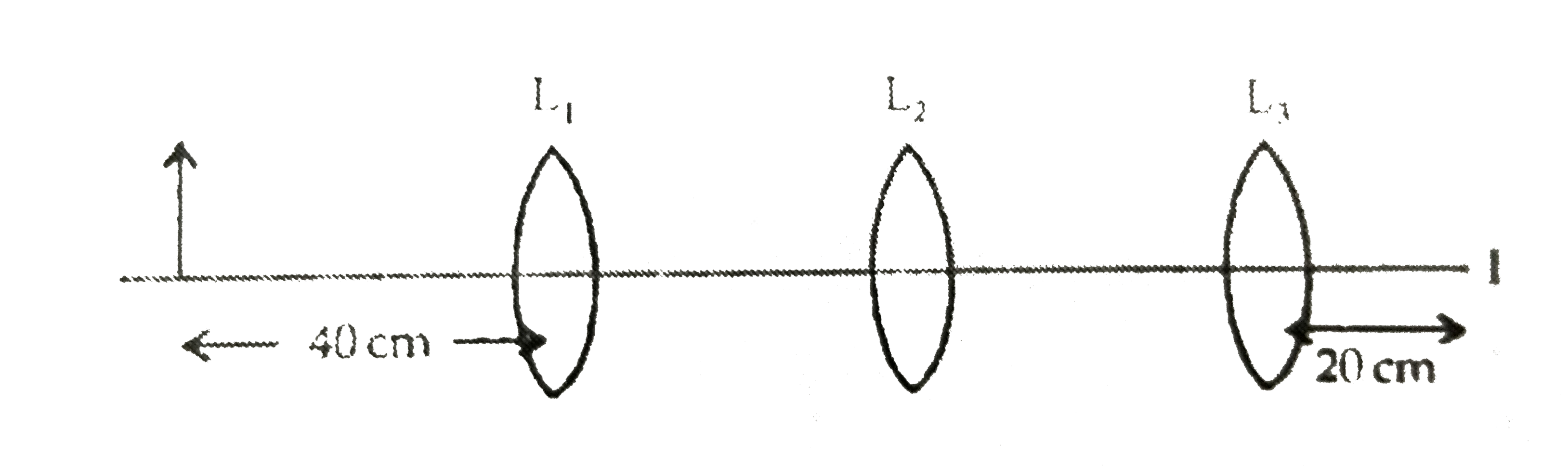
View SolutionYou are given three lenses L1,L2andL3 each of focal length 20 cm. An object is kept at 40 cm in front of L1, as shown. The final real image is formed at the focus 'I' of L3. Find the separations between L1,L2andL3.
View SolutionYou are given three lenses L1,L2andL3 each of focal length 10 cm. An object is kept at 15 cm in front of L1, as shown. The final real image is formed at the focus 'T' of L3. Find the separations between L1,L2andL3.
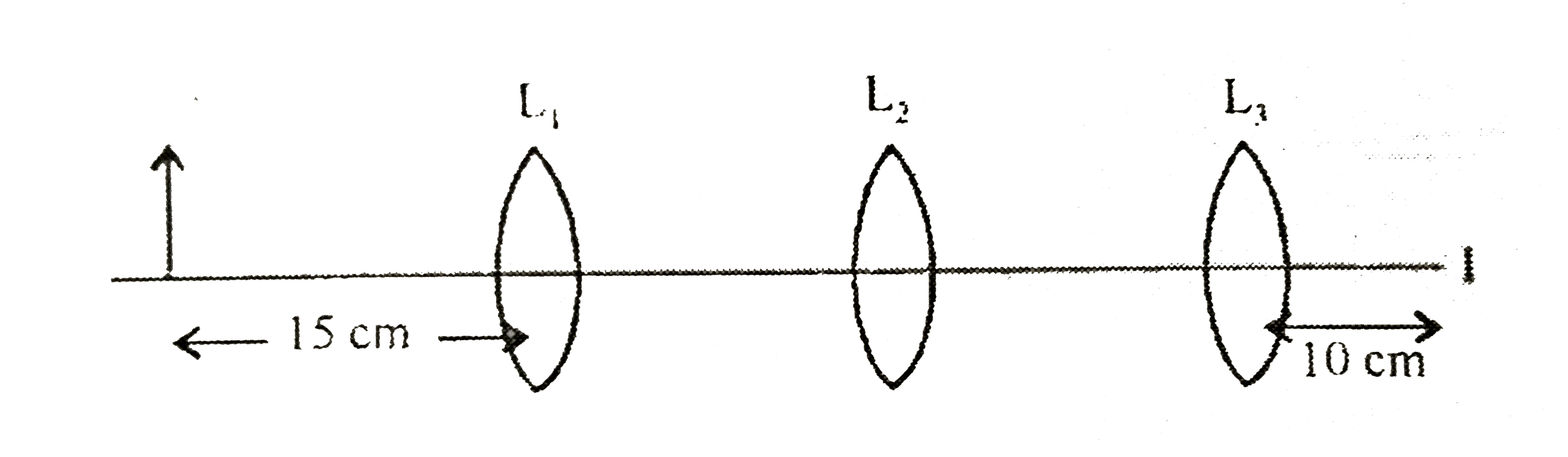
View SolutionLet the lines l1 and l2 be normals to y2=4x and tangents to x2=−12y (where l1andl2 are not x - axis). The absolute value of the difference of slopes of l1andl2 is
View SolutionConsider two intersecting (non-perpendicular lines L1=0andL2=0 and a point P1. Image of P1 and L1=0 is P2, image of P2 in L2=0 is P3 , image of P3 in L1 =0 is P4 and so on. Which of the following statements are incorrect ?
View SolutionL1=L0+rt. What does L(0) represent in the given expression?
View SolutionLet L1≡ax+by+a3√b=0andL2≡bx−ay+b3√a=0 be two straight lines . The equations of the bisectors of the angle formed by the foci whose equations are λ1L1−λ2L2=0andλ1l1+λ2=0,λ1andλ2 being non - zero real numbers ,are given by
View SolutionThe combined equation of the lines L1 and L2 is 2x2+6xy+y2=0 and that lines L3 and L4 is 4x2+18xy+y2=0. If the angle between L1 and L4 be α, then the angle between L2 and L3 will be
View Solution
