In ΔABC,AB=AC and the bisectors of angles B and C intersect at point O. Prove that
BO=OC
The correct Answer is:BO=CO
To prove that BO=OC in triangle ABC where AB=AC and the angle bisectors of angles B and C intersect at point O, we can follow these steps:
1. Draw Triangle ABC:
- Construct triangle ABC such that AB=AC. This makes triangle ABC isosceles.
2. Label Angles:
- Let the angles at vertices B and C be ∠B and ∠C respectively. Since AB=AC, we know that ∠B=∠C (angles opposite to equal sides are equal).
3. Draw Angle Bisectors:
- Draw the angle bisector of ∠B which intersects AC at point O.
- Similarly, draw the angle bisector of ∠C which intersects AB at point O.
4. Identify Angles:
- Let ∠XOB be the angle formed by the angle bisector of ∠B and ∠YOC be the angle formed by the angle bisector of ∠C.
- Since O is the intersection of the angle bisectors, we have ∠XOB=∠YOC.
5. Use Vertically Opposite Angles:
- The angles ∠BOX and ∠COY are vertically opposite angles, hence ∠BOX=∠COY.
6. Use the Angle Bisector Property:
- The angles ∠XBO and ∠YCO are equal because they are half of ∠B and ∠C respectively (as they are angle bisectors). Therefore, ∠XBO=∠YCO.
7. Establish Equality of Angles:
- Since ∠B=∠C, we can say that:
12∠B=12∠C⟹∠XBO=∠YCO
8. Consider Triangles BOX and COY:
- In triangles BOX and COY:
- ∠XOB=∠YOC (from step 4)
- ∠BOX=∠COY (from step 5)
- ∠XBO=∠YCO (from step 6)
9. Apply the Angle-Angle (AA) Criterion:
- Since two angles in triangle BOX are equal to two angles in triangle COY, by the Angle-Angle (AA) criterion, triangles BOX and COY are similar.
10. Conclude with Side Ratios:
- From the similarity of triangles BOX and COY, the sides opposite to equal angles are equal. Therefore, we conclude that:
BO=OC
- Construct triangle ABC such that AB=AC. This makes triangle ABC isosceles.
- Let the angles at vertices B and C be ∠B and ∠C respectively. Since AB=AC, we know that ∠B=∠C (angles opposite to equal sides are equal).
- Draw the angle bisector of ∠B which intersects AC at point O.
- Similarly, draw the angle bisector of ∠C which intersects AB at point O.
- Let ∠XOB be the angle formed by the angle bisector of ∠B and ∠YOC be the angle formed by the angle bisector of ∠C.
- Since O is the intersection of the angle bisectors, we have ∠XOB=∠YOC.
- The angles ∠BOX and ∠COY are vertically opposite angles, hence ∠BOX=∠COY.
- The angles ∠XBO and ∠YCO are equal because they are half of ∠B and ∠C respectively (as they are angle bisectors). Therefore, ∠XBO=∠YCO.
- Since ∠B=∠C, we can say that:
12∠B=12∠C⟹∠XBO=∠YCO
- In triangles BOX and COY:
- ∠XOB=∠YOC (from step 4)
- ∠BOX=∠COY (from step 5)
- ∠XBO=∠YCO (from step 6)
- Since two angles in triangle BOX are equal to two angles in triangle COY, by the Angle-Angle (AA) criterion, triangles BOX and COY are similar.
- From the similarity of triangles BOX and COY, the sides opposite to equal angles are equal. Therefore, we conclude that:
BO=OC
Topper's Solved these Questions
- BOOK - ICSECHAPTER - TRIANGLES EXERCISE - 4 MARKS QUESTIONS24videos
TRIANGLES
- BOOK - ICSECHAPTER - TRIANGLES EXERCISE - QUESTIONS8videos
TRIANGLES
- BOOK - ICSECHAPTER - STATISTICS AND GRAPH WORK EXERCISE - TOPIC - 2 (4 Marks Questions)14videos
STATISTICS AND GRAPH WORK
- BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - EXERCISE 22(B)50videos
TRIGONOMETRICAL RATIOS
Similar Questions
In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O. Prove that :
(i) BO = CO
(ii) AO bisects angle BAC.View SolutionIn ABC,AB=AC, and the bisectors of angles B and C intersect at point O . Prove that BO=CO and the ray AO is the bisector of angles BAC.
View SolutionIn ABC, AB=AC, and the bisectors of angles B and C intersect at point O. Prove that BO=CO and the ray AO is the bisector of angle BAC
View SolutionIn the adjoining figure, AB>AC and the angle bisectors of ∠B and ∠C meet at point P. Prove that
PB>PC.
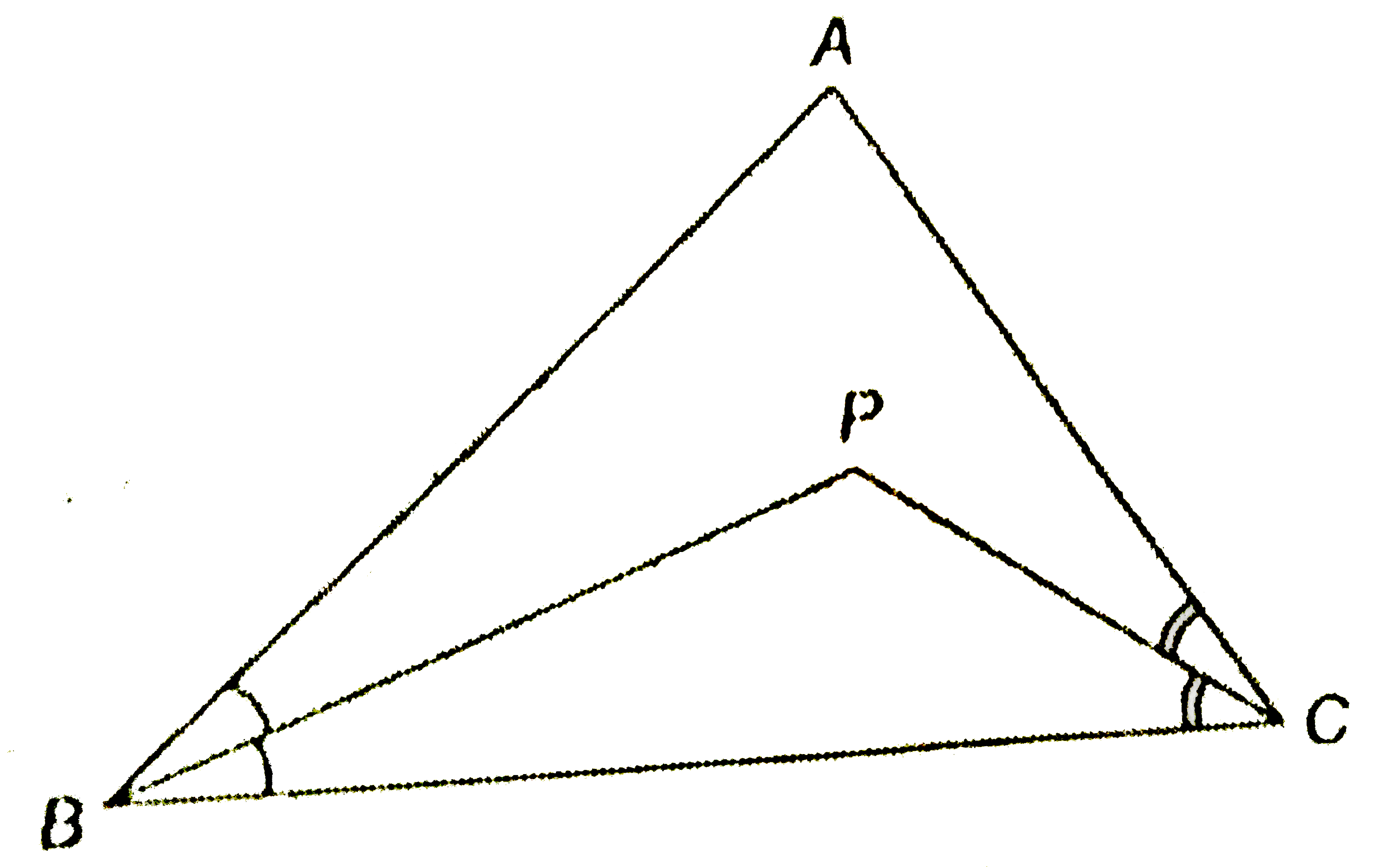 View Solution
View SolutionIn ΔABC,AB=AC and the bisectors of ∠B and ∠C meet AC and AB at point D and E respectively. Prove that BD=CE.
View SolutionIn parallelogram ABCD, the bisectors of adjacent angles A and D intersect each other at point P. prove that ∠APD=90∘.
View SolutionThe sides AB and AC of a triangle ABC are produced, and the bisectors of the external angles at B and C meet at P. Prove that if AB>AC, then PC>PB.
View SolutionIn a ABC, it is given that AB=AC and the bisectors of ∠B and C intersect at O. If M is a point on BO produced, prove that ∠MOC= ∠ABC
View SolutionIn triangle ABC,the bisector of interior angle A and the bisector angle C meet at point O. Prove that ∠AOC=12∠B
View SolutionIn triangle ABC , the internal bisector of ∠A∠Band∠C meet at point I . Prove that :
12(AB+BC+CA)<AI+BI+CIView Solution

