In the following, AB=EF, BC=De and ∠E=90∘
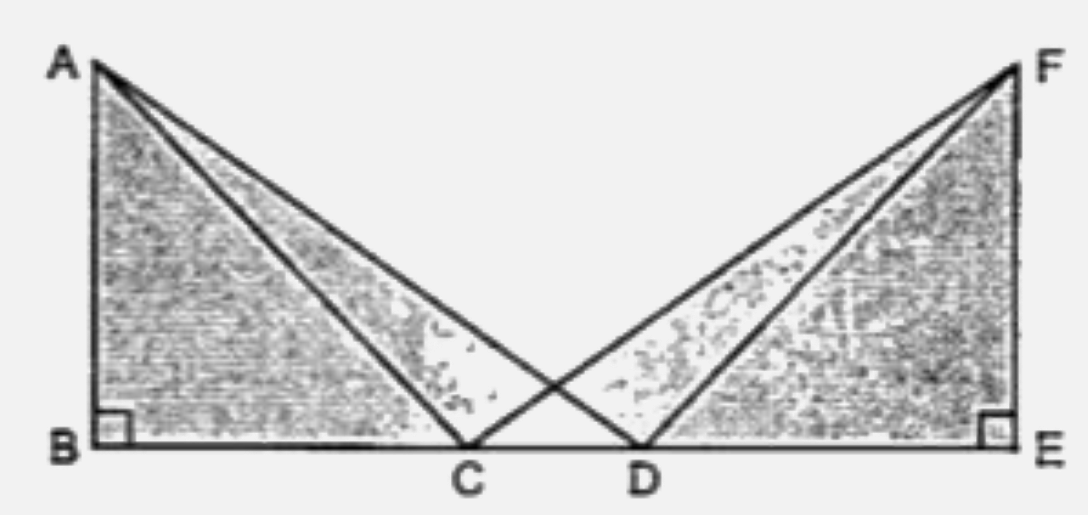
Prove that AD=FC
The correct Answer is:AD=FC
Step by step video & image solution for In the following, AB=EF, BC=De and angleE=90^(@) Prove that AD=FC by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - 4 MARKS QUESTIONS
24 Videos
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - QUESTIONS
8 Videos
- STATISTICS AND GRAPH WORK BOOK - ICSECHAPTER - STATISTICS AND GRAPH WORK EXERCISE - TOPIC - 2 (4 Marks Questions)
14 Videos
- TRIGONOMETRICAL RATIOS BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - EXERCISE 22(B)
50 Videos
Similar Questions
In the adjoining figure, AB=EF,BC=DE,∠ABC=∠FED=90∘, prove that AD=CF.
View SolutionIn the following figure, AB = EF, BC = DE and ∠B=∠E=90∘.
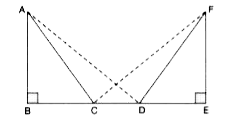
Pove that AD = FC.
View SolutionIn the given figure, AC=AE,AB=AD and ∠BAD=∠EAC. Prove that BC=DE.
View SolutionIn the following diagram, AD = AB and AE bisects angle A. Prove that :
(i) BE = DE (ii) ∠ABD>∠C
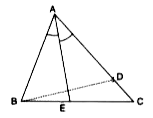
View SolutionIn the following figure , AB = BC , AD ⊥BCandCE⊥AB.prove that AD = CE
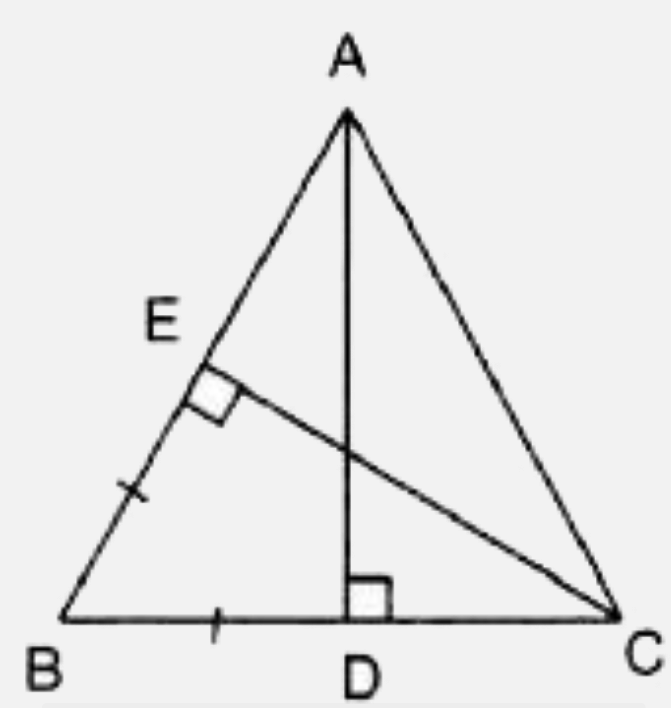
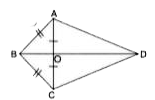
View SolutionIn the following figure, OA=OC and AB=BC.
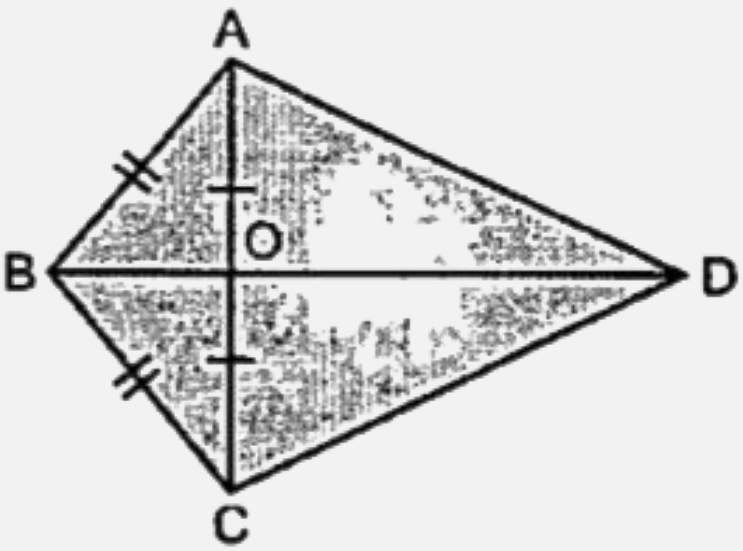
Prove that :
∠AOB=90∘
View SolutionIn the following figure, OA = OC and AB = BC. Prove that :
(i) ∠AOB=90∘
(ii) ΔAOD≅ΔCOD
(iii) AD = CD
View SolutionIn a quadrilateral ABCD ∠B=∠D=90∘ Prove that : 2AC2−BC2=AB2+AD2+DC2
View SolutionIn the following figure AB = AD , AC = AE and ∠BAD=∠CAE,
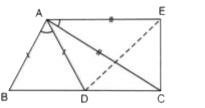
Prove that :BC = ED
View SolutionIn a quadrilateral ABCD, ∠A+∠D=90∘. Prove that
AC2+BD2=AD2+BC2
View Solution
