A small solid marble of mass M and radius r rolls down along the loop track, without slipping. Find the height h above the base, from where it has to start rolling down the incline such that the sphere just completes the vertical circular loop of radius R.
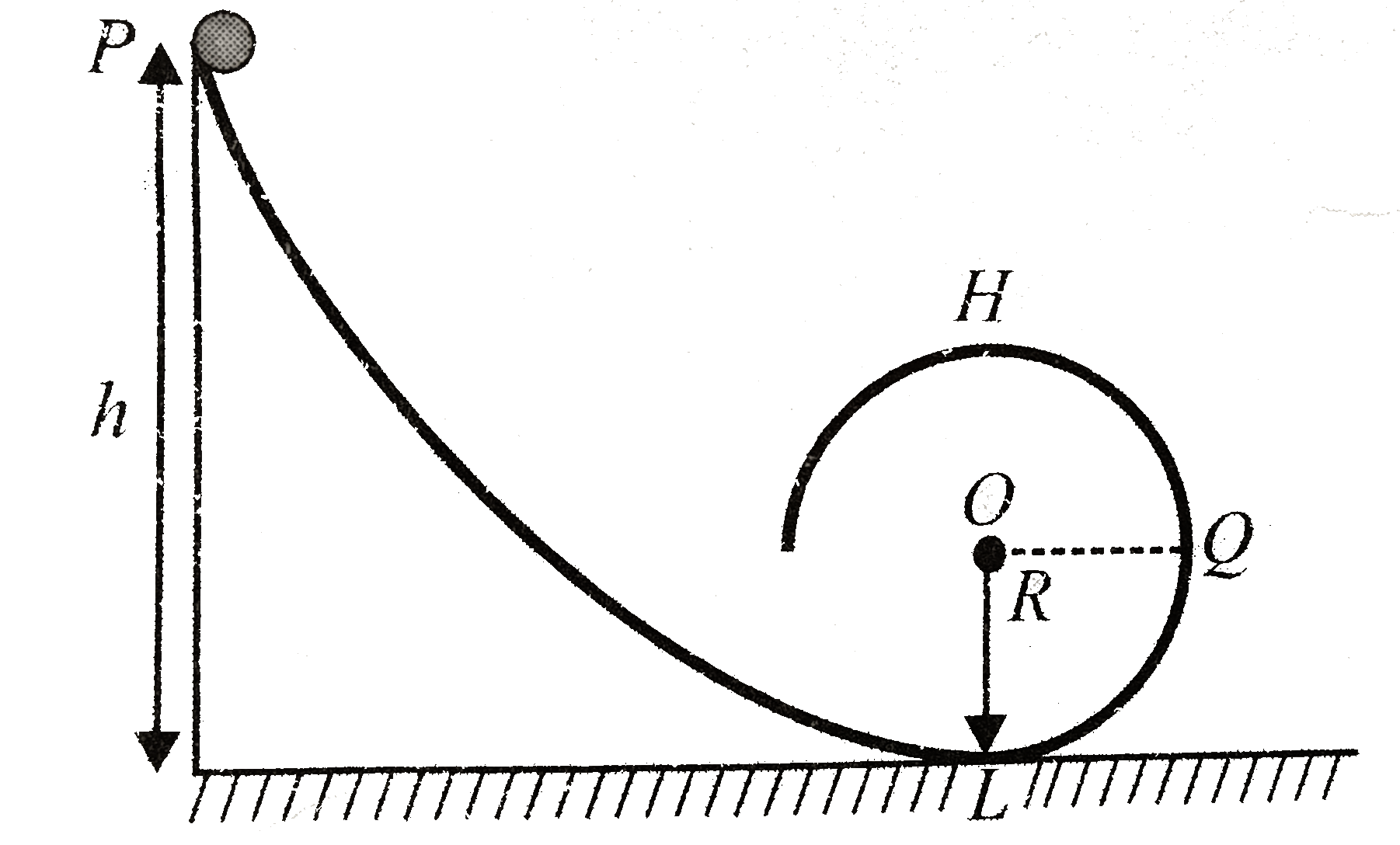
A small solid marble of mass M and radius r rolls down along the loop track, without slipping. Find the height h above the base, from where it has to start rolling down the incline such that the sphere just completes the vertical circular loop of radius R.

A

52R

B

52(R−r)

C

2510(R−r)

D

2710R−17r10

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:D

The minimum velolcity of P, topo of the loop, should be v=√g(R−r) if the sphere keeps on rolling at top v=ωR
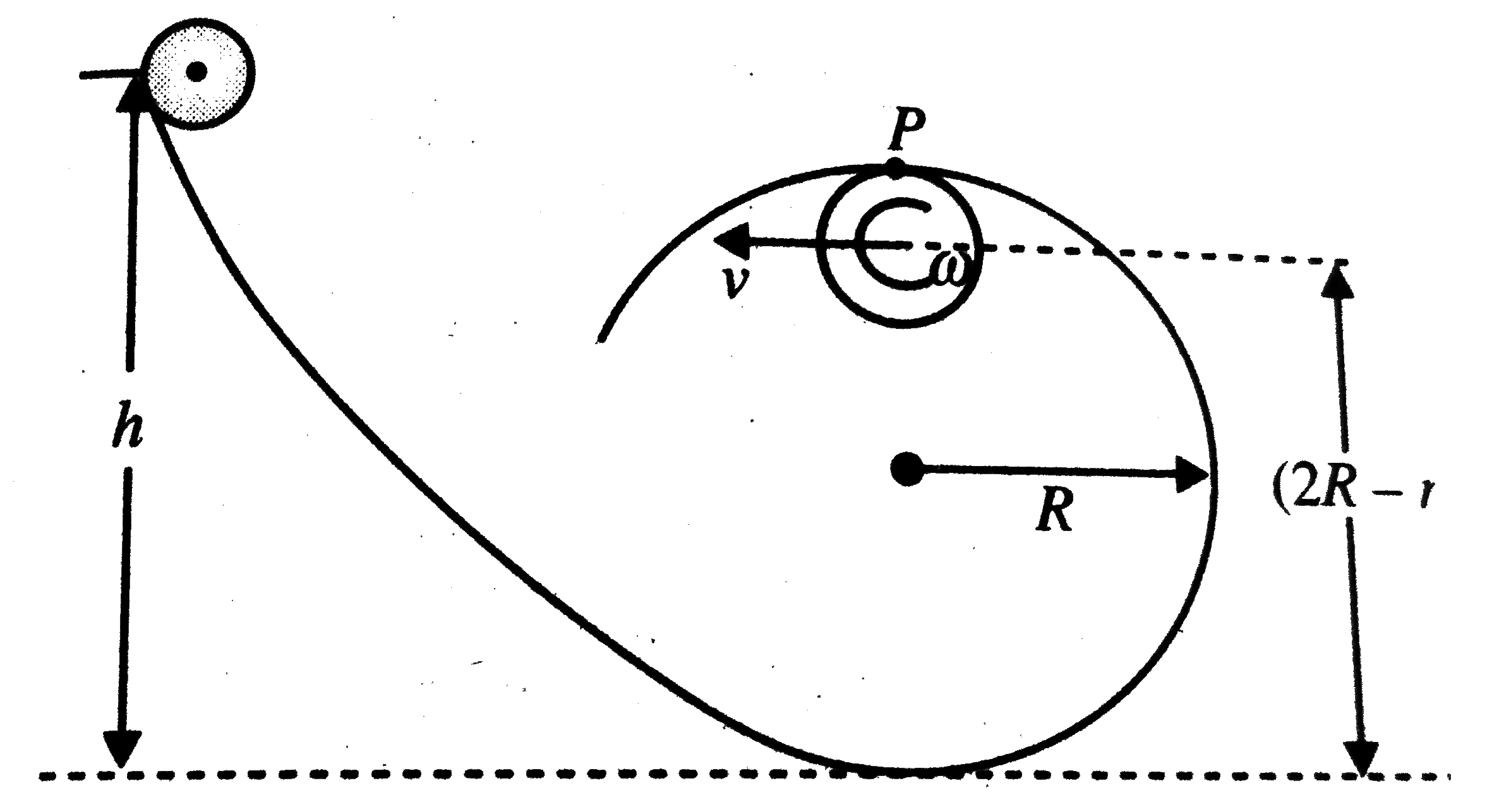
ocr_image
mgh=12mv2+12Iω2+mg(2R−r)
=12mg(R−r)+12(25)mR22ω2+mg(2R−r)
=710mg(R−r)+mg(2R−r)[ωr=v=√g(R−r)]
=mg10(27R−17r)orh=110(27r−17r)

Updated on:21/07/2023
|
Class 11PHYSICSRIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A solid cylinder of mass M and radius R rolls down an inclined plane without slipping. THE speed of its centre of mass when it reaches the bottom is

    A√(2gh)
    B√4/3.gh
    C√3/4.gh
    D√(4g/h)

  • A solid cylinder of mass M and radius R rolls without slipping down an inclined plane making an angle 6 with the horizontal. Then its acceleration is.

    A1/3 g sinθ
    B2/3 g sinθ
    C2/5 g sinθ
    D2/7 g sinθ