If the container filled with liquid gets accelerated horizontally or vertically, pressure in liquid gets changed. In liquid (ay) for calculation of pressure, effective g is used. A closed box horizontal base 6m by 6m and a height 2m is half filled with liquid. It is given a constant horizontal acceleration g/2 and vertical downward acceleration g/2.
The angle of the free surface with the horizons is equal to
30∘
tan−123
tan−1 13
45∘
The correct Answer is:D
To find the angle of the free surface of the liquid in the container that is being accelerated both horizontally and vertically, we can follow these steps:
Step 1: Identify the accelerations
The container is experiencing:
- A horizontal acceleration ax=g2 (to the right)
- A vertical downward acceleration ay=g2
Step 2: Determine the effective gravitational acceleration
When the container is accelerated, the effective gravitational acceleration geffective can be calculated by considering both the gravitational force and the pseudo forces due to the acceleration of the container.
The effective gravitational acceleration can be represented as a vector:
- The downward component is g−ay=g−g2=g2
- The horizontal component is ax=g2
Thus, we have:
- geffective=√(g−ay)2+a2x=√(g2)2+(g2)2
Step 3: Calculate geffective
Calculating geffective:
geffective=√(g2)2+(g2)2=√g24+g24=√g22=g√2
Step 4: Determine the angle of the free surface
The angle θ that the free surface makes with the horizontal can be found using the tangent function:
tan(θ)=vertical componenthorizontal component=g−ayax=g2g2=1
Step 5: Calculate θ
Since tan(θ)=1, we can find θ:
θ=tan−1(1)=45∘
Conclusion
The angle of the free surface with the horizontal is 45∘.
---
The container is experiencing:
- A horizontal acceleration ax=g2 (to the right)
- A vertical downward acceleration ay=g2
When the container is accelerated, the effective gravitational acceleration geffective can be calculated by considering both the gravitational force and the pseudo forces due to the acceleration of the container.
- The downward component is g−ay=g−g2=g2
- The horizontal component is ax=g2
- geffective=√(g−ay)2+a2x=√(g2)2+(g2)2
Calculating geffective:
geffective=√(g2)2+(g2)2=√g24+g24=√g22=g√2
The angle θ that the free surface makes with the horizontal can be found using the tangent function:
tan(θ)=vertical componenthorizontal component=g−ayax=g2g2=1
Since tan(θ)=1, we can find θ:
θ=tan−1(1)=45∘
The angle of the free surface with the horizontal is 45∘.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - True/False
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - SCQ_TYPE
13 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
If the container filled with liquid gets accelerated horizontally or vertically, pressure in liquid gets changed. In liquid (ay) for calculation of pressure, effective g is used. A closed box horizontal base 6m by 6m and a height 2m is half filled with liquid. It is given a constant horizontal acceleration g/2 and vertical downward acceleration g/2.
The maximum value of water pressure in the box is equal to
View SolutionIf the container filled with liquid gets accelerated horizontally or vertically, pressure in liquid gets changed. In liquid (ay) for calculation of pressure, effective g is used. A closed box horizontal base 6m by 6m and a height 2m is half filled with liquid. It is given a constant horizontal acceleration g/2 and vertical downward acceleration g/2.
Length of exposed portion of top of box is equal to
View SolutionIf the container filled with liquid gets accelerated horizontally or vertically, pressure in liquid gets changed. In liquid (ay) for calculation of pressure, effective g is used. A closed box horizontal base 6m by 6m and a height 2m is half filled with liquid. It is given a constant horizontal acceleration g/2 and vertical downward acceleration g/2.
Water pressure at the bottomof centre of the box is equal to (atmospheric pressure =105N/m2 density of water =1000kg/m3,g=10m/s2)
View SolutionIf the container filled with liquid gets accelerated horizontally or vertically, pressure in liquid gets changed. In liquid (ay) for calculation of pressure, effective g is used. A closed box horizontal base 6m by 6m and a height 2m is half filled with liquid. It is given a constant horizontal acceleration g/2 and vertical downward acceleration g/2.
What is the value of vertical acceleration of box for given horizontal acceleration (g/2), so that no part of the bottom of the box is exposed?
View SolutionA vessel contains a liquid has a constant acceleration 19.6m/s2 in horizontal direction. The free surface of water get sloped with horizontal at angle
View SolutionA liquid kept in a container has a horizontal acceleration 'a' as shown in figure.
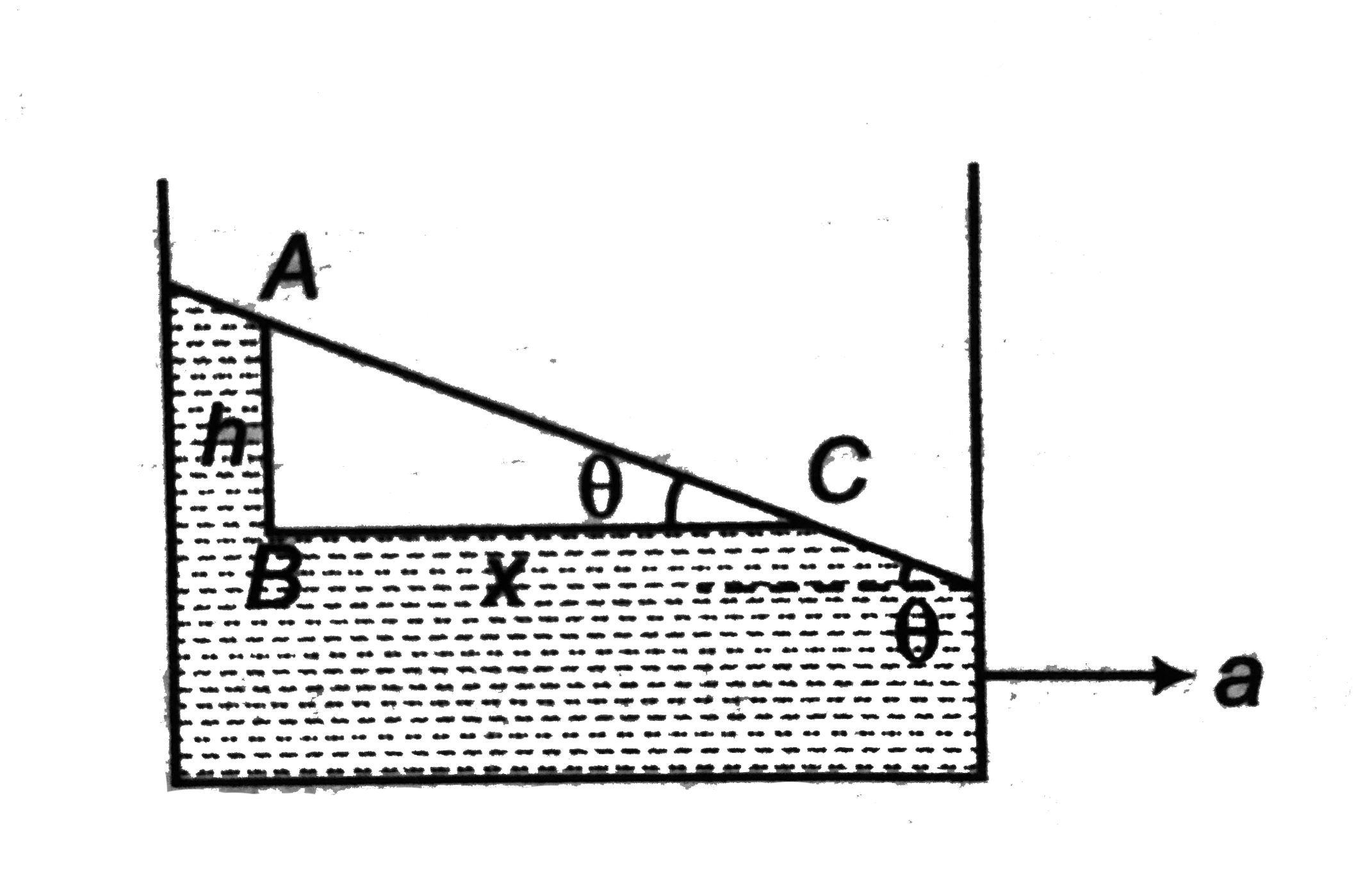
Using the pressure equation along the path ABC find the angle θ.
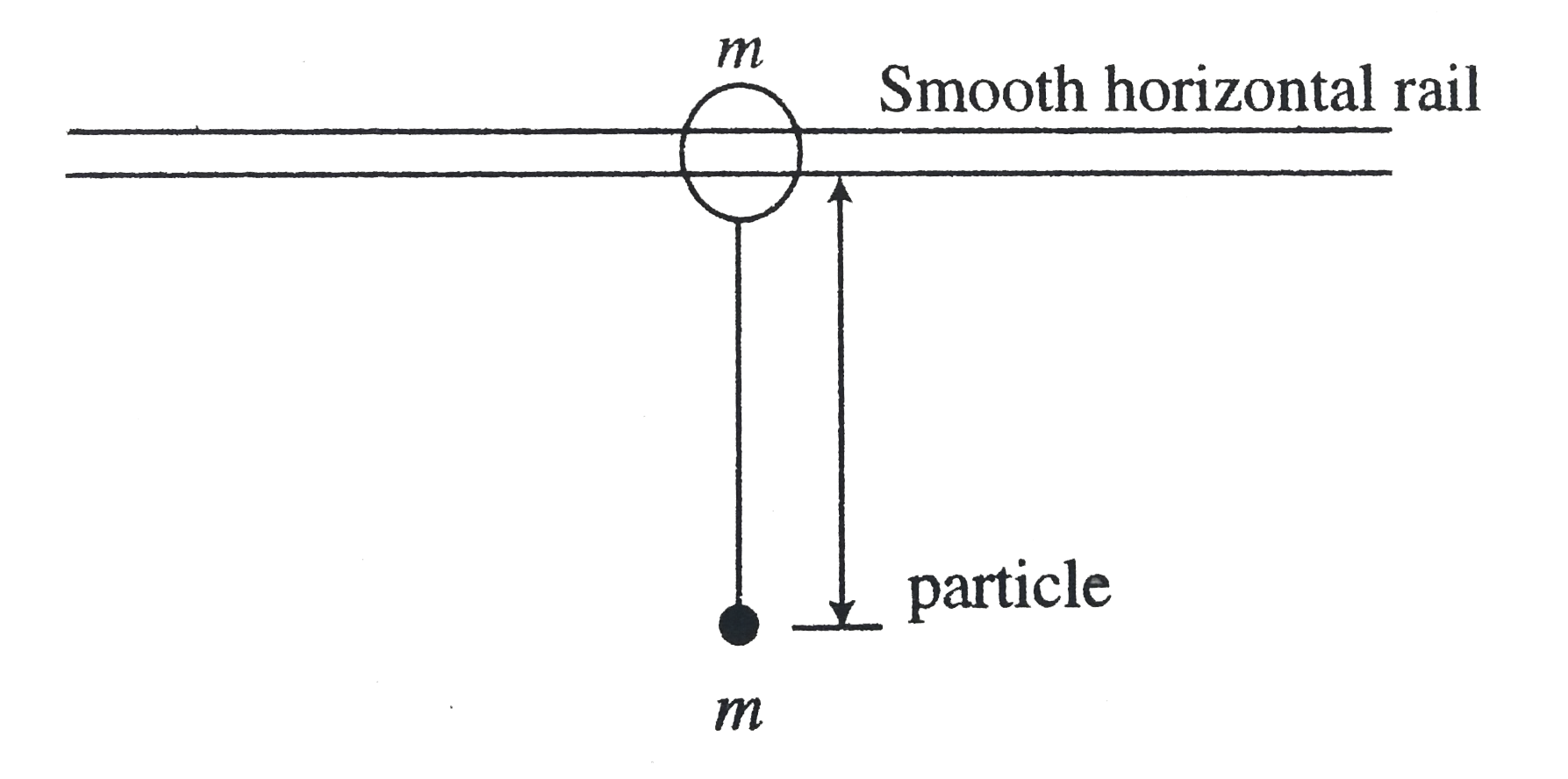
View SolutionThe ring shown in fig. is given a constant horizontal acceleration (a0=g√3). The maximum deflection of the string from the vertical is θ0. Then
View SolutionA trolley containing a liquid slides down a smooth inclined plane of angle α with the horizontal. Find the angle of inclination θ of the free surface with the horizontal.

View SolutionA container containing a liquid is moving down an incline plane with constant acceleration 'a' . The angle made by the water surface in equilibrium with the inclined plane is

View Solution
A sealed tank containing a liquid of density ρ moves with a horizontal acceleration a, as shown in the figure. The difference in pressure between the points A and B is
View Solution
