In the previous problem, if two falling conductors attain velocities respectively after falling through same height h, ratio of energy dissipated as heat to the energy dissipated in resistor per unit time for two conductors is same.
Find which of the following condition will be satisfied
The correct Answer is:A
To solve the problem, we need to analyze the energy dissipated as heat and the energy dissipated in the resistor per unit time for two falling conductors. Let's denote the two conductors as conductor 1 and conductor 2, with velocities v1 and v2 respectively after falling through the same height h.
Step 1: Write the energy conservation equation for both conductors
For each conductor, the potential energy lost while falling through height h is converted into kinetic energy and energy dissipated in the resistor. The energy conservation equation can be written as:
For conductor 1:
mgh=12mv21+Edissipated1
For conductor 2:
mgh=12mv22+Edissipated2
Where Edissipated1 and Edissipated2 are the energies dissipated in the resistors for conductors 1 and 2 respectively.
Step 2: Rearranging the equations
From the above equations, we can express the energies dissipated as:
Edissipated1=mgh−12mv21
Edissipated2=mgh−12mv22
Step 3: Calculate the ratio of energies dissipated as heat to the energy dissipated in resistors per unit time
The energy dissipated in the resistor per unit time can be expressed as:
P1=Edissipated1tandP2=Edissipated2t
Thus, the ratio of the energy dissipated as heat to the energy dissipated in the resistor per unit time for both conductors can be written as:
Edissipated1P1=mgh−12mv21Edissipated1tandEdissipated2P2=mgh−12mv22Edissipated2t
Step 4: Set the ratios equal to each other
According to the problem, these ratios are equal:
mgh−12mv21Edissipated1t=mgh−12mv22Edissipated2t
Step 5: Simplifying the equation
After simplification, we can equate the two expressions:
mgh−12mv21=mgh−12mv22
This leads to:
12mv21=12mv22
Step 6: Finding the condition
From the above, we can conclude:
v21=v22
Thus, the condition that will be satisfied is:
v1=v2orv1=−v2
Conclusion
The condition that will be satisfied is that the velocities of the two conductors after falling through the same height are equal.
mgh=12mv21+Edissipated1
mgh=12mv22+Edissipated2
Edissipated1=mgh−12mv21
Edissipated2=mgh−12mv22
P1=Edissipated1tandP2=Edissipated2t
Edissipated1P1=mgh−12mv21Edissipated1tandEdissipated2P2=mgh−12mv22Edissipated2t
mgh−12mv21Edissipated1t=mgh−12mv22Edissipated2t
mgh−12mv21=mgh−12mv22
12mv21=12mv22
v21=v22
v1=v2orv1=−v2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Multiple Correct34videos
MISCELLANEOUS VOLUME 5
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Linked Comprehension84videos
MISCELLANEOUS VOLUME 5
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False3videos
MISCELLANEOUS VOLUME 3
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.514videos
NUCLEAR PHYSICS
Similar Questions
In the above problem if m=1kg and teminal velocity attained by its is 4m/s after falling a height of 1m, the energy dissipated as heat till then is (g=10m/s2)
View SolutionIf two persons A and B take 2 seconds and 4 seconds respectively to lift an object to the same height h, then the ratio of their potential energies at highest point is
View Solution
Knowledge Check
- Question 1 - Select One
Two wires of the same material and length but diameter in the ratic 1: 2 are stretched by the same load. The ratio of elastic potential energy per unit volume for the two wires is
A1:1B2:1C4:1D16:1
The capacities of two conductors are C1 and C2 and their respectively potentials are V1 and V2. If they are connected by a thin wire, then the loss of energy will be given by
View SolutionTwo cars of same mass are moving with velocities v1andv2 respectively. If they are stopped by supplying same breaking power in time t1andt2 respectively then v1v2 is
View SolutionTwo bodies of masses m1andm2 fall from heights h1andh2 respectively. The ratio of their velocities when the hit the ground is
View SolutionA current of 2A is increasing at a rate of 4As−1 through a coil of inductance 1H. Find the energy stored in the inductor per unit time in the units of Js−1.
View SolutionIf two pieces of caesium metal are supplied with energy hv1andhv2 respectively and v1>v2 then which of the following relation does not hold true?
View SolutionTwo plates 1 and 2 move with velocities −v and 2v respectively. If the sphere does not slide relative to the plates, assuming the masses of each body as m, find the kinetic energy of the system (plates+sphere).
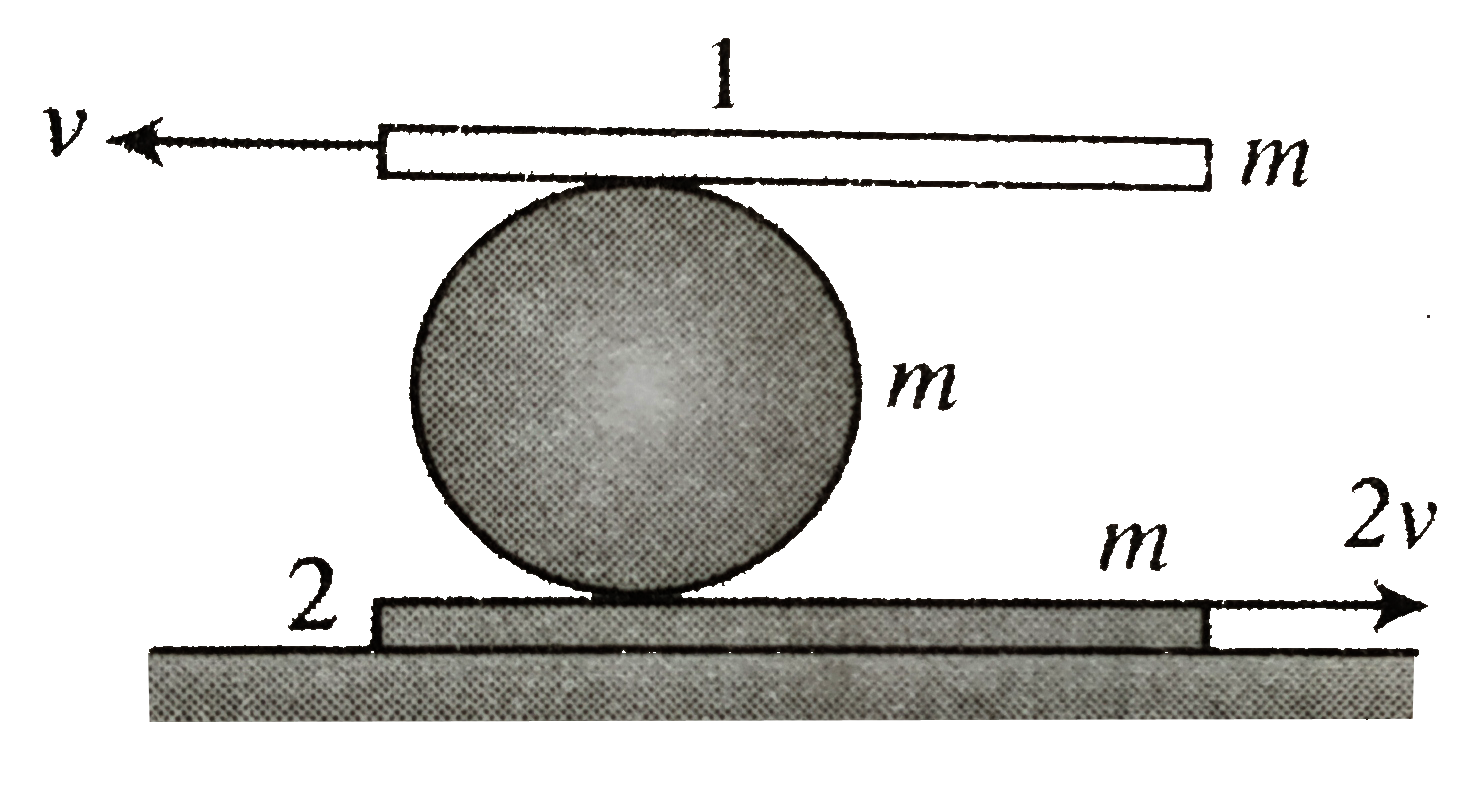 View Solution
View SolutionTwo bodies are thrown up at angles of 45∘ and 60∘, respectively, with the horizontal. If both bodies attain same vertical height, then the ratio of velocities with which these are thrown is
View Solution

