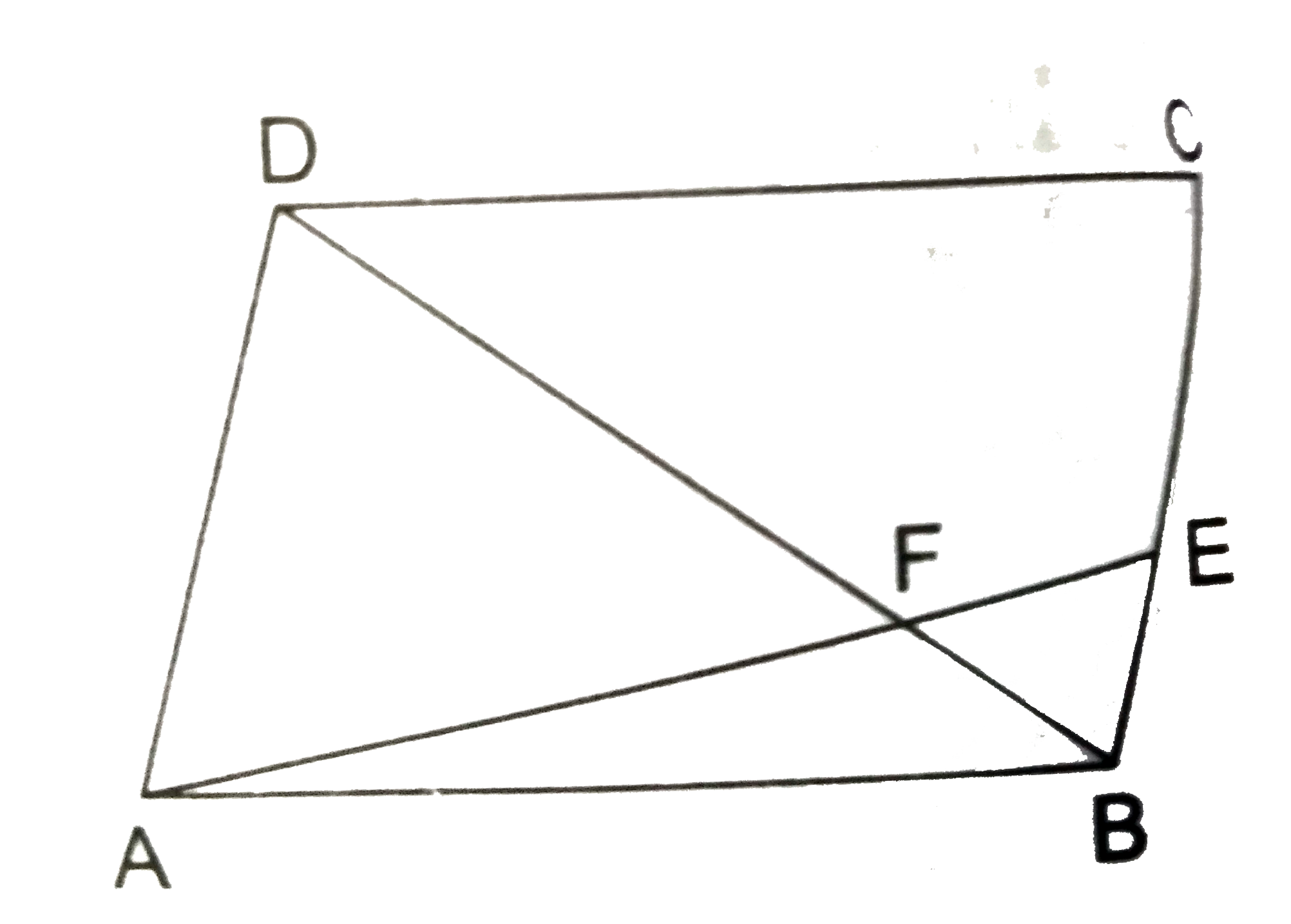
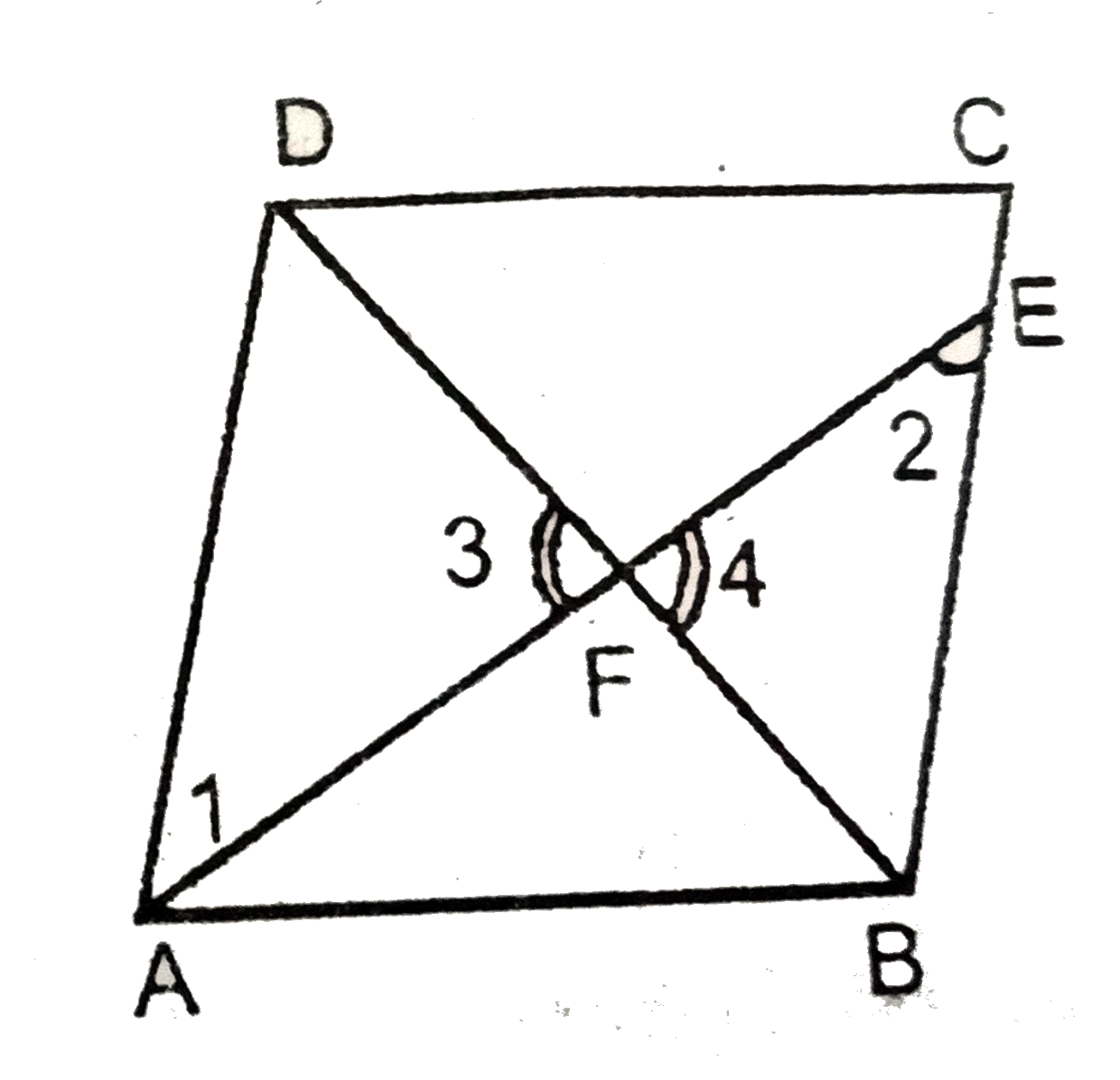
E is any point on the side BC of the parallelogram ABCD. AE intersects the diagonal BD at point F. Prove that DF×EF=FB×FA.Solution in Assamese
Step by step video & image solution for E is any point on the side BC of the parallelogram ABCD. AE intersects the diagonal BD at point F. Prove that DF×EF=FB×FA. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The ratio of the edges of a cuboid is 1:2:3 and its volume is 1,296cm3. What is the area of the whole surface?
View SolutionA line segment drawn parallel to the base BC of ΔABC intersects AB and AC at X and Y respectively.
If AB=7.2 , AC=4.8 and AX=4.2 then show that AY=2.8.
View SolutionThe diagonal BD of a parallelogram ABCD intersects the segment AE at the point F, where E is any point on the side BC . Prove that DFxEF=FBxFA.
View SolutionIn a trapezium ABCD, if E and F be the mid-points of diagonal AC and BD respectively. Prove that EF=12(AB−CD).

View SolutionThe diagonal BD of a parallelogram ABCD intersects the segment AE at the point F, where E is any point on the Side BC. Prove that DF×EF=FB×FA.
View SolutionABCD is a parallelogram and E is a point, on BC. If the diagonal BD intersects AE at F, prove that AF×FB×EF×FD.
View Solutionकिसी समान्तर चतुर्भुज ABCD का विकर्ण BD , रेखा AE को बिन्दु F पर प्रतिच्छेदित करता है , जहाँ बिन्दु E , भुजा BC पर स्थित है , तो सिद्ध कीजिए कि DF×EF=FB×FA.
View Solutionबगल के चित्र में समान्तर चतुर्भुज ABCD का विकर्ण BD रेखा खण्ड AE को F बिन्दु पर प्रतिच्छेद करता है , जहाँ E,BC भुजा पर कोई बिन्दु है । दिखाएँ कि DF×FE=BF×FA.
View Solutionएक समानांतर चतुर्भुज ABCD की भुजा BC , पर एक बिंदु E स्थित है|यदि इस समानांतर चतुर्भुज का विकर्ण BD रेखा AE को बिंदु F पर काटता हों,तो सिद्ध करो की: DF×EF×=FB×FA
View SolutionThe diagonal BD of parallelogram ABCD intersect AE at F as shown in the figure. If E is any point on BC, then prove that DF×EF=FB=FA.
View SolutionP is any point on the diagonal BD of the parallelogram ABCD. Prove that ΔAPD=ΔCPD.
View SolutionABCD एक समांतर चतुर्भुज है जिसके विकर्ण E बिंदु प्रतिच्छेद करते है। AC को F तक इस तरह बढाया गया हैं कि CF = AE सिध्द कीजिए। कि ar (ΔBDF)=ar(∣∣gmABCD)
View SolutionABCD is a parallelogram as shown in the figure. 'E' is a point on BC, the diagonal BD intersects AE in 'F'. Then prove that DF x EF = FB x FA.
View SolutionABCD is a quadrilateral in which AB=AD . The bisector of BAC and CAD intersect the sides BC and CD at the points E and F respectively. Prove that EF||BD.
View Solutionएक समान्तर चतुर्भुज ABCD में, विकर्ण BD,रेखाखण्ड AE को बिंदु F पर प्रतिच्छेद करता है। जहाँ भुजा BC पर कोई बिंदु E है, तो सिद्ध कीजिए कि DF×FE=BF×FA
View Solution
