A particle travels half the distance of a straight journey with a speed 5 m/s. The remaining part of the distance is covered with speed 6 m/s for half the remaining time, and with speed 4 m/s for the other half of the remaining time. The average speed of the particle is
3 m/s
4 m/s
34 m/s
5 m/s
The correct Answer is:D
To solve the problem step by step, we will calculate the average speed of the particle based on the given conditions.
Step 1: Define the total distance
Let the total distance of the journey from point A to point B be D. According to the problem, the particle travels half of this distance first, which means the distance from A to C (the midpoint) is D2.
Step 2: Calculate the time taken for the first half of the journey
The speed for the first half of the journey (from A to C) is given as 5 m/s. The time taken to cover this distance can be calculated using the formula:
Time=DistanceSpeed=D25=D10
Let this time be T′. Therefore, T′=D10.
Step 3: Analyze the second half of the journey
The remaining distance from C to B is also D2. This distance is covered in two parts:
- The first half of the remaining time is traveled at a speed of 6 m/s.
- The second half of the remaining time is traveled at a speed of 4 m/s.
Let the total time taken to cover the second half of the journey be T. Thus, the time for each part is:
- First half time: T2
- Second half time: T2
Step 4: Calculate the distances covered in the second half
The distance covered in the first half of the remaining time is:
Distance1=Speed×Time=6×T2=3T
The distance covered in the second half of the remaining time is:
Distance2=Speed×Time=4×T2=2T
The total distance for the second half of the journey is:
Total Distancesecond half=3T+2T=5T
Step 5: Calculate the total distance
Now, we can express the total distance D:
D=D2+D2=5T+D10
This gives us the equation:
D=5T
Step 6: Calculate the total time taken
The total time taken for the entire journey is:
Total Time=T′+T=D10+T
Substituting T from the previous equation T=D5:
Total Time=D10+D5=D10+2D10=3D10
Step 7: Calculate the average speed
The average speed Vavg is given by:
Vavg=Total DistanceTotal Time=D3D10=D×103D=103 m/s
Final Answer
The average speed of the particle is 103 m/s.
Let the total distance of the journey from point A to point B be D. According to the problem, the particle travels half of this distance first, which means the distance from A to C (the midpoint) is D2.
The speed for the first half of the journey (from A to C) is given as 5 m/s. The time taken to cover this distance can be calculated using the formula:
Time=DistanceSpeed=D25=D10
Let this time be T′. Therefore, T′=D10.
The remaining distance from C to B is also D2. This distance is covered in two parts:
- The first half of the remaining time is traveled at a speed of 6 m/s.
- The second half of the remaining time is traveled at a speed of 4 m/s.
- First half time: T2
- Second half time: T2
The distance covered in the first half of the remaining time is:
Distance1=Speed×Time=6×T2=3T
The distance covered in the second half of the remaining time is:
Distance2=Speed×Time=4×T2=2T
The total distance for the second half of the journey is:
Total Distancesecond half=3T+2T=5T
Now, we can express the total distance D:
D=D2+D2=5T+D10
This gives us the equation:
D=5T
The total time taken for the entire journey is:
Total Time=T′+T=D10+T
Substituting T from the previous equation T=D5:
Total Time=D10+D5=D10+2D10=3D10
The average speed Vavg is given by:
Vavg=Total DistanceTotal Time=D3D10=D×103D=103 m/s
The average speed of the particle is 103 m/s.
Topper's Solved these Questions
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - C)
7 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - D)
24 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - A)
50 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
Similar Questions
A particle travels along a straight line. It covers halg the distance with a speed (v). The remaining part of the distance was covere with speed v1 for half the time and with speed v2 for the other half the time . Find the average speed of the particle over the entire motion.
View SolutionA man traversed half the distance with a velocity v0. The remaining part of the distance was covered with velocity v1. For half the time and with velocity v2 for the other half of the time . Find the average speed of the man over the whole time of motion.
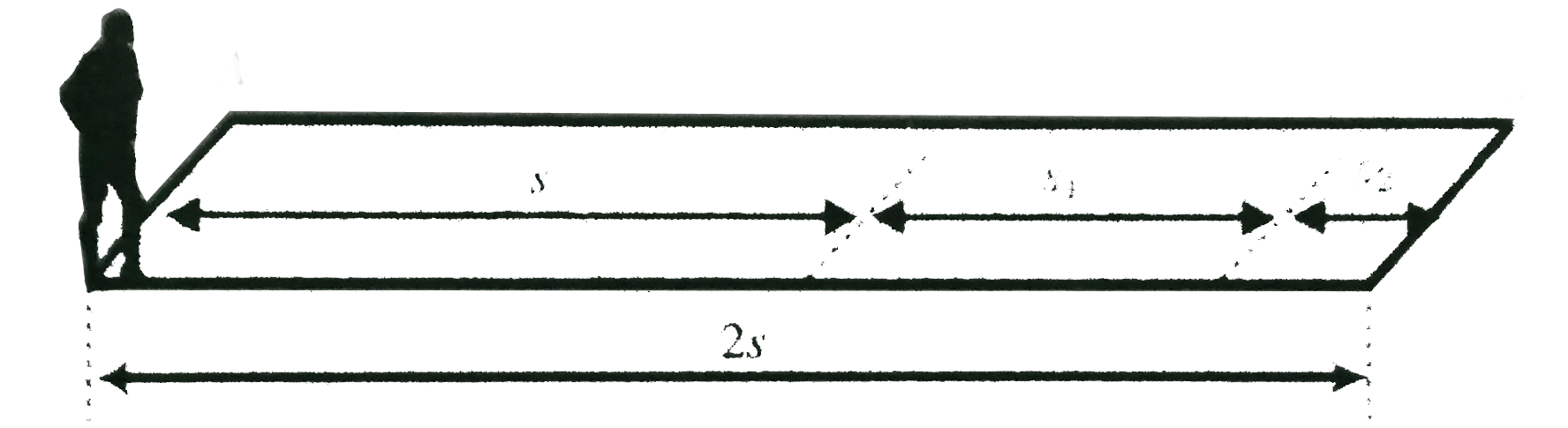 .
.
View Solution
Knowledge Check
A body moving along a straight line travels one third of the total distance with a speed of 3.0ms−1. The remaining distance is covered with a speed of 4.0ms−1 for half the time and 5.0ms−1 for the other half of the time. The average speed during the motion is
A4.0ms−1B6.0ms−1C3.8ms−1D2.4ms−1
A body travelling along a straight line traversed one third of the total distance with a velocity 4m/s. The remaining part of the distance was covered with a velocity 1 m/s for half the time and with velocity 3 m/s for the other half of time. The mean velocity averaged over the whole time of motion is :
View SolutionA particle traversed half of the distance with a velocity of V0.The remaining parts of the distance was covered with velocity V1, for half of the time and with V2 for other half of the time .Find the mean velocity of the particle averahed and the whole time of motion .
View SolutionA body travelling along a straight line , one thired of the total distance with a velocity 4ms−1. The remaining part of the distance was covered with a velocity 2ms−1 for half the time and with velocity 6ms−1 for the other half of time . What is the mean velocity averaged over te whle time of motin ?
View SolutionA man travels half time of its journey with speed of 5m/s and for remaining half time he moves half of its total distance travelled, with speed of 3 m/s and remaining half distance with speed of 6 m/s. Find out the average speed of the man?
View SolutionA train covers the first half of the distance between two stations with a speed of 40 km/h and the other half with 60 km/h. Then its average speed is :
View SolutionA particle moving in a straight line covers half the distance with speed of 3 m/s. The other half of the distance covered in two equal time intervals with speed of 4.5 m/s and 7.5 m/s respectively. The average speed of the particle during this motion is :-
View SolutionA particle moving in a straight line covers half the distance with speed of 3m/s. The half of the distance is covered in two equal intervals with speed of 4.5m/sand7.5m/s respectively. The average speed of the particle during this motion is :
View Solution
