The moment of inertia of a solid cylindrer about its axis of ratation is MR22. What is the value if the radius of gyration of the cylinder about this axis ?
To find the radius of gyration of a solid cylinder about its axis of rotation, we can follow these steps:
Step 1: Understand the Moment of Inertia
The moment of inertia I of a solid cylinder about its axis of rotation is given by the formula:
I=12MR2
where M is the mass of the cylinder and R is the radius of the cylinder.
Step 2: Use the Definition of Radius of Gyration
The moment of inertia can also be expressed in terms of the radius of gyration k:
I=Mk2
where k is the radius of gyration.
Step 3: Equate the Two Expressions for Moment of Inertia
Since both expressions represent the moment of inertia, we can set them equal to each other:
12MR2=Mk2
Step 4: Simplify the Equation
We can divide both sides of the equation by M (assuming M≠0):
12R2=k2
Step 5: Solve for the Radius of Gyration
To find k, we take the square root of both sides:
k=√12R2
This simplifies to:
k=R√2
Final Answer
Thus, the radius of gyration k of the solid cylinder about its axis of rotation is:
k=R√2
---
The moment of inertia I of a solid cylinder about its axis of rotation is given by the formula:
I=12MR2
where M is the mass of the cylinder and R is the radius of the cylinder.
The moment of inertia can also be expressed in terms of the radius of gyration k:
I=Mk2
where k is the radius of gyration.
Since both expressions represent the moment of inertia, we can set them equal to each other:
12MR2=Mk2
We can divide both sides of the equation by M (assuming M≠0):
12R2=k2
To find k, we take the square root of both sides:
k=√12R2
This simplifies to:
k=R√2
Thus, the radius of gyration k of the solid cylinder about its axis of rotation is:
k=R√2
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Illustration4videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)62videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))5videos
SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE21videos
TEST 1
Similar Questions
The moment of inertia of a solid cylinder about its axis of rotation is MR22. What is the value of the radius of gyration of the cylinder about this axis ?
View SolutionThe moment of inertia of a body about a given axis of rotation depends upon :-
View SolutionThe moment of inertia of a solid cylinder about its axis is given by (1/2)MR2. If this cylinder rolls without slipping the ratio of its rotational kinetic energy to its translational kinetic energy is -
View SolutionThe moment of inertia of a solid sphere about an axis passing through the centre radius is 25MR2 , then its radius of gyration about a parallel axis t a distance 2R from first axis is
View SolutionFind the moment of inertia of a solid cylinder of mass M and radius R about a line parallel to the axis of the cylinder and on the surface of the cylinder.
View SolutionCalculate the moment of inertia of the given rod about an axis CD
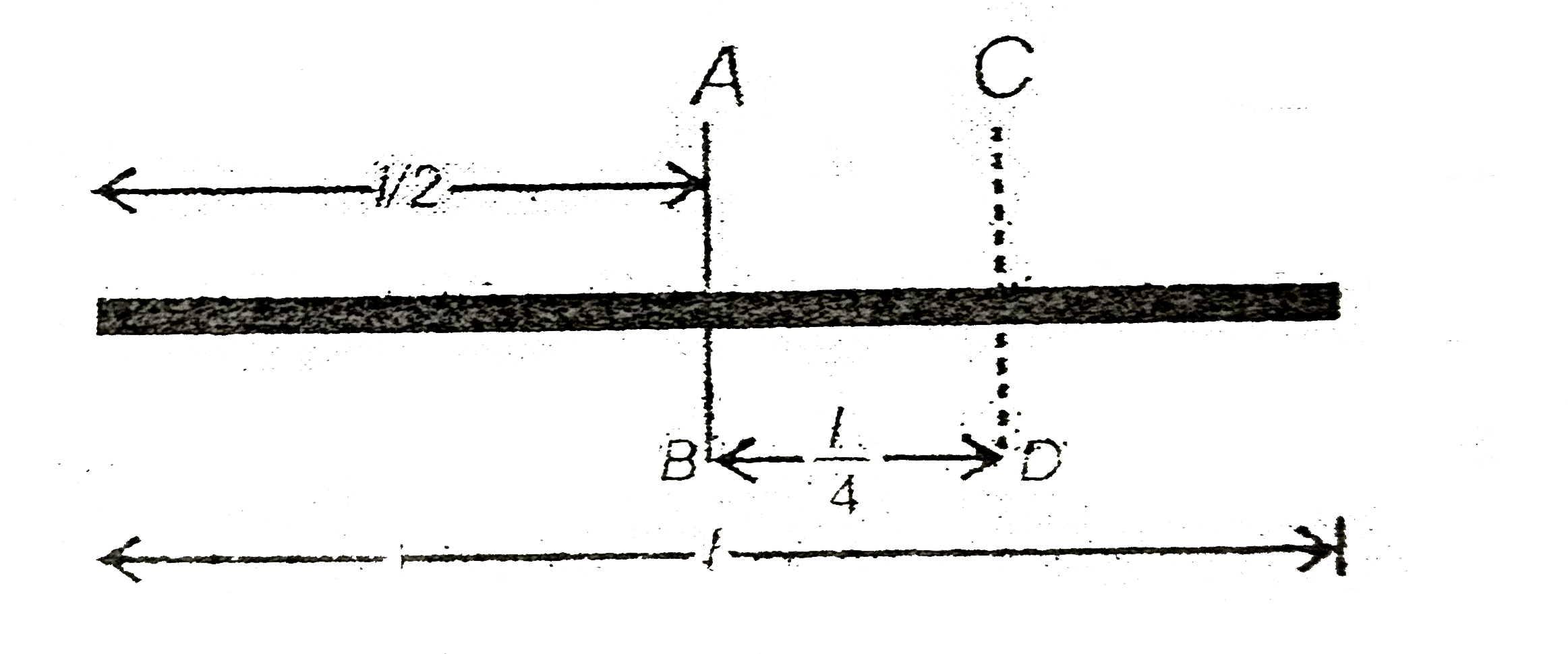 View Solution
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be
 View Solution
View SolutionCalculate the moment of inertia of the given rod about an axis CD.
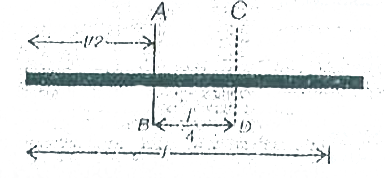 View Solution
View Solution
Find the moment of inertia of a solid sphere of mass M and radius R about an axis XX shown in figure. Also find radius of gyration about the given axis.View Solution
Find the moment of inertia of a solid sphere of mass M and radius R about an axis XX shown in figure. Also find radius of gyration about the given axis.View Solution

