In series LCR AC circuit, the voltage of the source at any instant is equal to
The sum of the maximum voltage across the elements
The voltage drop across the resistor
The sum of the instantaneous voltages across the elements
The sum of the rms voltages across the elements
The correct Answer is:C
To find the voltage of the source at any instant in a series LCR AC circuit, we can analyze the situation step by step.
1. Understand the Circuit Components:
- In a series LCR circuit, we have three main components: an inductor (L), a capacitor (C), and a resistor (R). The circuit is powered by an alternating current (AC) voltage source.
2. Identify the Instantaneous Voltages:
- The instantaneous voltages across each component can be represented as:
- Voltage across the resistor (V_R)
- Voltage across the inductor (V_L)
- Voltage across the capacitor (V_C)
3. Apply Kirchhoff's Voltage Law:
- According to Kirchhoff's Voltage Law, the sum of the instantaneous voltages across all elements in a closed loop is equal to the instantaneous voltage of the source (V_s). Therefore, we can write:
Vs=VR+VL+VC
4. Conclusion:
- From the above equation, we can conclude that the voltage of the source at any instant is equal to the sum of the instantaneous voltages across the resistor, inductor, and capacitor. Thus, the correct answer is:
Vs=VR+VL+VC
Final Answer:
The voltage of the source at any instant in a series LCR AC circuit is equal to the sum of the instantaneous voltages across the elements.
---
- In a series LCR circuit, we have three main components: an inductor (L), a capacitor (C), and a resistor (R). The circuit is powered by an alternating current (AC) voltage source.
- The instantaneous voltages across each component can be represented as:
- Voltage across the resistor (V_R)
- Voltage across the inductor (V_L)
- Voltage across the capacitor (V_C)
- According to Kirchhoff's Voltage Law, the sum of the instantaneous voltages across all elements in a closed loop is equal to the instantaneous voltage of the source (V_s). Therefore, we can write:
Vs=VR+VL+VC
- From the above equation, we can conclude that the voltage of the source at any instant is equal to the sum of the instantaneous voltages across the resistor, inductor, and capacitor. Thus, the correct answer is:
Vs=VR+VL+VC
The voltage of the source at any instant in a series LCR AC circuit is equal to the sum of the instantaneous voltages across the elements.
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - B) (Objective Type Questions (One option is correct))
14 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - C ) (Objective Type Questions) ( More than one option are correct)
2 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Try Yourself
15 Videos
- ATOMSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION J (Aakash Challengers )
5 Videos
Similar Questions
Knowledge Check
In a series LCR circuit, the voltage across the resistance, capacitance and inductance is 10 V each. If the capacitance is short circuited, the voltage across the inductance will be
A10VB10√2VC10√2VD20VIn a series LCR circuit the voltage across an inductor, capacitor and resistor are 20 V, 20 V and 40 V respectively. The phase difference between the applied voltage and the current in the circuit is
A30∘B45∘C60∘D0∘
In a series LCR circuit the rms voltage across the inductance , capacitance and resistance are respectively 4 V, 8 V and 5 V . The RMS voltage of the AC source in the circuit is
View SolutionIn series LCR circuit voltage leads the current. When
(Given that ω0 = resonant angular frequency)
View SolutionIn series LCR circuit, the voltages across R, L and C are shown in the figure. The voltage of applied source
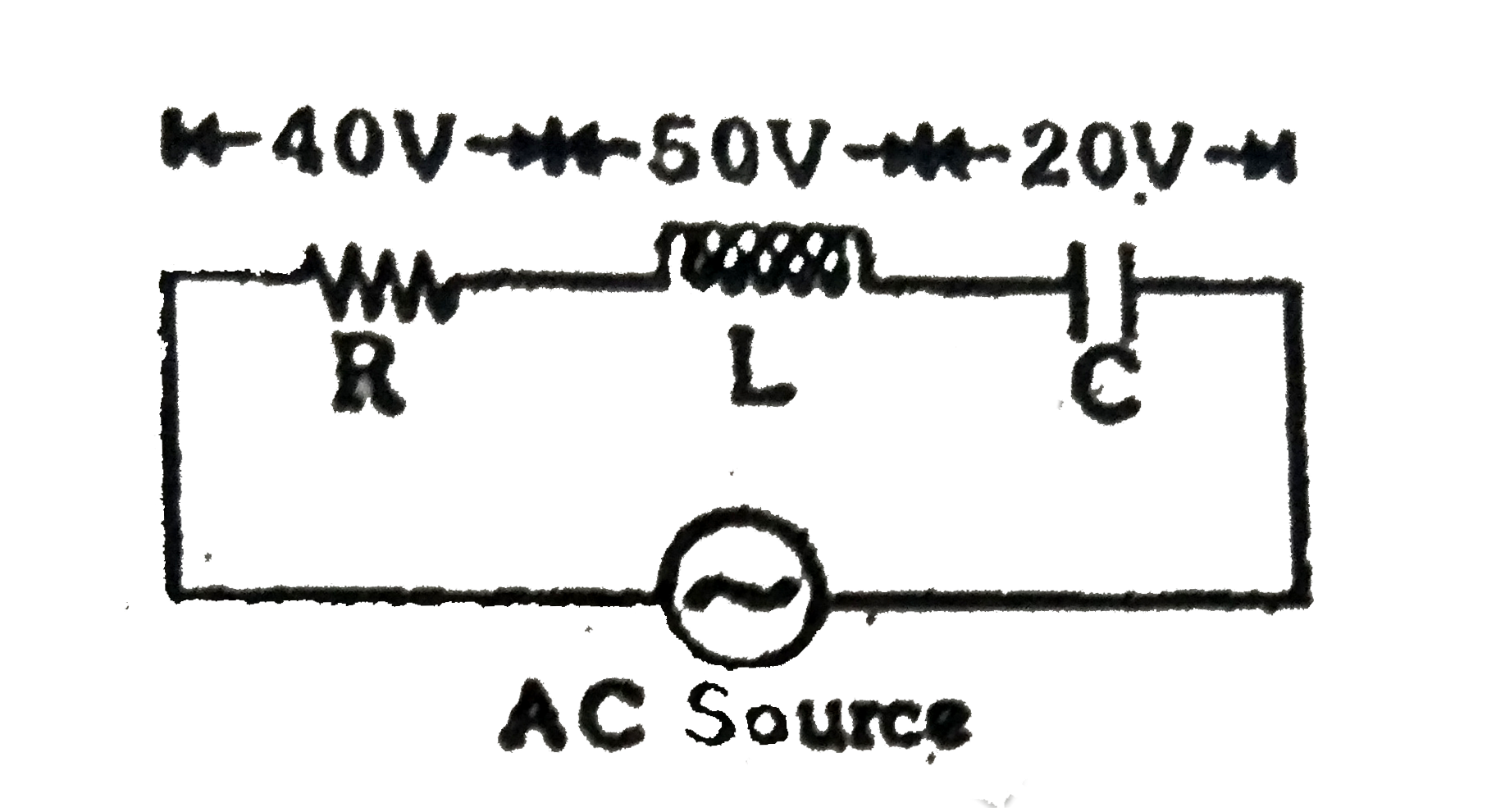
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionAn ac source of 50 V (r.m.s value) is connected across a series R - C circuit. If the r.m.s voltage across the resistor is 40 V, then the r.m.s voltage across the capacitor is
View Solution
