Question

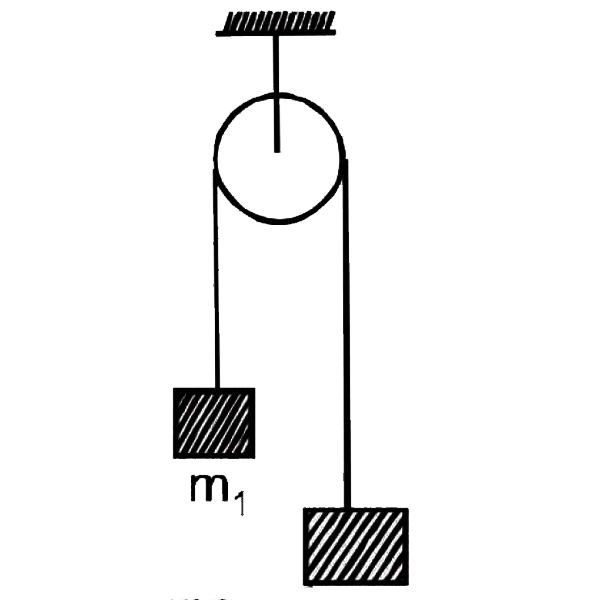
Two blocks of masses 2 kg and 4 kg tied to a string passing over a frictionless pulley. What will be the magnitude of acceleration of the masses, when they are allowed to move freely?

Explanation

To solve the problem of two blocks of masses 2 kg and 4 kg tied to a string passing over a frictionless pulley, we will follow these steps:

1. Identify the masses and their weights:
- Mass \( m_1 = 2 \, \text{kg} \)
- Mass \( m_2 = 4 \, \text{kg} \)
- Weight of \( m_1 \) (2 kg): \( W_1 = m_1 \cdot g = 2 \cdot 9.8 = 19.6 \, \text{N} \)
- Weight of \( m_2 \) (4 kg): \( W_2 = m_2 \cdot g = 4 \cdot 9.8 = 39.2 \, \text{N} \)

2. Determine the direction of motion:
- Since \( m_2 \) (4 kg) is heavier than \( m_1 \) (2 kg), \( m_2 \) will move downward and \( m_1 \) will move upward.

3. Set up the equations of motion:
- For the 4 kg mass (moving downward):
\( W_2 - T = m_2 \cdot a \quad \text{(1)} \)
- For the 2 kg mass (moving upward):
\( T - W_1 = m_1 \cdot a \quad \text{(2)} \)

4. Substitute the weights into the equations:
- From equation (1):
\( 39.2 - T = 4a \quad \text{(3)} \)
- From equation (2):
\( T - 19.6 = 2a \quad \text{(4)} \)

5. Solve for tension \( T \) from equation (4):
\( T = 2a + 19.6 \quad \text{(5)} \)

6. Substitute equation (5) into equation (3):
\( 39.2 - (2a + 19.6) = 4a \)
\( 39.2 - 19.6 - 2a = 4a \)
\( 19.6 = 6a \)

7. Solve for acceleration \( a \):
\( a = \frac{19.6}{6} \approx 3.27 \, \text{m/s}^2 \)

8. Final answer:
The magnitude of the acceleration of the masses when they are allowed to move freely is approximately \( 3.27 \, \text{m/s}^2 \).