The limiting value of static friction between two contact surfaces is
Proportional to normal force between the surface in contact
Independent of apparent area of contact
Depends on the microscopic area of contact
All of these
To find the limiting value of static friction between two contact surfaces, we can follow these steps:
1. Understanding Static Friction:
- Static friction is the force that prevents two surfaces from sliding past each other. It acts in response to an applied force and can vary in magnitude up to a maximum value.
2. Identifying Forces:
- Consider a block of mass M resting on a surface. The weight of the block, Mg, acts downward due to gravity, where g is the acceleration due to gravity.
- The normal force N acts upward, balancing the weight of the block.
3. Limiting Friction:
- The maximum static frictional force, known as limiting friction, is reached just before the block begins to slide. It can be expressed mathematically as:
Flimiting=μs⋅N
- Here, μs is the coefficient of static friction, and N is the normal force.
4. Normal Force:
- For a horizontal surface, the normal force N is equal to the weight of the block:
N=Mg
- Therefore, the limiting friction can be rewritten as:
Flimiting=μs⋅Mg
5. Dependence on Surface Properties:
- The coefficient of static friction μs depends on the nature of the surfaces in contact (e.g., roughness). A rough surface will have a higher coefficient than a smooth surface.
6. Independence from Area of Contact:
- It is important to note that the limiting friction does not depend on the apparent area of contact between the two surfaces. This means that regardless of how much area is in contact, the maximum static friction is determined by the normal force and the coefficient of friction.
7. Conclusion:
- The limiting value of static friction is thus given by the equation:
Flimiting=μs⋅Mg
- This indicates that the limiting friction is proportional to the normal force and influenced by the surface characteristics.
Final Answer
The limiting value of static friction between two contact surfaces is given by:
Flimiting=μs⋅N
where N=Mg.
---
- Static friction is the force that prevents two surfaces from sliding past each other. It acts in response to an applied force and can vary in magnitude up to a maximum value.
- Consider a block of mass M resting on a surface. The weight of the block, Mg, acts downward due to gravity, where g is the acceleration due to gravity.
- The normal force N acts upward, balancing the weight of the block.
- The maximum static frictional force, known as limiting friction, is reached just before the block begins to slide. It can be expressed mathematically as:
Flimiting=μs⋅N
- Here, μs is the coefficient of static friction, and N is the normal force.
- For a horizontal surface, the normal force N is equal to the weight of the block:
N=Mg
- Therefore, the limiting friction can be rewritten as:
Flimiting=μs⋅Mg
- The coefficient of static friction μs depends on the nature of the surfaces in contact (e.g., roughness). A rough surface will have a higher coefficient than a smooth surface.
- It is important to note that the limiting friction does not depend on the apparent area of contact between the two surfaces. This means that regardless of how much area is in contact, the maximum static friction is determined by the normal force and the coefficient of friction.
- The limiting value of static friction is thus given by the equation:
Flimiting=μs⋅Mg
- This indicates that the limiting friction is proportional to the normal force and influenced by the surface characteristics.
The limiting value of static friction between two contact surfaces is given by:
Flimiting=μs⋅N
where N=Mg.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-A)
65 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-B)
44 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Example
24 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
Knowledge Check
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
The coefficient of static friction between two surfaces depend on
View SolutionThe limiting friction between two surfaces depends
View SolutionTwo uniform boards, tied together with the help of a string, are balanced on a surface as shown in Fig.
The coefficient of static friction between boards and surface is 0.5. The minimum value of θ, for which this type of arrangement is possible is

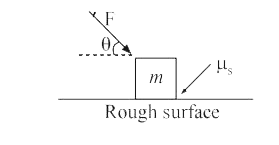
View SolutionWhat is the value of applied force ‘F’ which is exerted at an angle θ with the horizontal on a block of mass ‘m’ placed on a horizontal rough surface, if the coefficient of static friction between the block and the surface is , such that the block is on the verge of moving horizontally?
View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionThe coefficient of static friction may be
View SolutionFind the accelerations of rod A and wedge B in the arrangement shown in figure if the ratio of the mass of the wedge to that of the rod equals η, and the friction between all contact surfaces is negligible.

View Solution
