In perfectly elastic collision between two masses m1andm2 in one dimension energy transfer is a maximum, when
m1=2m2
m1<<m2
m1>>m2
m1=m2
The correct Answer is:D
To solve the problem regarding the maximum energy transfer in a perfectly elastic collision between two masses m1 and m2 in one dimension, we can follow these steps:
Step 1: Understand the Concept of Elastic Collision
In a perfectly elastic collision, both momentum and kinetic energy are conserved. This means that the total momentum before the collision is equal to the total momentum after the collision, and the same applies to kinetic energy.
Step 2: Write Down the Conservation Equations
For two masses m1 and m2 colliding elastically, the conservation of momentum and kinetic energy can be expressed as:
1. Conservation of Momentum:
m1u1+m2u2=m1v1+m2v2
where u1 and u2 are the initial velocities, and v1 and v2 are the final velocities of masses m1 and m2 respectively.
2. Conservation of Kinetic Energy:
12m1u21+12m2u22=12m1v21+12m2v22
Step 3: Analyze Energy Transfer
The energy transfer during the collision can be analyzed by looking at the change in kinetic energy of the masses. The maximum energy transfer occurs when one mass transfers its kinetic energy to the other mass effectively.
Step 4: Determine Conditions for Maximum Energy Transfer
To find the condition for maximum energy transfer, we can analyze the case when the two masses are equal:
- If m1=m2, then the velocities after the collision can be derived from the conservation equations.
Step 5: Conclusion
The energy transfer is maximum when the masses are equal, i.e., m1=m2. This is because, in this scenario, the velocities after the collision will be such that the kinetic energy is transferred most efficiently from one mass to the other.
Final Answer
The energy transfer is maximum when m1=m2.
---
In a perfectly elastic collision, both momentum and kinetic energy are conserved. This means that the total momentum before the collision is equal to the total momentum after the collision, and the same applies to kinetic energy.
For two masses m1 and m2 colliding elastically, the conservation of momentum and kinetic energy can be expressed as:
1. Conservation of Momentum:
m1u1+m2u2=m1v1+m2v2
where u1 and u2 are the initial velocities, and v1 and v2 are the final velocities of masses m1 and m2 respectively.
12m1u21+12m2u22=12m1v21+12m2v22
The energy transfer during the collision can be analyzed by looking at the change in kinetic energy of the masses. The maximum energy transfer occurs when one mass transfers its kinetic energy to the other mass effectively.
To find the condition for maximum energy transfer, we can analyze the case when the two masses are equal:
- If m1=m2, then the velocities after the collision can be derived from the conservation equations.
The energy transfer is maximum when the masses are equal, i.e., m1=m2. This is because, in this scenario, the velocities after the collision will be such that the kinetic energy is transferred most efficiently from one mass to the other.
The energy transfer is maximum when m1=m2.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - A)
64 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
35 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-J (AAKASH CHALLENGERS QUESTIONS)
14 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
In an elastic collision between two particles
View SolutionShow that in a head on elastic collision between two particles, the transference of energy is maximum when their mass ratio is unity.
View SolutionConsider objects of masses m1andm2 moving initially along the same straight line with velocities u1andu2 respectively. Considering a perfectly elastic collision (with u1>u2) derive expressions for their velocities after collision.
View SolutionFrom study of elastic collision we understand that if two colliding particles have equal masses they interchange their velocities and energy transfer is maximum if they have equal masses and one is at rest w... other. This principle can be easily used in nuclear reactor to select a moderator. If m1=m2=m and m2 at rest then m1 will stop after colliding with m2 and m2 will move with velocity of m1. In case of nuclear reactor we used a moderator such that neutrons can be slowed down. With above reference answer the following questions
Which is correct statement
View SolutionConsider an elastic collision between a neutron and a light nuclei like Beryllium and colculate neuton's energy transferred to Beryllium,
View SolutionA bullet of mass m hits a block of mass M. The transfer of energy is maximum when
View SolutionA bullet of mass m hits a block of mass M. The transfer of energy is maximum when
View SolutionA stationary body explodes into two fragments of masses m1 and m2. If momentum of one fragment is p, the energy of explosion is
View SolutionA stationary body explodes into two fragments of masses m1 and m2. If momentum of one fragment is p, the energy of explosion is
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
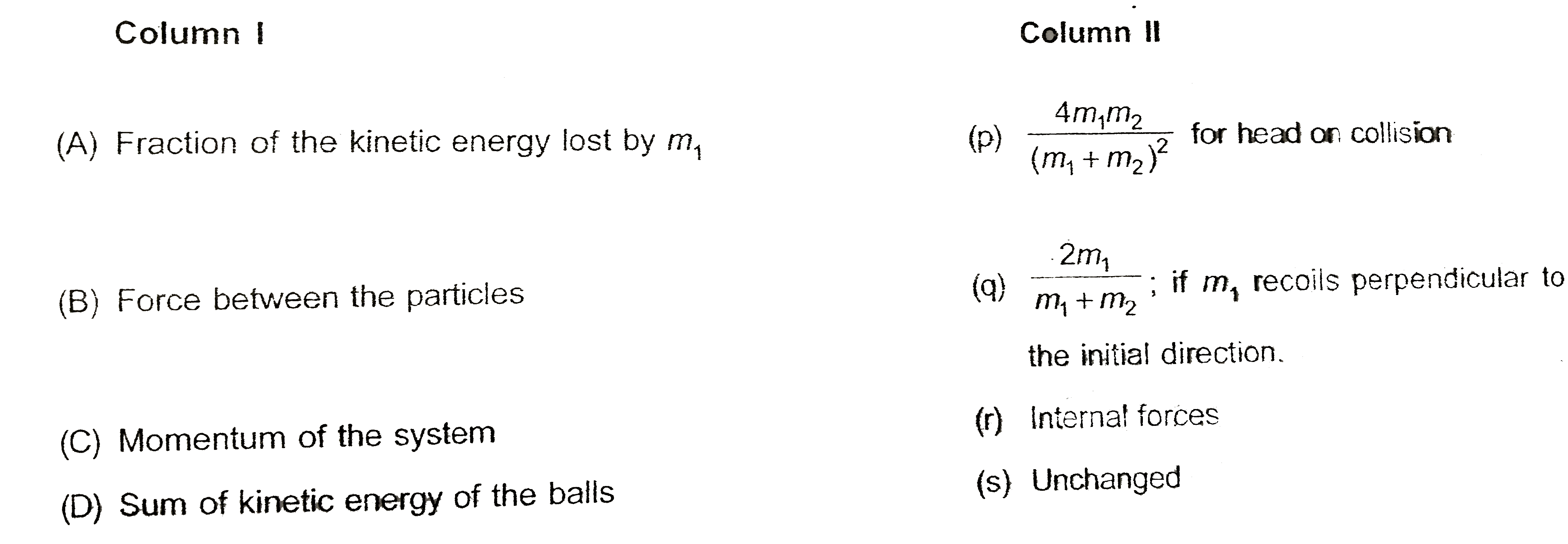
View Solution
