1000 small water drops each of capacitance C join togethter to form one large spherical drop. The capacitance of bigge sphere is
C
10 C
100 C
1000 c
The correct Answer is:B
To solve the problem of finding the capacitance of a larger spherical drop formed by combining 1000 small water drops, we can follow these steps:
Step 1: Understand the Capacitance of a Sphere
The capacitance C of a small spherical drop of radius r is given by the formula:
C=4πϵ0r
where ϵ0 is the permittivity of free space.
Step 2: Volume Conservation
When 1000 small drops combine to form one larger drop, the volume of the larger drop must equal the total volume of the small drops. The volume V of a sphere is given by:
V=43πr3
Thus, the total volume of 1000 small drops is:
Vsmall=1000×43πr3
The volume of the larger drop of radius R is:
Vlarge=43πR3
Setting these volumes equal gives us:
1000×43πr3=43πR3
Step 3: Simplifying the Volume Equation
We can cancel 43π from both sides:
1000r3=R3
Taking the cube root of both sides, we find:
R=10r
Step 4: Calculate the Capacitance of the Larger Sphere
Now, we can find the capacitance C′ of the larger sphere using its radius R:
C′=4πϵ0R
Substituting R=10r:
C′=4πϵ0(10r)=10×(4πϵ0r)
Since C=4πϵ0r, we can substitute:
C′=10C
Conclusion
The capacitance of the larger spherical drop is:
C′=10C
Final Answer
Thus, the capacitance of the larger sphere is 10C.
---
The capacitance C of a small spherical drop of radius r is given by the formula:
C=4πϵ0r
where ϵ0 is the permittivity of free space.
When 1000 small drops combine to form one larger drop, the volume of the larger drop must equal the total volume of the small drops. The volume V of a sphere is given by:
V=43πr3
Thus, the total volume of 1000 small drops is:
Vsmall=1000×43πr3
The volume of the larger drop of radius R is:
Vlarge=43πR3
Setting these volumes equal gives us:
1000×43πr3=43πR3
We can cancel 43π from both sides:
1000r3=R3
Taking the cube root of both sides, we find:
R=10r
Now, we can find the capacitance C′ of the larger sphere using its radius R:
C′=4πϵ0R
Substituting R=10r:
C′=4πϵ0(10r)=10×(4πϵ0r)
Since C=4πϵ0r, we can substitute:
C′=10C
The capacitance of the larger spherical drop is:
C′=10C
Thus, the capacitance of the larger sphere is 10C.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:AAKASH INSTITUTE ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:ASSIGNMENT SECTION - B
Explore 38 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:AAKASH INSTITUTE ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:ASSIGNMENT SECTION - C
Explore 53 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:AAKASH INSTITUTE ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:EXERCISE
Explore 20 Videos
- ELECTROMAGNETIC WAVESBook:AAKASH INSTITUTE ENGLISHChapter:ELECTROMAGNETIC WAVESExercise:ASSIGNMENT SECTION - D Assertion-Reason Type Questions
Explore 25 Videos
- GRAVITATION Book:AAKASH INSTITUTE ENGLISHChapter:GRAVITATION Exercise:ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
Explore 15 Videos
Similar Questions
1000 small water drops each of radius r and charge q coalesce together to form one spherical drop. The potential of the bigger drop is larger than that of the smaller one by a factor
View SolutionSixty four identical sphere of change q and capacitance C each are combined to form a large sphere . The charge and capacitance of the large sphere is
View SolutionCalculate the energy released when 1000 small water drops each of same radius 10−7m coalesce to form one large drop. The surface tension of water is 7.0×10−2N/m.
View SolutionA spherical drop of capacitance 1μF is broken into eight drop of equal radius. Then, the capacitance of each small drop is
View SolutionThree capacitors each of capacitance C and of breakdown voltage V are joined in series. The capacitance and breakdown voltage of the combination will be
View SolutionA network of six identical capacitors, each of capacitance C is formed as shown below. The equivalent capacitance between the point A and B is
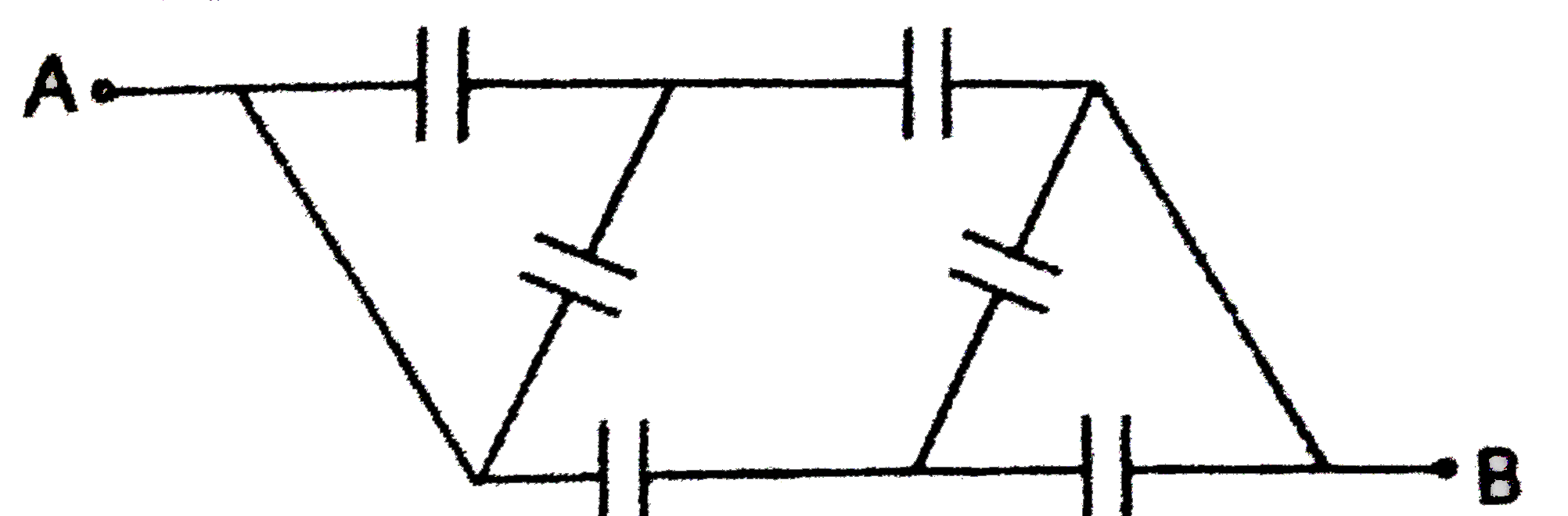
View SolutionA spherical drop of capacitance 12μF is broken into eight drops of equal radius. What is the capacitance of each small drop in μF?
View SolutionIf n drops, each of capacitance C and charged to a potential V, coalesce to form a big drop, the ratio of the energy stored in the big drop to that in each small drop will be
View SolutionA large number of liquid drops each of radius 'a' coalesce to form a single spherical drop of radius b. The energy released in the process is converted into kinetic energy of the big drops formed. The speed of big drop will be
View SolutionA parallel plate capacitor of capacitance C is connected to a battery and is charged to a potential difference V. Another capacitor of capacitance 2C is ismilarly charged to a potential difference 2V. The charging battery is now disconnected and the capacitors are connected in parallel to each other in such a way that the poistive terminal of one is connected to the negative terminal of the other. The final energy of the configuration is
View Solution