If a current carrying loo experiences a force = (where is positive constant) then nature of magnetic field should be
Uniform parallel to xy-plane
Uniform along positive z-direction
Uniform along negative z-direction
Radially outward and symmetric
To determine the nature of the magnetic field when a current-carrying loop experiences a force ¯F=F0^k, we can analyze the situation using the relationship between the magnetic force, current, and magnetic field.
1. Understanding the Force on a Current-Carrying Loop:
The force ¯F acting on a current-carrying conductor in a magnetic field is given by the equation:
¯F=I(¯LׯB)
where I is the current, ¯L is the length vector of the conductor, and ¯B is the magnetic field vector.
2. Analyzing the Given Force:
The force is given as:
¯F=F0^k
This indicates that the force is directed along the positive z-axis.
3. Determining the Direction of the Magnetic Field:
Since the force is perpendicular to both the current direction and the magnetic field, we need to find a magnetic field ¯B such that:
¯F⋅¯B=0
This means that ¯B cannot have a component in the z-direction (i.e., it cannot be parallel to the z-axis).
4. Evaluating the Options:
- Option 1: Magnetic field is parallel to the XY plane (e.g., ¯B=λ1^i+λ2^j).
- Here, ¯F⋅¯B=F0^k⋅(λ1^i+λ2^j)=0. This is valid.
- Option 2: Magnetic field is uniform along the positive Z direction (e.g., ¯B=λ1^k).
- Here, ¯F⋅¯B=F0^k⋅λ1^k≠0. This is invalid.
- Option 3: Magnetic field is uniform along the negative Z direction (e.g., ¯B=λ1(−^k)).
- Here, ¯F⋅¯B=F0^k⋅λ1(−^k)≠0. This is invalid.
- Option 4: Magnetic field is radially outward and symmetric (e.g., ¯B=λ1^i+λ2^j+λ3^k).
- Here, ¯F⋅¯B≠0 because it has a component in the z-direction. This is invalid.
5. Conclusion:
The only valid option is Option 1, where the magnetic field is parallel to the XY plane. Therefore, the nature of the magnetic field should be such that it is perpendicular to the force acting on the current-carrying loop.
Final Answer:
The nature of the magnetic field should be parallel to the XY plane.
The force ¯F acting on a current-carrying conductor in a magnetic field is given by the equation:
¯F=I(¯LׯB)
where I is the current, ¯L is the length vector of the conductor, and ¯B is the magnetic field vector.
The force is given as:
¯F=F0^k
This indicates that the force is directed along the positive z-axis.
Since the force is perpendicular to both the current direction and the magnetic field, we need to find a magnetic field ¯B such that:
¯F⋅¯B=0
This means that ¯B cannot have a component in the z-direction (i.e., it cannot be parallel to the z-axis).
- Option 1: Magnetic field is parallel to the XY plane (e.g., ¯B=λ1^i+λ2^j).
- Here, ¯F⋅¯B=F0^k⋅(λ1^i+λ2^j)=0. This is valid.
- Option 2: Magnetic field is uniform along the positive Z direction (e.g., ¯B=λ1^k).
- Here, ¯F⋅¯B=F0^k⋅λ1^k≠0. This is invalid.
- Option 3: Magnetic field is uniform along the negative Z direction (e.g., ¯B=λ1(−^k)).
- Here, ¯F⋅¯B=F0^k⋅λ1(−^k)≠0. This is invalid.
- Option 4: Magnetic field is radially outward and symmetric (e.g., ¯B=λ1^i+λ2^j+λ3^k).
- Here, ¯F⋅¯B≠0 because it has a component in the z-direction. This is invalid.
The only valid option is Option 1, where the magnetic field is parallel to the XY plane. Therefore, the nature of the magnetic field should be such that it is perpendicular to the force acting on the current-carrying loop.
The nature of the magnetic field should be parallel to the XY plane.
Topper's Solved these Questions
Similar Questions
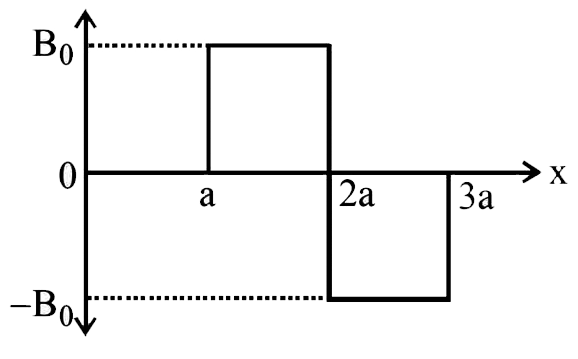
A magnetic field →B=B0ˆj , exists in the region a<x<2a , and →B=−B0ˆj , in the region 2a<x<3a, where B0 is a positive constant . A positive point charge moving with a velocity →v=v0ˆi , where v0 is a positive constant , enters the magnetic field at x=a . The trajectory of the charge in this region can be like
View SolutionA current carrying conductor placed in a magnetic field experiences maximum force when angle between current and magnetic field is :
View SolutionA particle of charge q>0 is moving at speed v in the +z direction through a region of uniform magnetic field. The magnetic force on the particle →F=F0(3ˆi+4ˆj), where F0 is a positive constant.
(a) Determine the components Bx,By and Bz or at least as many of the three components as is possible from the information given.
(b) If it is given in addition that the magnetic field has magnitude 6F0qv , determine the magnitude of Bz.
View SolutionA particle moving along the x axis is acted upon by a single force F = F0e−kx , where F0 and k are constants. The particle is released from rest at x = 0. It will attain a maximum kinetic energy of :
View SolutionA particle is placed at the origin and a force F=Kx is acting on it (where k is a positive constant). If U(0)=0, the graph of U(x) verses x will be (where U is the potential energy function.)
View SolutionA particle is placed at the origin and a force F=Kx is acting on it (where k is a positive constant). If U(0)=0, the graph of U(x) verses x will be (where U is the potential energy function.)
View SolutionWhen a current carrying conductor is placed in a magnetic field, it experiences a mechanical force. What should be the angle between the magnetic field and the length of the conductor so that the force experienced is :
(i) Zero
(ii) Maximum ?
View SolutionAn electron accelerated through a potential difference V passes through a uniform tranverses magnetic field and experiences a force F. If the accelerating potential is increased to 2V, the electron in the same magnetic field will experience a force:
View SolutionThe potential energy U for a force field →F is such that U=−kxy where K is a constant . Then
View SolutionThe force acting on a particle moving along X-axis is F=−k(x−v0t) where k is a positive constant. An observer moving at a constant velocity v0 along the X-axis looks the particle . What kind of motion does he find for the particle?
View Solution
