A plane electromagnetic wave of Intensity 6Wm2 strikes a small mirror of area 20cm2, hel perpendicular to the incident wave. The radiation force on the mirror will be (Assume that mirror is perfectly reflecting)
8⋅10−11N
4⋅10−11N
10−11N
2⋅10−12N
To solve the problem of finding the radiation force on a small mirror when a plane electromagnetic wave strikes it, we can follow these steps:
1. Identify Given Values:
- Intensity of the electromagnetic wave, I=6W/m2
- Area of the mirror, A=20cm2=20×10−4m2=2×10−3m2
2. Calculate the Energy Incident on the Mirror:
- The energy E incident on the mirror can be calculated using the formula:
E=I×A
- Substituting the values:
E=6W/m2×2×10−3m2=12×10−3W=0.012W
3. Relate Energy to Momentum:
- The momentum P of the electromagnetic wave can be related to energy using the formula:
P=Ec
- Where c is the speed of light, c≈3×108m/s.
- Substituting the energy calculated:
P=0.012W3×108m/s=4×10−11kg m/s
4. Calculate Change in Momentum:
- When the wave reflects off the mirror, the change in momentum ΔP is given by:
ΔP=2P
- Therefore:
ΔP=2×4×10−11kg m/s=8×10−11kg m/s
5. Calculate the Radiation Force:
- The radiation force F can be calculated as:
F=ΔPΔt
- Assuming Δt=1s:
F=8×10−11N
Final Answer:
The radiation force on the mirror is 8×10−11N.
---
- Intensity of the electromagnetic wave, I=6W/m2
- Area of the mirror, A=20cm2=20×10−4m2=2×10−3m2
- The energy E incident on the mirror can be calculated using the formula:
E=I×A
- Substituting the values:
E=6W/m2×2×10−3m2=12×10−3W=0.012W
- The momentum P of the electromagnetic wave can be related to energy using the formula:
P=Ec
- Where c is the speed of light, c≈3×108m/s.
- Substituting the energy calculated:
P=0.012W3×108m/s=4×10−11kg m/s
- When the wave reflects off the mirror, the change in momentum ΔP is given by:
ΔP=2P
- Therefore:
ΔP=2×4×10−11kg m/s=8×10−11kg m/s
- The radiation force F can be calculated as:
F=ΔPΔt
- Assuming Δt=1s:
F=8×10−11N
The radiation force on the mirror is 8×10−11N.
Topper's Solved these Questions
Similar Questions
A plane electromagnetic wave of intensity of 10W/m2 strikes a small mmirror of area 20cm2, held perpendicular to the approcaching wave. The radiation froce on the mirror will be:
View SolutionA plane electromagnetic wave of wave intensity 6W/m2 strikes a small mirror of area 40cm(2), held perpendicular to the approaching wave. The momentum transferred by the wave to the mirror each second will be
View SolutionRadiation of intensity 1W/m2 are striking on a metal plate. The pressure on the plate is
View Solution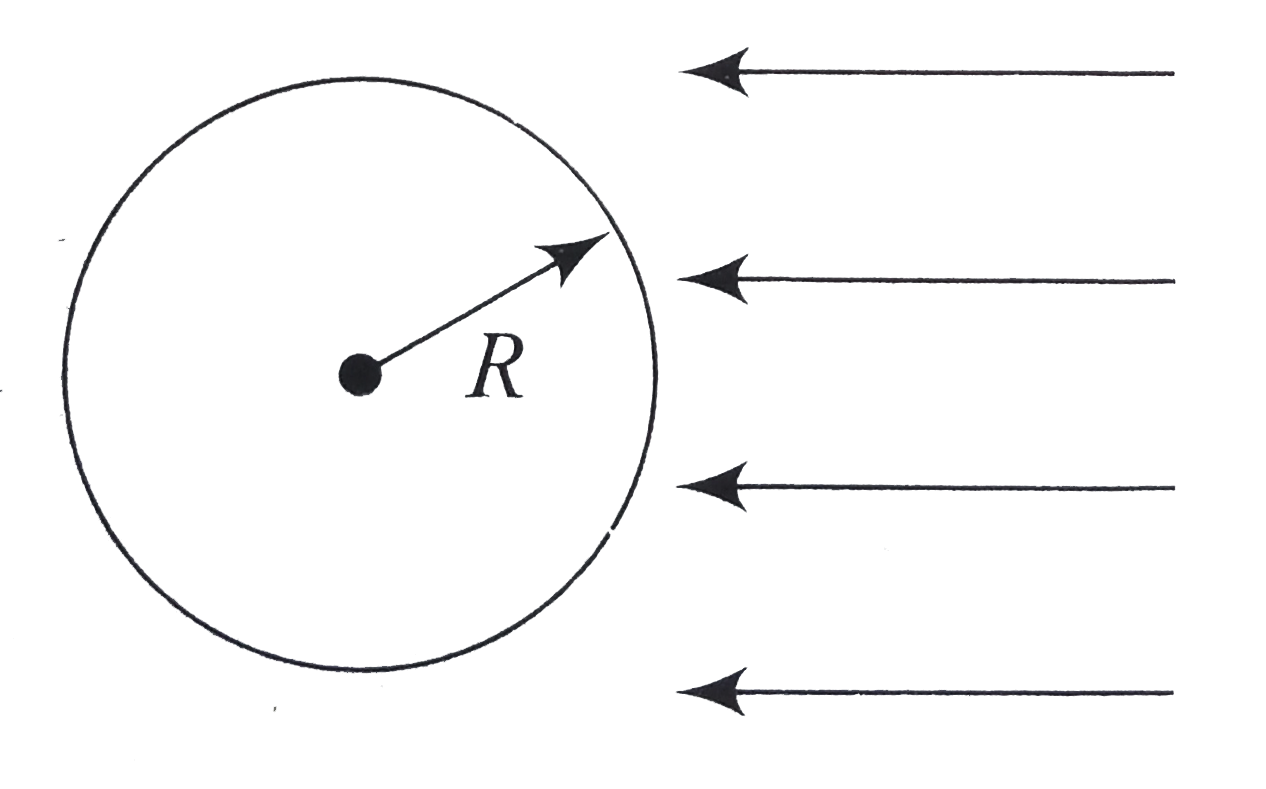
A plane wave of intensity I=0.70Wcm−2 illuminates a sphere with ideal mirror surface. The radius of sphere is R=5.0cm. From the standpoint of photon theory, find the force that light exerts on the sphere.
View SolutionSpherical wave fronts shown in figure,strike a plane mirror .reflected

View SolutionThe pressure exerted by an electromagnetic wave of intensity I(Wm−2) on a non-reflecting surface is ( c is the velocity of light)
View SolutionA cylinderical glass rod of radius 0.1m and refractive index √3 lies on a horizontal plane mirror. A horizontal ray of light moving perpendicular to the axis of the rod is incident on it. At what height from the mirror should the ray be incident so that it leaves the rod at a height of 0.1m above the plane mirror? At what centre to centre distance a second similar rod, parallel to the first, be placed on the mirror, such that the emergent ray from the second rod is in line with the incident ray on the first rod?
View SolutionA plane electromagnetic wave is incident on a plane surface of area A, normally and is perfectly reflected. If energy E strikes the surface in time t then average pressure exerted on the surface is (c = speed of light)
View SolutionA plane light wave of intensity I=0.20Wcm−2 falls on a plane mirror surface with reflection coefficient ρ=0.8. The angle of incidence is 45∘. In terms of corpuscular theory, find the magnitude of the normal pressure exerted on that surface.
View SolutionA point source of light is 60 cm from a screen and is kept at the focus of a concave mirror which reflects light on the screen. The focal length of the mirror is 20 cm. The ratio of average intensities of the illumination on the screen when the mirror is present and when the mirror is removed is:
View Solution