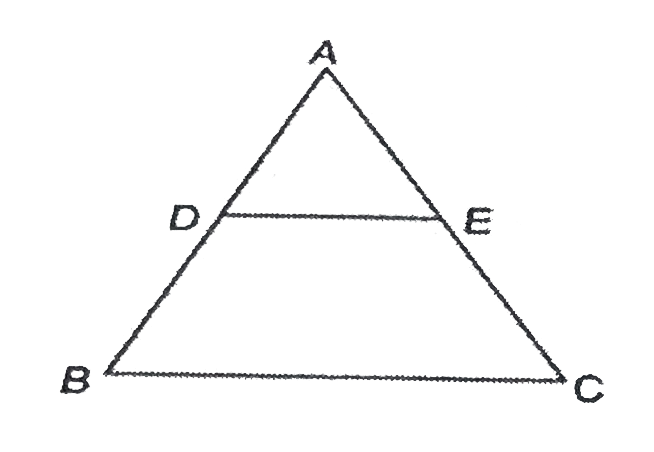
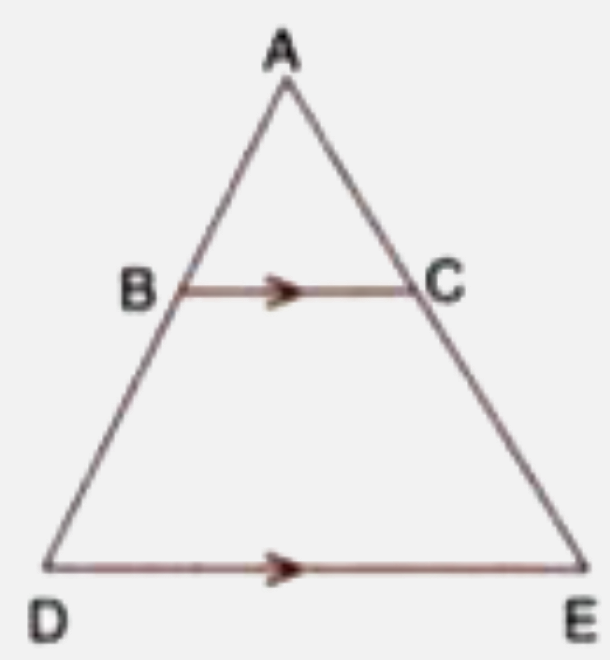
In the given figure, DE is parallel to the base BC of triangle ABC and AD: DB = 5: 3. Find the ratio :
and then
The correct Answer is:
Step by step video, text & image solution for In the given figure, DE is parallel to the base BC of triangle ABC and AD: DB = 5: 3. Find the ratio : (AD)/(AB) and then (DE)/(BC) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(A)48videos
SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)
- BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)17videos
SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)
- BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)10videos
SIMILARITY
- BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )18videos
SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)
Similar Questions
In the given figure, DE is parallel to the base BC of triangle ABC and AD: DB = 5: 4. Find the ratio :

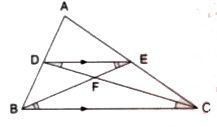
Area of ΔDEFArea ofΔBFCView SolutionIn the given figure, ABC is a triangle. DE is parallel to BC and ADDB=32
Determine the ratios and ADABView SolutionIn the given figure if DE||BC, find the value of x.
View SolutionIn the given figure, ABC is a triangle. DE is parallel to BC and ADDB=32

What is the ratio of the areas of ΔDEF and ΔBFC ?View SolutionIn the given figure, AC = AE, AB = AD and ∠BAD=∠EAC. Show that : BC = DE.
 View Solution
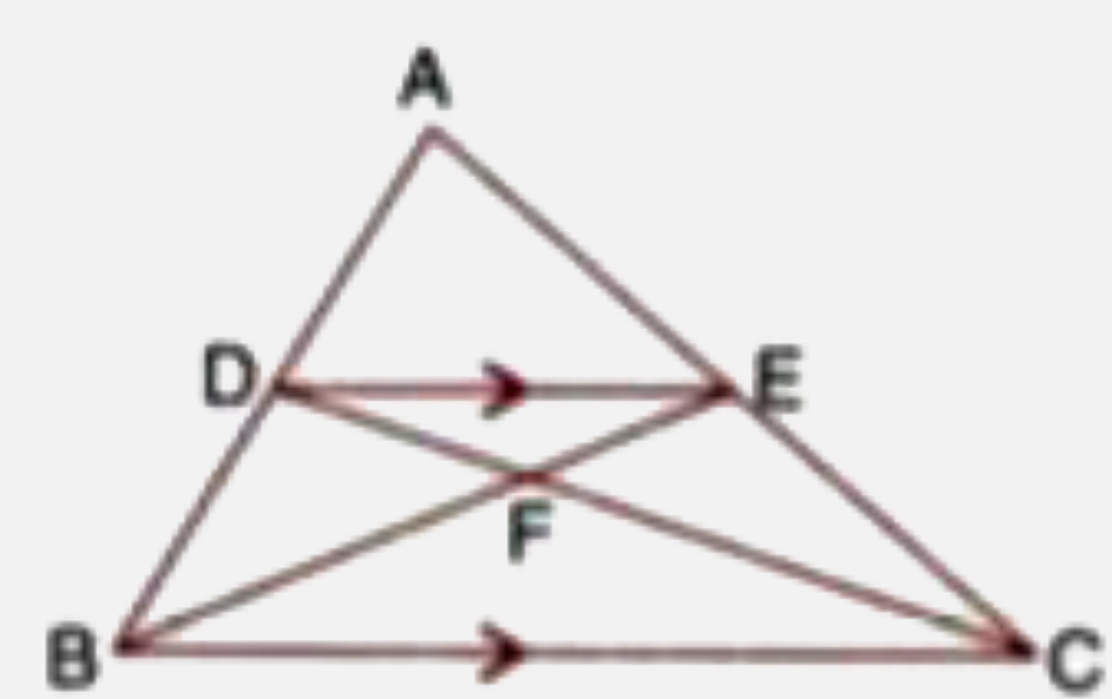
View SolutionIn the given figure DE∣∣BCandAD:DB=5:4
Find the ratio ar(ΔDEF):ar(ΔCFB)
 View Solution
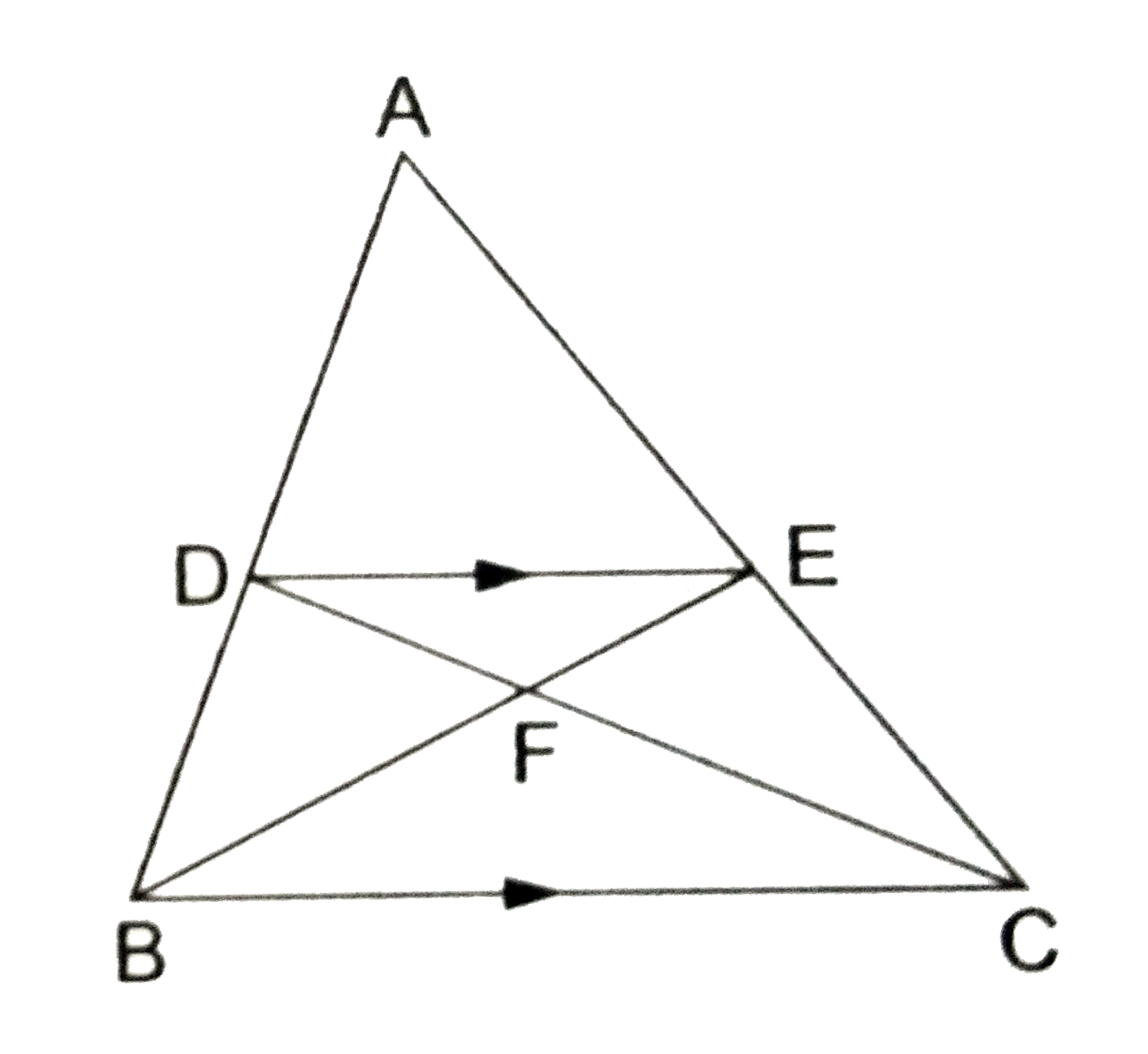
View SolutionIn the figure DE is parallel to BC and ADDB=23 if AE = 3.7 cm find EC.
 View Solution
View SolutionIn the given figure, DE // BC.

Prove that ΔADEandΔABC are similarView SolutionIn figure, DE||BC and the ratio of the areas of △ADEand trapezium BDEC is 4:5 . Find the ratio of DE:BC . If BD = 2cm. Then find AD.
View SolutionIn the given figure, BC is parallel to DE. Area of triangle ABC=25cm2, Area of trapezium BCED=24cm2andDE=14cm. Calculate the length of BC.
Also, find the area of triangle BCD.
 View Solution
View Solution

