The acceleration of a particle travelling along a straight line is a=kv, where k is constant (k>0). If at t=0,V=V0 then speed of particle at t=V202.
The correct Answer is:C
To solve the problem, we start with the given acceleration of a particle traveling along a straight line, which is defined as:
a=kv
where k is a constant and v is the velocity of the particle. We know that acceleration can also be expressed in terms of velocity as:
a=dvdt
Thus, we can equate the two expressions for acceleration:
dvdt=kv
Now, we can rearrange this equation to separate the variables v and t:
vdv=kdt
Next, we will integrate both sides. The left side will be integrated with respect to v and the right side with respect to t:
∫vdv=∫kdt
The integral of vdv is:
v22+C1
And the integral of kdt is:
kt+C2
Setting the constants of integration equal to zero for simplicity, we have:
v22=kt
Now, we need to apply the limits for the integration. At t=0, the velocity v=V0. So we can rewrite our equation with limits:
v22−V202=kt
At t=V202:
v22−V202=k(V202)
Now, simplifying this equation:
v22−V202=kV202
Multiplying through by 2 to eliminate the fraction:
v2−V20=kV20
Rearranging gives us:
v2=V20+kV20
Factoring out V20:
v2=V20(1+k)
Taking the square root of both sides, we find:
v=V0√1+k
Thus, the speed of the particle at time t=V202 is:
v=V0√1+k
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.8
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.9
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.6
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
The acceleration of a particle moving along a straight line at any time t is given by a = 4 - 2v, where v is the speed of particle at any time t The maximum velocity is
View SolutionThe speed v of a particle moving along a straight line is given by a+bv2=x2 where x is its distance from the origin. The acceleration of the particle is
View SolutionAcceleration time graph of a particle moving along a straight line is given. Then average acceleration between t=0 & t=4 is :−
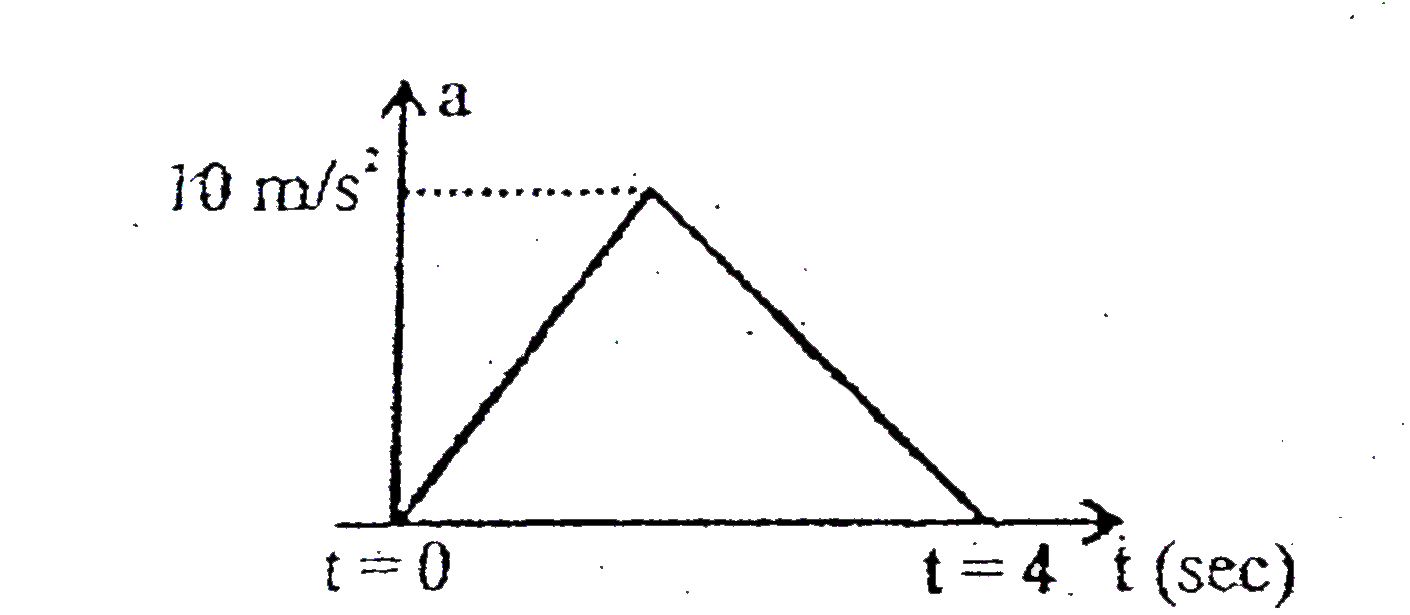
View SolutionThe velocity of a particle along a straight line increases according to the linear law v=v0+kx, where k is a constant. Then
View SolutionV=k(PT)0.33 where k is constant. It is an,
View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The displacement of aprticle from t=0 to t=2 seconds is :
View SolutionThe motion of a particle moving along x-axis is represented by the equation dvdt=6−3v, where v is in m/s and t is in second. If the particle is at rest at t = 0 , then
View SolutionThe acceleration versus time graph of a particle moving along a straight line is shown in the figure. Draw the respective velocity-time graph Given v=0 at t=0.

View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The distance travelled by particle from t=0 to t=2seconds is :
View Solution
