If a long hollow copper pipe carries a current, then magnetic field is produced:
inside the pipe only
outside the pipe only
both inside and outside the pipe
no where
The correct Answer is:B
To determine the magnetic field produced by a long hollow copper pipe carrying a current, we can follow these steps:
Step 1: Understand the Configuration
We have a long hollow copper pipe that carries an electric current. The pipe is hollow, meaning there is an empty space inside it.
Step 2: Apply Ampère's Law
According to Ampère's Law, the magnetic field (B) around a current-carrying conductor can be determined by considering the current enclosed by an imaginary loop (Amperian loop) around the conductor.
Step 3: Analyze the Inside of the Hollow Pipe
When we consider a loop inside the hollow portion of the pipe:
- The current enclosed by this loop is zero because there is no current flowing through the hollow space.
- Therefore, according to Ampère's Law, the magnetic field inside the hollow pipe (B_inside) is given by:
Binside=0
Step 4: Analyze the Outside of the Hollow Pipe
Now, consider a loop outside the hollow pipe:
- The current enclosed by this loop is equal to the total current flowing through the pipe.
- Hence, the magnetic field outside the pipe (B_outside) is non-zero and can be calculated using Ampère's Law.
Step 5: Conclusion
From the above analysis, we conclude that:
- The magnetic field inside the hollow copper pipe is zero.
- The magnetic field exists outside the hollow copper pipe.
Thus, the answer to the question is that the magnetic field is produced outside the pipe.
Final Answer
The magnetic field is produced outside the hollow copper pipe.
---
We have a long hollow copper pipe that carries an electric current. The pipe is hollow, meaning there is an empty space inside it.
According to Ampère's Law, the magnetic field (B) around a current-carrying conductor can be determined by considering the current enclosed by an imaginary loop (Amperian loop) around the conductor.
When we consider a loop inside the hollow portion of the pipe:
- The current enclosed by this loop is zero because there is no current flowing through the hollow space.
- Therefore, according to Ampère's Law, the magnetic field inside the hollow pipe (B_inside) is given by:
Binside=0
Now, consider a loop outside the hollow pipe:
- The current enclosed by this loop is equal to the total current flowing through the pipe.
- Hence, the magnetic field outside the pipe (B_outside) is non-zero and can be calculated using Ampère's Law.
From the above analysis, we conclude that:
- The magnetic field inside the hollow copper pipe is zero.
- The magnetic field exists outside the hollow copper pipe.
The magnetic field is produced outside the hollow copper pipe.
Topper's Solved these Questions
Similar Questions
If a long hollow copper pipe carriers a direct current, the magnetic field associated with the current will be:
View SolutionIf a long hollow copper pipe carriers a direct current, the magnetic field associated with the current will be:
View SolutionA long copper tube of inner radius R carriers a current i. The magnetic field B inside the tube is
View SolutionA long copper wire carries a current of I ampere. Calculate the magnetic flux per meter of the wire for a plane surface S inside the wire as shown in Fig.
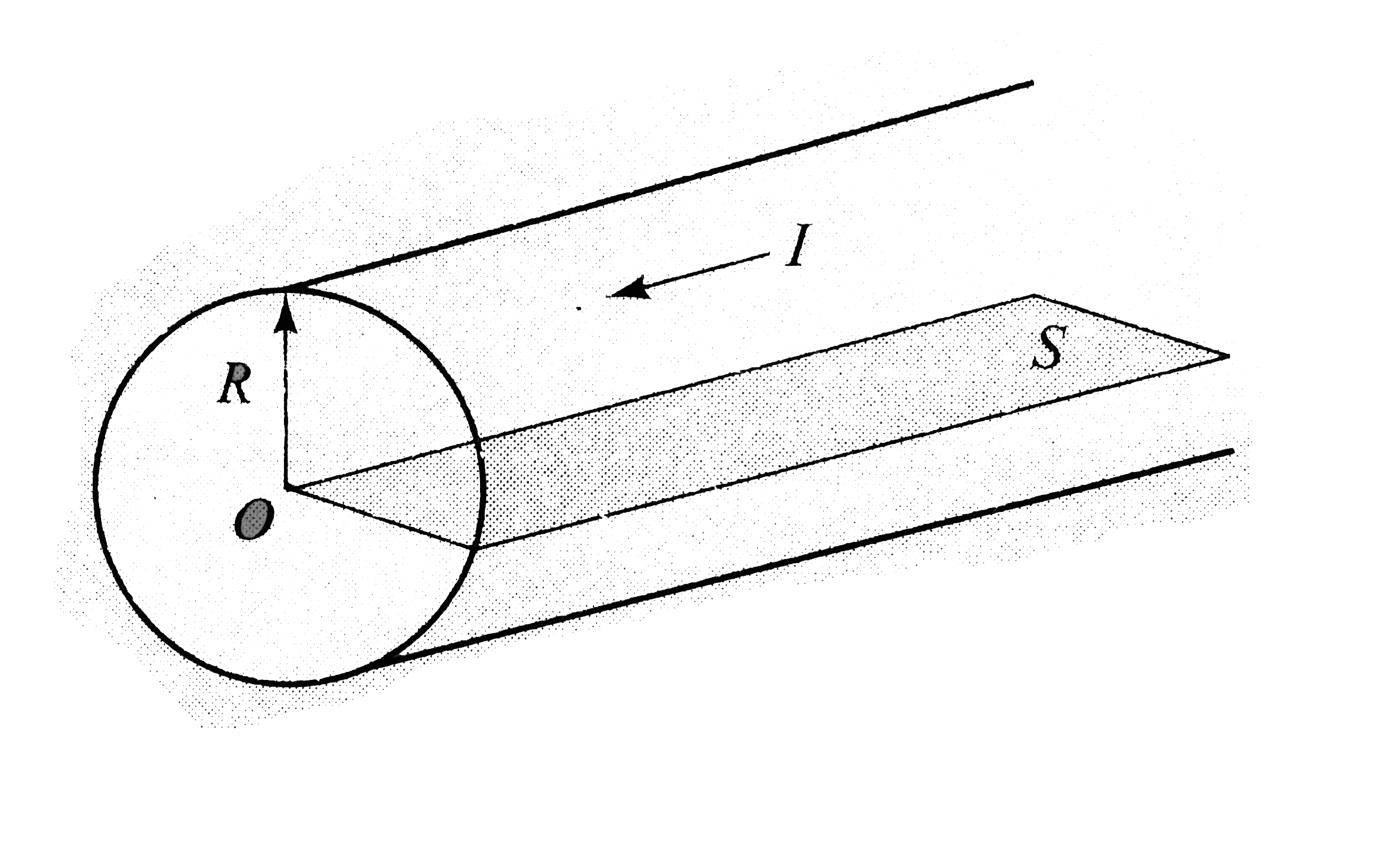
View SolutionA long solenoid has a radius a and number of turns per unit length is n. If it carries a current i, then the magnetic field on its axis is directly proportional to
View SolutionA long copper wire carries a current of 10 ampere . Calculate the magnetic flux per metre of the wire for a plane surface S inside the wire as shown in the figure.
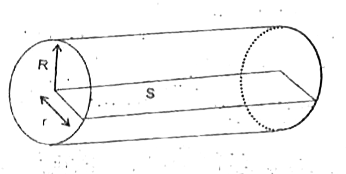
View SolutionA square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionA sphere of radius R is placed near a long straight wire that carries a steady current l. The magnetic field generated by the current is B. The total magnetic flux passing through the sphere is
View SolutionA long solenoid of 50 cm length having 100 turns carries a current of 2.5A. The magnetic field at centre of solenoid is:
View SolutionA long solenoid has 200 turns per cm and carries a current i. The magnetic field at its centre is 6.28×10−2weber/cm2. Another long soloenoid has 100 turns per cm and it carries a current i3. The value of the magnetic field at its centre is
View Solution