A particle moves along x-axis in positive direction. Its acceleration 'a' is given as a=cx+d, where x denotes the x- coordinate of particle, c and d are positive constants. For velocity position graph of particle to be of type as shown in figure, the value of speed of particle at x=0 should be 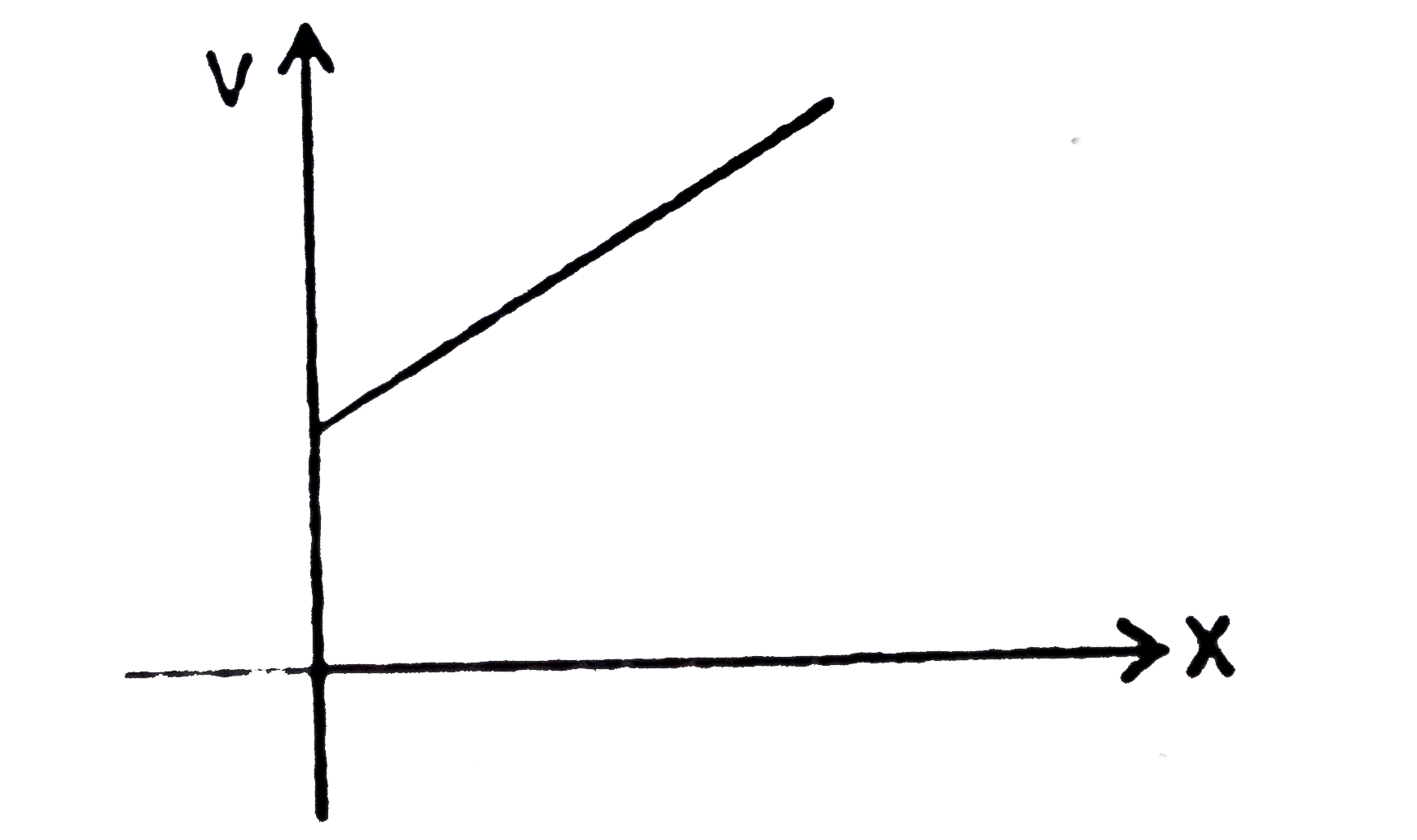
√2d2c
√(8d2c)
√4d2c
√d2c
The correct Answer is:D
a=Vdvdx=cx+d
Let at x=0v=u
∴∫vuvdv=∫x0(cx+d)dx
or v2=cx2+2dx+u2
v shall be linear function of x if cx2+2dx+u2 is perfect square
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
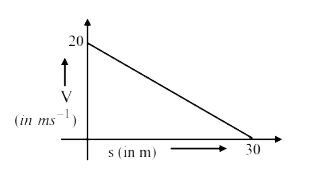
The velocity –position graph of a particle moving along a straight line is shown. Then acceleration of the particle at s = 15 m is :
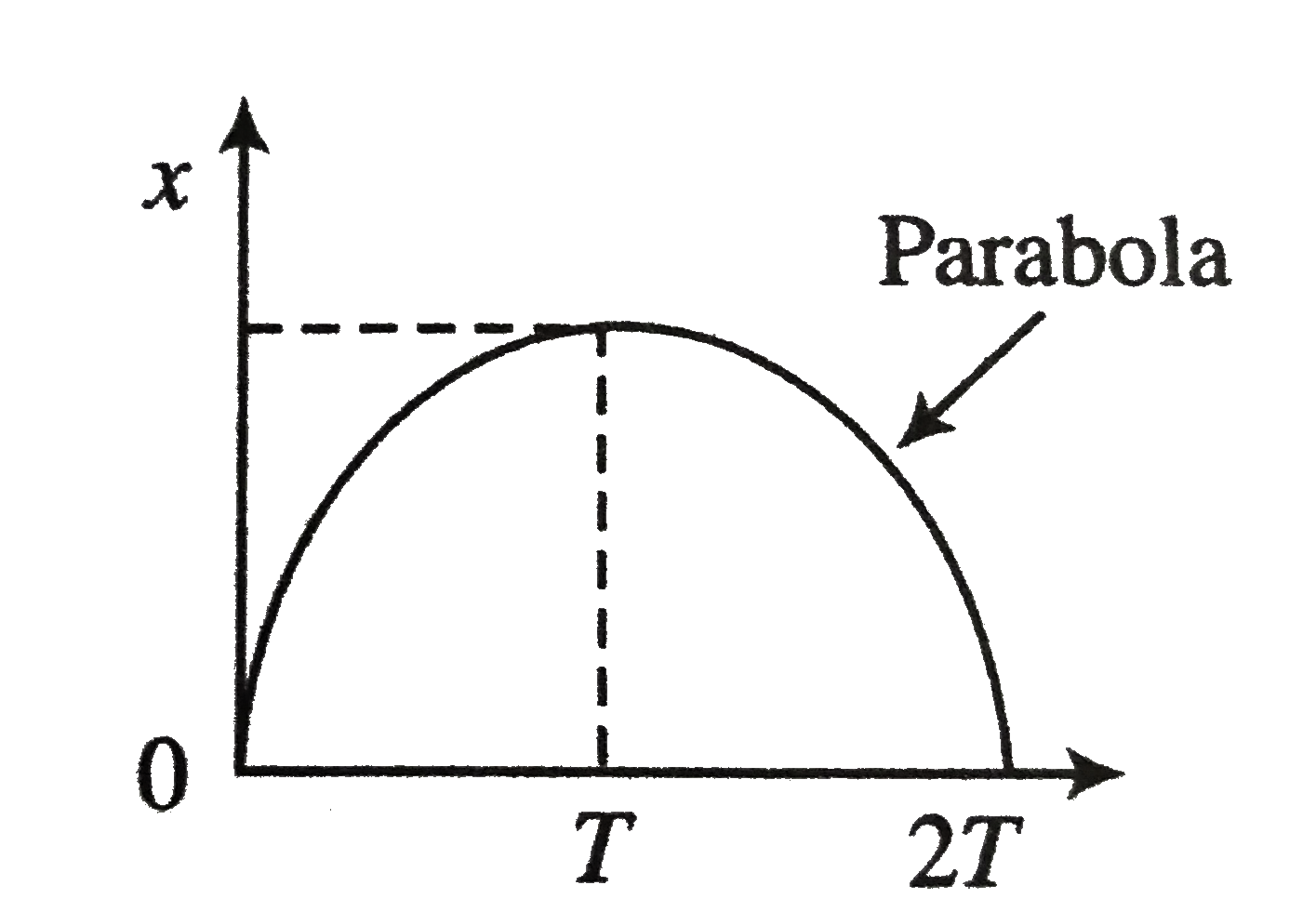
View SolutionThe x-t graph of a particle moving along a straight line is shown in figure
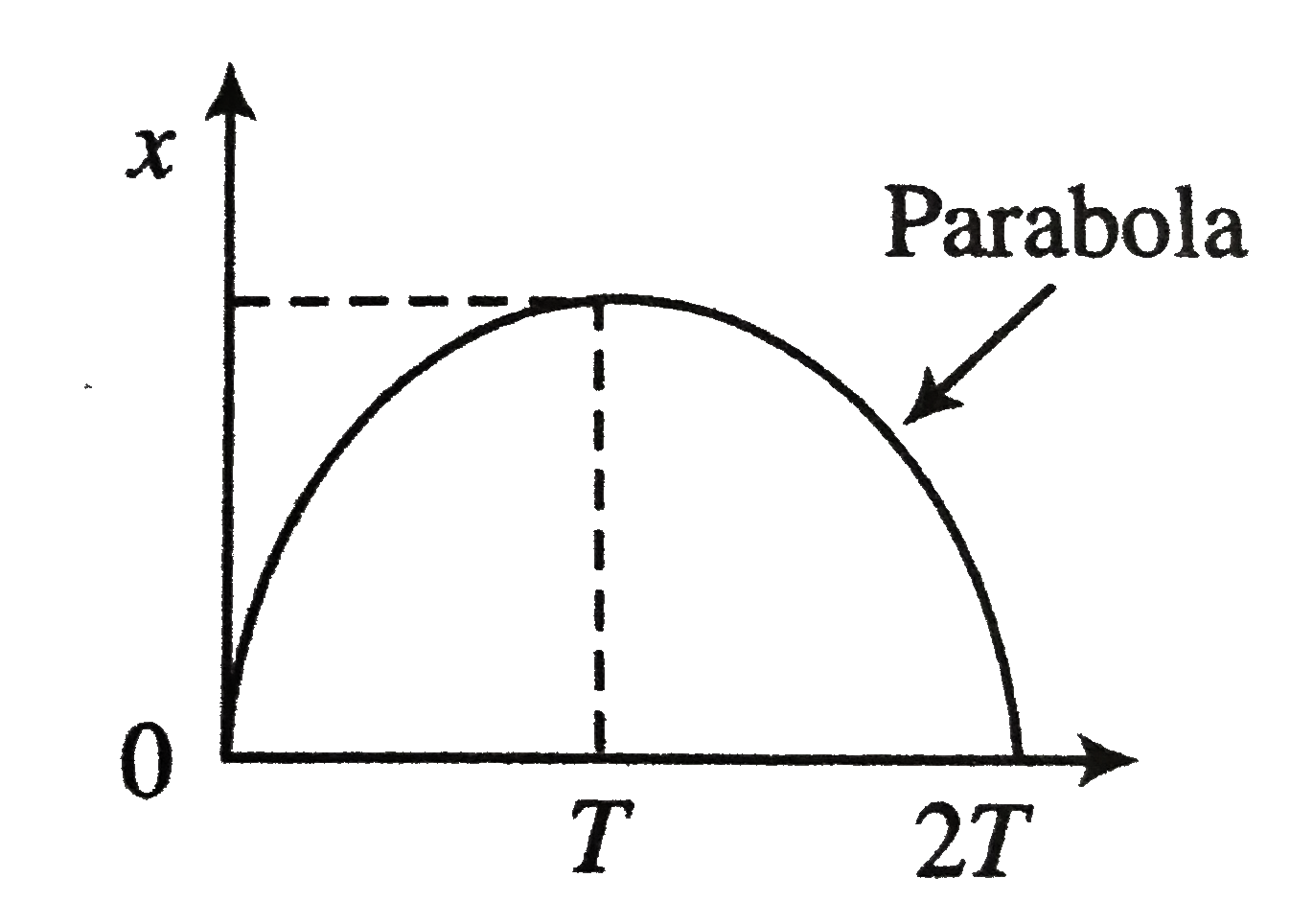
The v-t graph of the particle is correctly shown by
View SolutionPosition-time graph for a particle moving along x− direction is as shown in the figure. Average speed of the particle from t=0 to t=4 is :

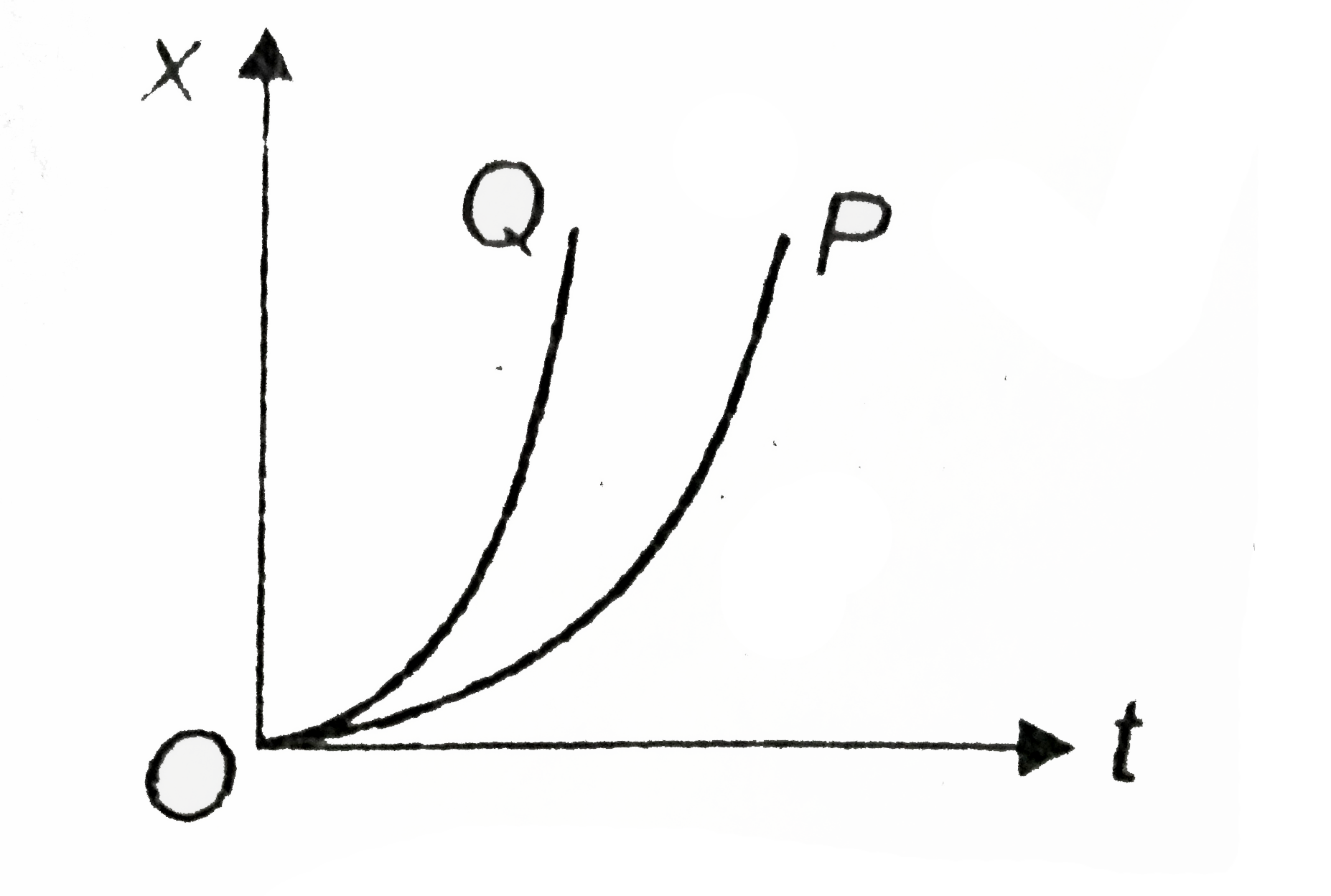
View SolutionThe position-time graph for two particles P and Q moving on x-axis is shown in the figure. Then
View SolutionThe position (X)-time (t) graph for a particle of mass 1 kg moving along x-axis is shown in figure. Find impulse on particle at t = 4 s.
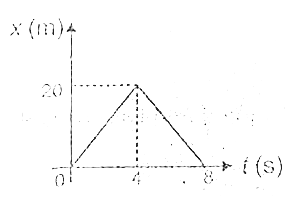
View SolutionThe x-t graph of a particle moving along a straight line is shown in figure
The speed-time graph of the particle is correctly shown by
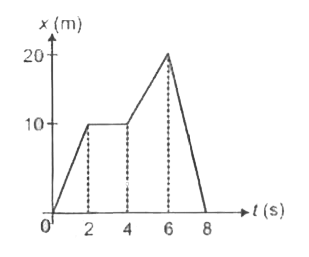
View SolutionThe position (x) - time (t) graph for a particle moving along a straight line is shown in figure. The average speed of particle in time interval t = 0 to t = 8 s is
View SolutionA particle starts from the origin at time t = 0 and moves along the positive x-axis. The graph of velocity with respect to time is shown in figure. What is the position of the particle at time t = 5s ?

View SolutionA particle moving along the X-axis executes simple harmonic motion, then the force acting on it is given by
where, A and K are positive constants.
View SolutionThe velocity of a particle moving along x-axis is given as v=x2−5x+4 (in m/s) where x denotes the x-coordinate of the particle in metres. Find the magnitude of acceleration of the particle when the velocity of particle is zero?
View Solution
