A block of mass $m$ is connected to a spring (spring constant $k$ ). Initially the block is at rest and the spring is in its natural length. Now the system is released in gravitational field and a variable force $F$ is applied on the upper end of the spring such that the downward acceleration of the block is given as $a = g - α t$ , where $t$ is time elapses and $α = 1 m / s^{2}$ , the velocity of the point of application of the force is:

A

$\frac{m α}{k} - gt - \frac{α t^{2}}{2}$

B

$\frac{m α}{k} - gt - α t^{2}$

C

$\frac{m α}{k} - gt + \frac{α t^{2}}{2}$

D

$\frac{m α}{k} + gt + \frac{α t^{2}}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

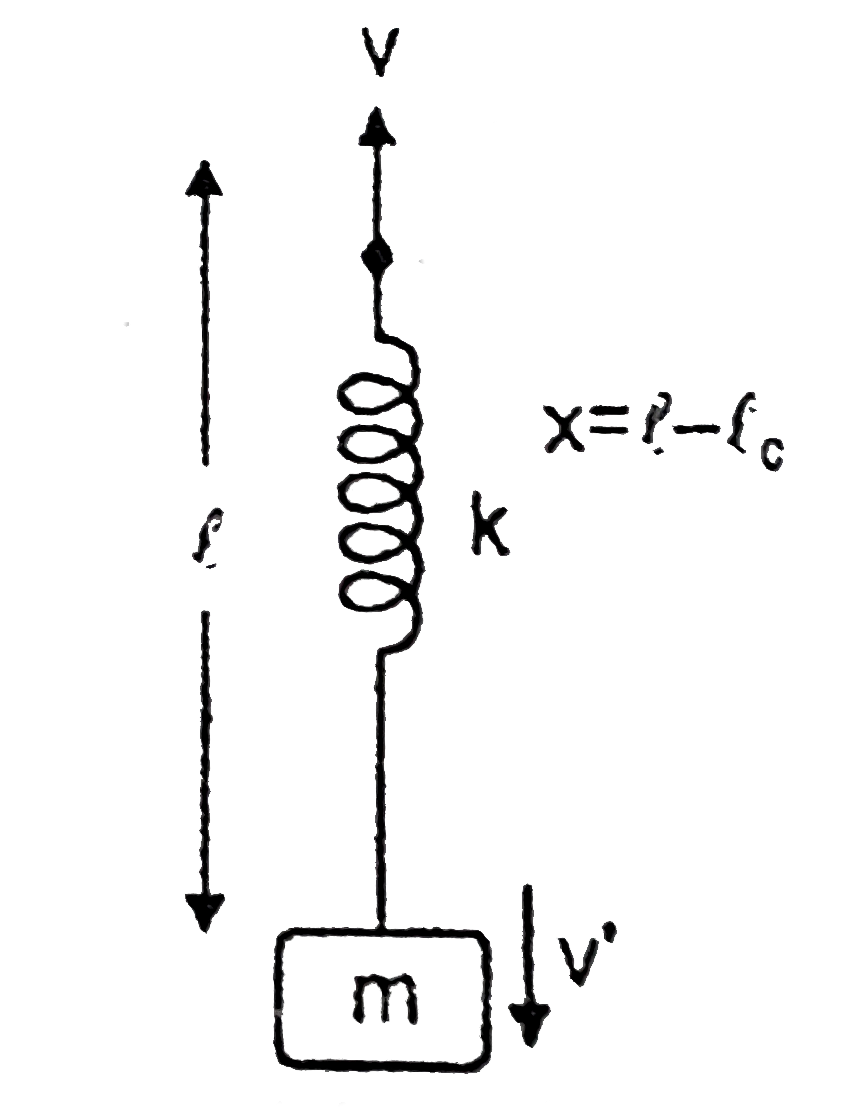
Let thhe elongation of the spring be $x$ . From the N.L.M of the block
$m g - k x = m (g - t)$
$k x = m t$
or $k (I - I_{0}) = m t$
Differentiatinng w.r.t time
$k (\frac{d l}{d t}) = m$
$V = \frac{m}{k} - V^{'} = \frac{m}{k} - \int_{0}^{1} (g - t) d t$
$= \frac{m}{k} - g t + \frac{t^{2}}{2}$

Updated on:21/07/2023

|

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-TEST PAPERS-PHYSICS

Home
Ask Doubt
Profile