A uniformly tapering conical wire is made from a material of young's modulus Y and has a normal unextended length L the radii at the upper and lower ends of this conical wire, have values R and 3R, respectively the upper end of the wire is fixed to a rigid support and a mass M is suspended from its lower end. the equilibrium extended length of this wire would equal to:
a. L(1+13MgπYR2)
b. L(1+23MgπYR2)
c. L(1+19MgπYR2)
d. L(1+29MgπYR(2))
The correct Answer is:A
To find the equilibrium extended length of a uniformly tapering conical wire under the influence of a suspended mass, we can follow these steps:
Step 1: Understand the Geometry of the Wire
The conical wire has a radius R at the upper end and 3R at the lower end. The unextended length of the wire is L.
Step 2: Define the Variables
- Let Y be the Young's modulus of the material.
- Let M be the mass suspended from the lower end of the wire.
- The weight of the mass is given by W=Mg, where g is the acceleration due to gravity.
Step 3: Consider an Element of the Wire
Consider a small element of the wire at a distance x from the top, with a thickness dx. The radius of this element can be expressed as a function of x.
Step 4: Establish the Relationship for Radius
Using similar triangles, the radius r at a distance x can be expressed as:
r(x)=R+(3R−R)Lx=R+2RLx=R(1+2xL)
Step 5: Calculate the Area of Cross-Section
The area A of the cross-section of the wire at distance x is:
A=πr2=π(R(1+2xL))2=πR2(1+2xL)2
Step 6: Calculate the Stress and Strain
The stress σ on the element is given by:
σ=WA=MgπR2(1+2xL)2
The strain ϵ is given by:
ϵ=σY=MgYπR2(1+2xL)2
Step 7: Relate Strain to Elongation
The elongation dL of the small element dx can be expressed as:
dL=ϵ⋅dx=MgYπR2(1+2xL)2dx
Step 8: Integrate to Find Total Elongation
To find the total elongation ΔL of the wire, integrate dL from 0 to L:
ΔL=∫L0MgYπR2(1+2xL)2dx
Step 9: Solve the Integral
Let u=1+2xL, then du=2Ldx or dx=L2du. The limits change from x=0 to x=L which corresponds to u=1 to u=3:
ΔL=∫31MgYπR2u2⋅L2du=MgL2YπR2(−1u)31
=MgL2YπR2(−13+1)=MgL2YπR2⋅23=MgL3YπR2
Step 10: Calculate the Total Extended Length
The total extended length L′ of the wire is:
L′=L+ΔL=L+MgL3YπR2
=L(1+Mg3YπR2)
Final Answer
The equilibrium extended length of the conical wire is:
L′=L(1+Mg3YπR2)
The conical wire has a radius R at the upper end and 3R at the lower end. The unextended length of the wire is L.
- Let Y be the Young's modulus of the material.
- Let M be the mass suspended from the lower end of the wire.
- The weight of the mass is given by W=Mg, where g is the acceleration due to gravity.
Consider a small element of the wire at a distance x from the top, with a thickness dx. The radius of this element can be expressed as a function of x.
Using similar triangles, the radius r at a distance x can be expressed as:
r(x)=R+(3R−R)Lx=R+2RLx=R(1+2xL)
The area A of the cross-section of the wire at distance x is:
A=πr2=π(R(1+2xL))2=πR2(1+2xL)2
The stress σ on the element is given by:
σ=WA=MgπR2(1+2xL)2
ϵ=σY=MgYπR2(1+2xL)2
The elongation dL of the small element dx can be expressed as:
dL=ϵ⋅dx=MgYπR2(1+2xL)2dx
To find the total elongation ΔL of the wire, integrate dL from 0 to L:
ΔL=∫L0MgYπR2(1+2xL)2dx
Let u=1+2xL, then du=2Ldx or dx=L2du. The limits change from x=0 to x=L which corresponds to u=1 to u=3:
ΔL=∫31MgYπR2u2⋅L2du=MgL2YπR2(−1u)31
=MgL2YπR2(−13+1)=MgL2YπR2⋅23=MgL3YπR2
The total extended length L′ of the wire is:
L′=L+ΔL=L+MgL3YπR2
=L(1+Mg3YπR2)
The equilibrium extended length of the conical wire is:
L′=L(1+Mg3YπR2)
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
A metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
View SolutionA body of mass M is attached to the lower end of a metal wire, whose upper end is fixed . The elongation of the wire is l.
View Solution
Knowledge Check
A wire of length L and cross-sectional area A is made of a material of Young's modulus Y. IF the wire is stretched by an amount x, the workdone is
AYAx22LBYAx2L2CYAx2LDYAx2L
A wire of natural length l, young's modulus Y and area of cross-section A is extended by x. Then, the energy stored in the wire is given by
View SolutionA wire of length L,area of cross section A is hanging from a fixed support. The length of the wire changes to L1 when mass M is suspended from its free end. The expression for Young's modulus is:
View SolutionTwo wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is
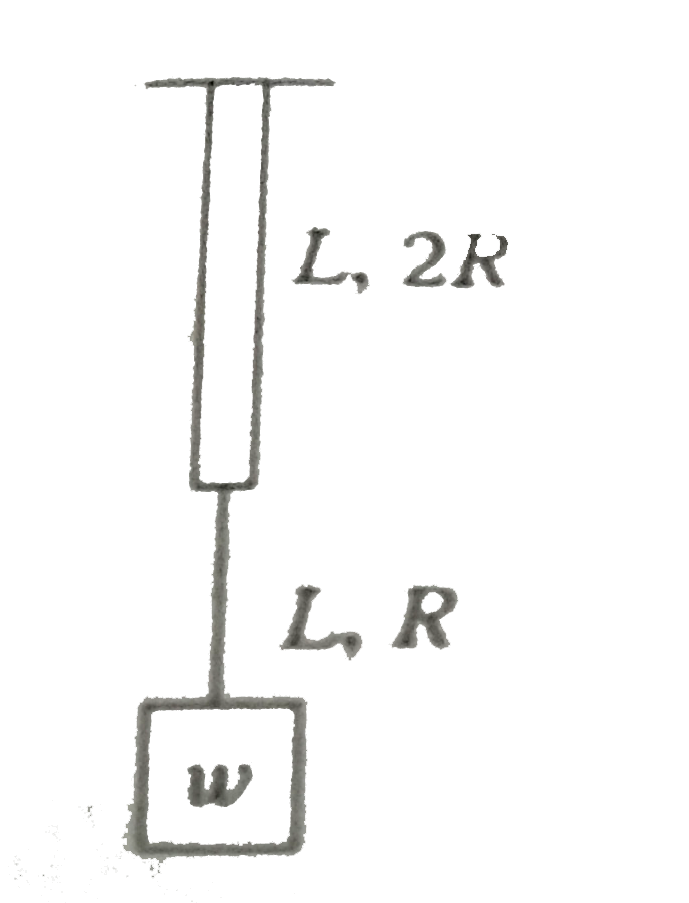
View SolutionTwo wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is

View SolutionA steel wire 2m long is suspended from the ceiling. When a mass is hung from its lower end, the increase in length recorded is 1cm. Determine the strain in the wire.
View SolutionA uniform rod of mass m is hinged at one end and other end is connected to a light extensible string having length L.If the Youngs modulus and crosssectional area of the wire is Y and A respectively then the elongation in the string is
View SolutionA thin rod of mass m and length l is hinged at the lower end to a level floor and stands vertically. Then its upper end will strike the floor with a velocity given by:
View Solution
