A radioactive substance X decays into another radioactive substance Y Initially only X was present. λx and λyy are the disnttegration constants of Xa nd Y Nx and Ny are the number of nuclie of X and Y at any time t. Number of nuclei Ny will be maximum when
NYNX−NY=λYλX−λY
NXNX−NY=(λYλX−λY)2
λYNY=λXNX
λYNX=λXNY
The correct Answer is:C
At any instant
dNydt=λxNx−λyNy
For maximum Ny,dNydt=0
∴λxNx=λyNy
Topper's Solved these Questions
Similar Questions
A radioactive substance X decys into another radioactive substance Y. Initially, only X was present . λx and λy are the disintegration constant of X and Y. Ny will be maximum when.
View SolutionA radioactive substance A having N0 active nuclei at t=0, decays to another radioactive substance B with decay constant λ1. B further decays to a stable substance C with decay constant λ2. (a) Find the number of nuclei of A,B and C time t. (b) What should be the answer of part (a) if λ1>>λ2 and λ1<<λ2
View SolutionHalf-life of a radioactive substance A is two times the half-life of another radioactive substance B. Initially, the number of A and B are NA and NB, respectively . After three half-lives of A, number of nuclei of both are equal. Then, the ratio NA/NB is .
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
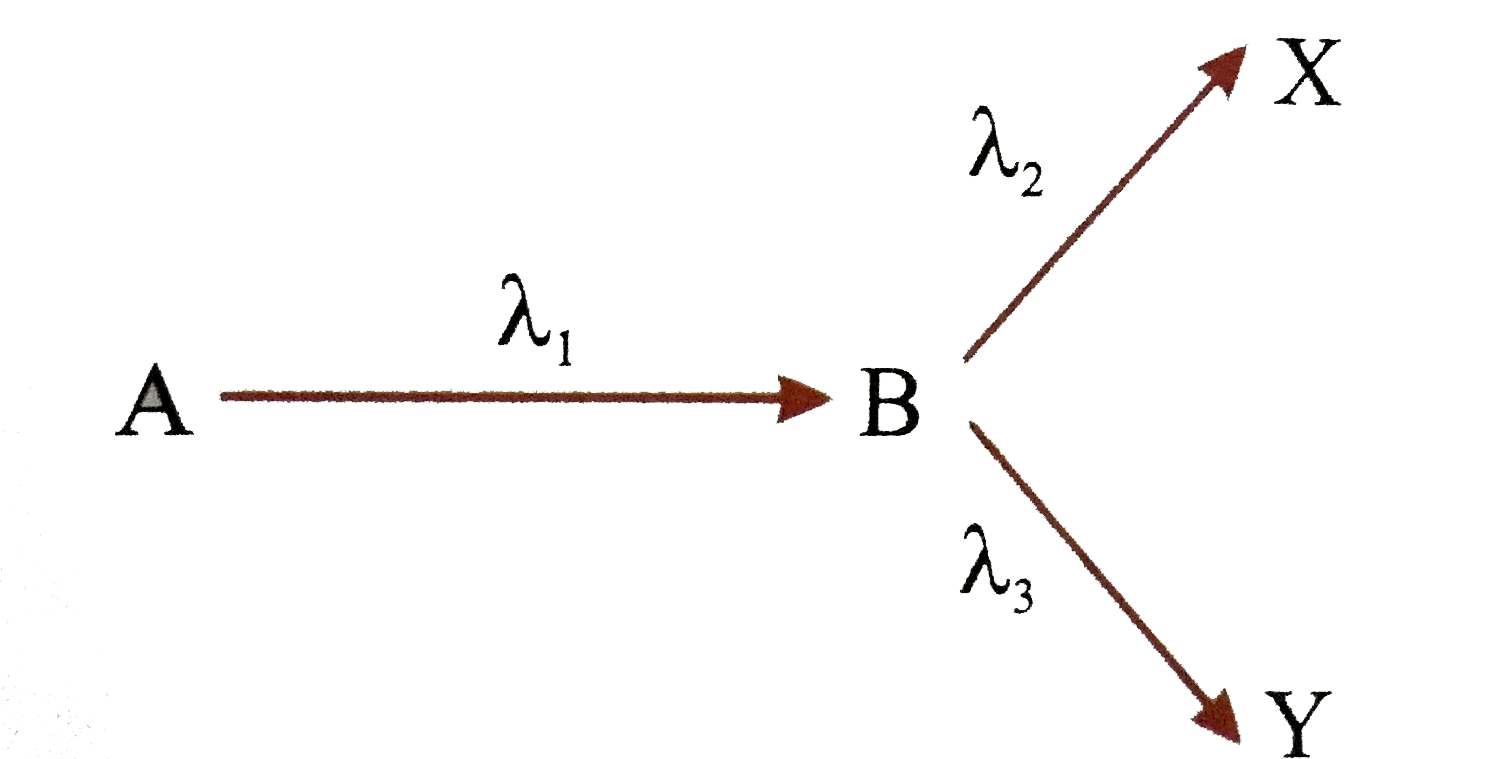
The number of nuclei of B will first increase and then after a maximum value, it decreases forView SolutionNucleus A decays into B with a decay constant λ1 and B further decays into C with a decay constant λ2 . Initially, at t = 0, the number of nuclei of A and B were 3N0 and N0 respectively. If at t = t0 number of nuclei of B becomes constant and equal to 2N0, then
View SolutionSuppose, the daughter nucleus in a nuclear decay is itself radioactive. Let λpandλd be the decay constants of the parent and the daughter nuclei. Also, let NpandNd be the number of parent and daughter nuclei at time t. Find the condition for which the number of daughter nuclei becomes constant.
View SolutionSuppose, the daughter nucleus in a nuclear decay is itself radioactive. Let λpandλd be the decay constants of the parent and the daughter nuclei. Also, let NpandNd be the number of parent and daughter nuclei at time t. Find the condition for which the number of daughter nuclei becomes constant.
View SolutionSuppose, the daughter nucleus in a nuclear decay is itself radioactive. Let λpandλd be the decay constants of the parent and the daughter nuclei. Also, let NpandNd be the number of parent and daughter nuclei at time t. Find the condition for which the number of daughter nuclei becomes constant.
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
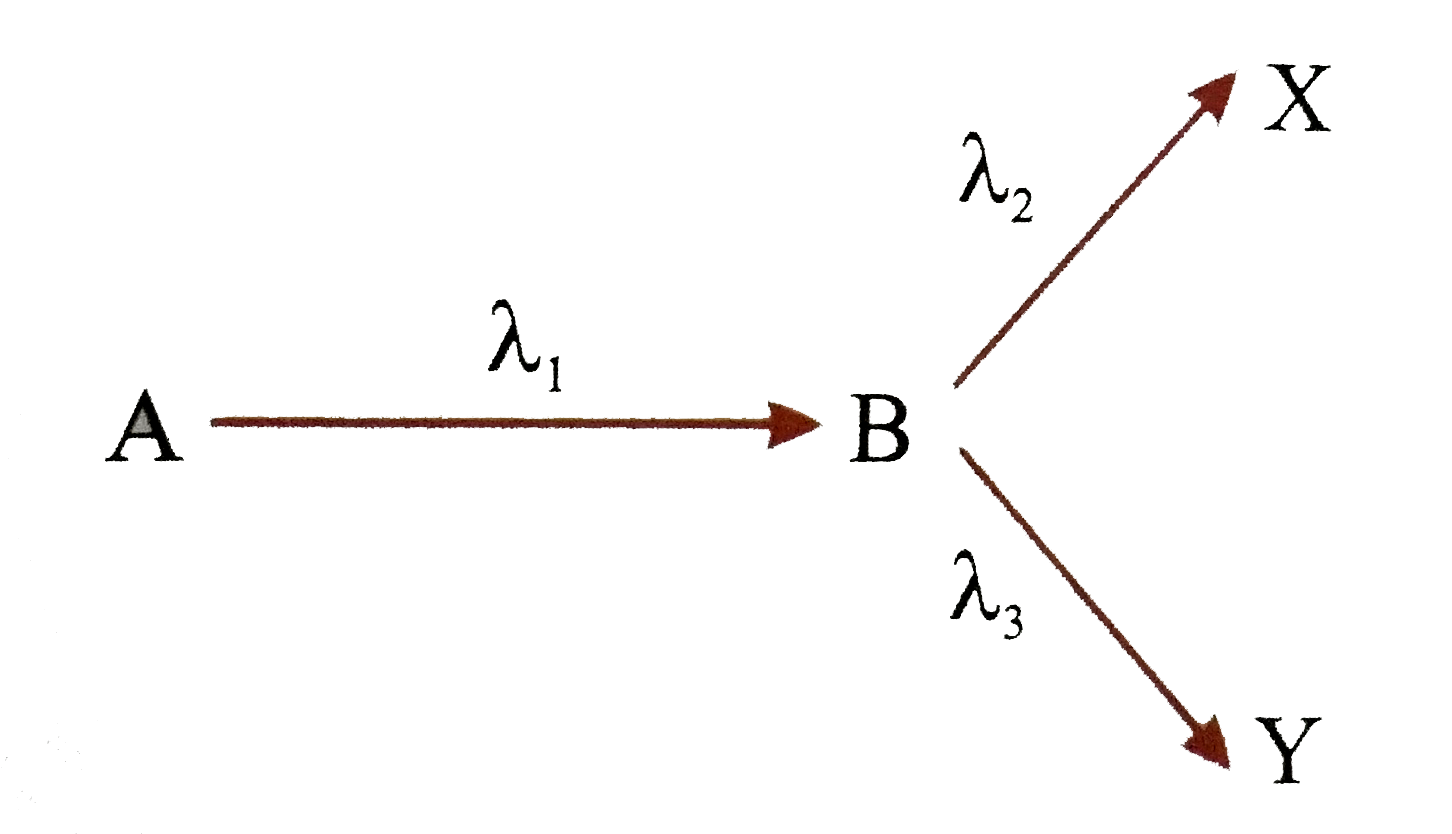
The net rate of accumulation of B at any instant isView SolutionTwo radioactive substances have half-lives T and 2T. Initially, they have equal number of nuclei. After time t=4T, the ratio of their number of nuclei is x and the ratio of their activity is y. Then,
View Solution

