Suppose the drift velocity vd in a material varied with the applied electric field E as vd∝√E. Then V – I graph for a wire made of such a material is best given by :
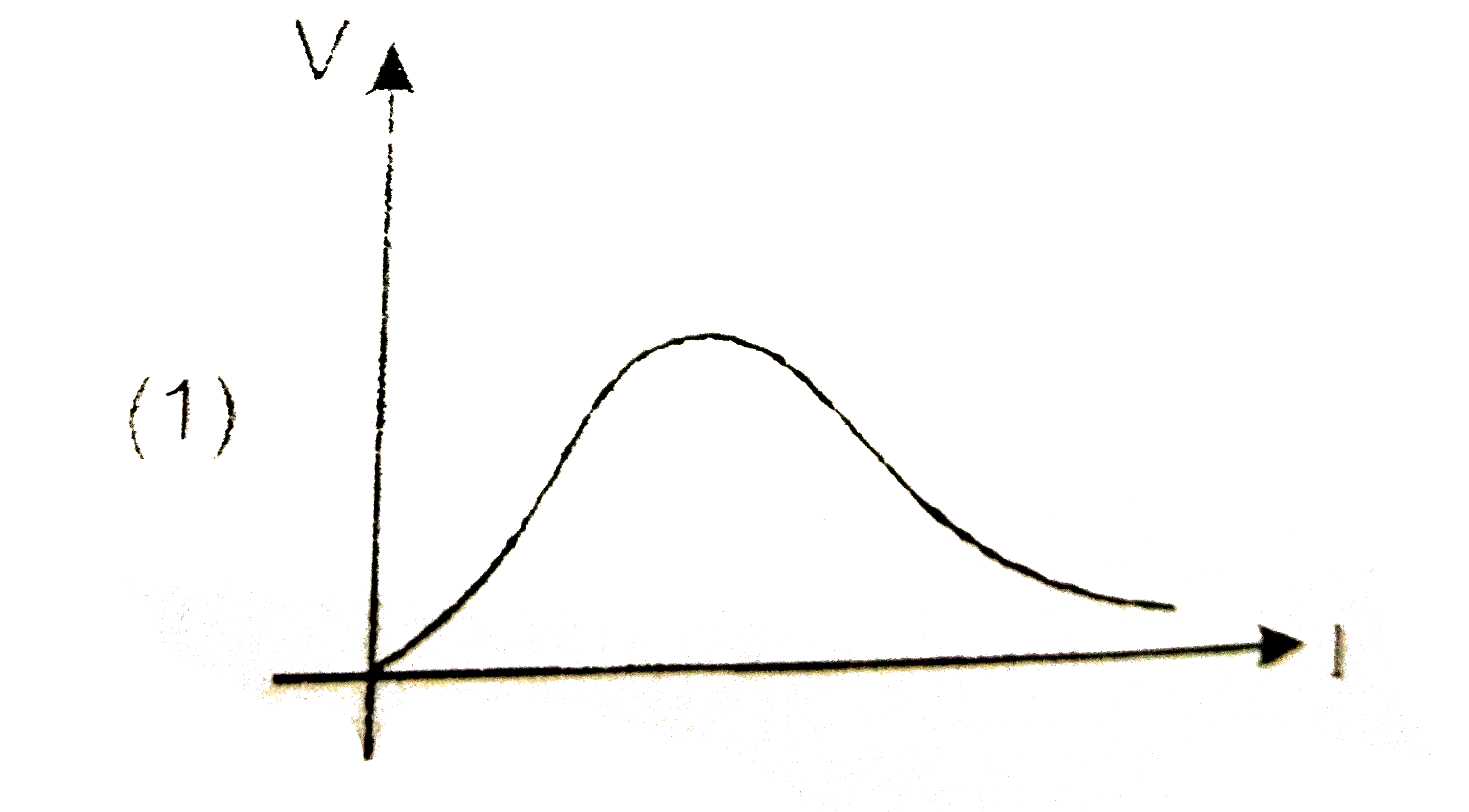
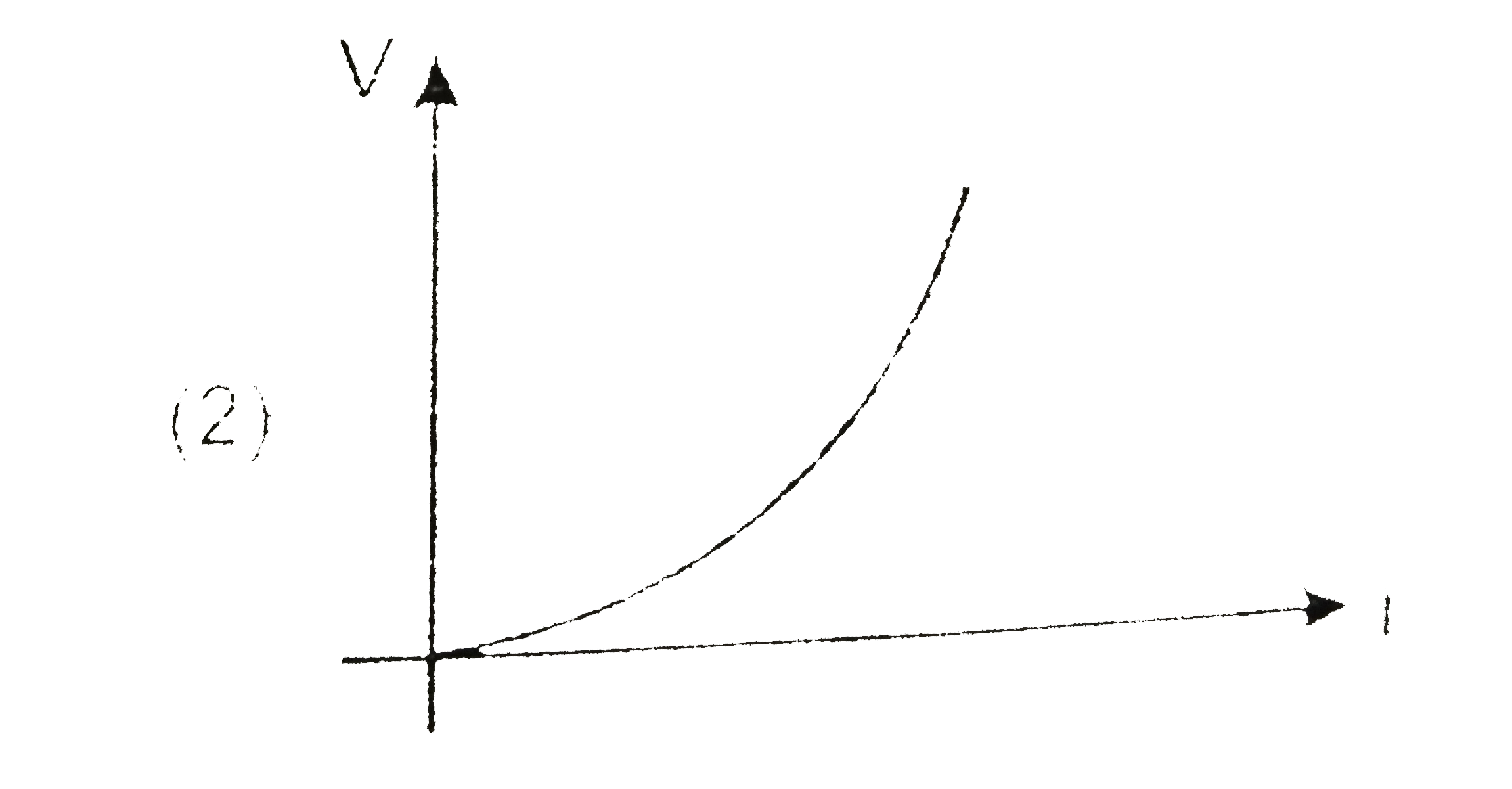

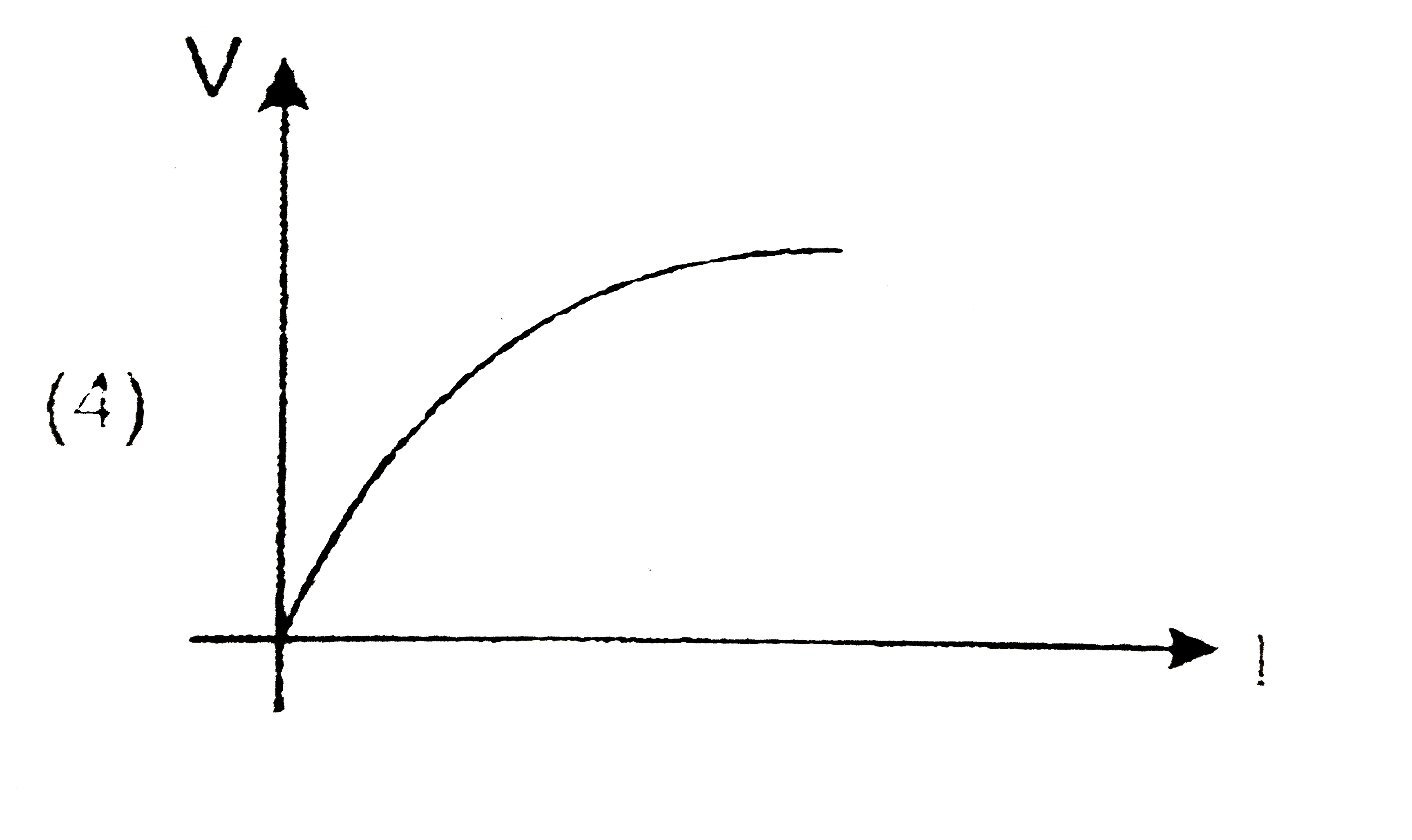
The correct Answer is:B
To solve the problem, we need to analyze the relationship between the drift velocity vd, the electric field E, and the current I in the wire. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the relationship between drift velocity and electric field
We are given that the drift velocity vd is proportional to the square root of the electric field E:
vd∝√E
This can be expressed mathematically as:
vd=k√E
where k is a proportionality constant.
Step 2: Relate drift velocity to current
The current I in a conductor is given by the formula:
I=neAvd
where:
- n is the number density of charge carriers,
- e is the charge of an electron,
- A is the cross-sectional area of the wire,
- vd is the drift velocity.
Step 3: Substitute the expression for drift velocity
Substituting the expression for vd from Step 1 into the current equation:
I=neA(k√E)=neAk√E
This shows that the current I is directly proportional to √E:
I∝√E
Step 4: Relate electric field to potential difference
The electric field E can also be expressed in terms of the potential difference V and the length L of the conductor:
E=VL
Step 5: Substitute electric field in the current equation
Now, substituting E in terms of V:
I∝√VL
Since L is a constant for a given wire, we can simplify this to:
I∝√V
Step 6: Express the relationship between voltage and current
From the relationship I∝√V, we can express it as:
I=k′√V
where k′ is a new constant.
Step 7: Square both sides to find the relationship between V and I
Squaring both sides gives:
I2=(k′)2V
This indicates that the potential difference V is proportional to the square of the current I:
V∝I2
Step 8: Identify the shape of the V-I graph
The relationship V∝I2 describes a parabolic curve that opens upwards, which means the graph of V versus I is a parabola that is concave upwards.
Conclusion
Thus, the V-I graph for a wire made of such a material is best represented by a parabolic curve concave upwards.
Final Answer
The correct option is B.
---
We are given that the drift velocity vd is proportional to the square root of the electric field E:
vd∝√E
This can be expressed mathematically as:
vd=k√E
where k is a proportionality constant.
The current I in a conductor is given by the formula:
I=neAvd
where:
- n is the number density of charge carriers,
- e is the charge of an electron,
- A is the cross-sectional area of the wire,
- vd is the drift velocity.
Substituting the expression for vd from Step 1 into the current equation:
I=neA(k√E)=neAk√E
This shows that the current I is directly proportional to √E:
I∝√E
The electric field E can also be expressed in terms of the potential difference V and the length L of the conductor:
E=VL
Now, substituting E in terms of V:
I∝√VL
Since L is a constant for a given wire, we can simplify this to:
I∝√V
From the relationship I∝√V, we can express it as:
I=k′√V
where k′ is a new constant.
Squaring both sides gives:
I2=(k′)2V
This indicates that the potential difference V is proportional to the square of the current I:
V∝I2
The relationship V∝I2 describes a parabolic curve that opens upwards, which means the graph of V versus I is a parabola that is concave upwards.
Thus, the V-I graph for a wire made of such a material is best represented by a parabolic curve concave upwards.
The correct option is B.
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
Drift velocity vd varies with the intensity of electric field as per their relation
View SolutionA charged oil drop falls with terminal velocity v0 in the absence of electric field. An electric field E keeps it stationary. The drop acquires charge 3q, it starts moving upwards with velocity v0. The initial charge on the drop is
View Solution
Knowledge Check
For which of the following depndences of drift velocity, vd on electric field E, Ohm's law obeyed?
AvdαEBvdαE2Cvdα√EDvdα1√E
A current .I. flows through a metallic wire of radius .r. and the free electrons in it drift with a velocity vd. Calculate the drift velocity of the free electrons through the wire of the same material, having double the radius, when same current flows through it.
View SolutionThe drift velocity of free electron in a metal wire of a given potential gradient along it is Vd . If this potential gradient is doubled the new drift velocity will be
View SolutionThe electric field and the electric potential at a point are E and V respectively. Give the relationship between E and V
View SolutionAn electron projectes with velocity →v=v0ˆi in the electric field →E=E0ˆj. There the path followed by the electron E0.
View SolutionThe electric field and the electric potential at a point are E and V respectively.
View SolutionWhich of the following is a true nut?
View SolutionLet V0 be the potential at the origin in an electric field →E=Exˆi+Eyˆj. The potential at the point (x,y) is
View Solution
