A simple pendulum , a physical pendulum, a torsional pendulum and a spring-mass systeam, each of same frequency are taken to the Moon. If frequencies are measured on the moon, which systeam of systeams will have it unchanged?
spring-mass system and torisonal pendulum.
only spring-mass system.
spring-mass system and physical pendulum.
None of these
The correct Answer is:A
To solve the problem, we need to analyze the frequency of each system when taken to the Moon, where the acceleration due to gravity (g) is different from that on Earth.
1. Understand the systems: We have four systems:
- Simple Pendulum
- Physical Pendulum
- Torsional Pendulum
- Spring-Mass System
2. Frequency of Simple Pendulum:
The frequency f of a simple pendulum is given by:
f=1T=12π√gL
where g is the acceleration due to gravity and L is the length of the pendulum. Since g changes on the Moon, the frequency will change.
3. Frequency of Physical Pendulum:
The frequency of a physical pendulum is also dependent on g:
f=1T=12π√gI/mh
where I is the moment of inertia, m is the mass, and h is the distance from the pivot to the center of mass. Since g changes, the frequency will also change.
4. Frequency of Torsional Pendulum:
The frequency of a torsional pendulum is given by:
f=1T=12π√kI
where k is the torsional constant and I is the moment of inertia. This system does not depend on g, so its frequency remains unchanged on the Moon.
5. Frequency of Spring-Mass System:
The frequency of a spring-mass system is given by:
f=1T=12π√km
where k is the spring constant and m is the mass. This system also does not depend on g, so its frequency remains unchanged on the Moon.
6. Conclusion:
The systems whose frequencies remain unchanged when taken to the Moon are the Torsional Pendulum and the Spring-Mass System. Therefore, the answer is that the Torsional Pendulum and Spring-Mass System will have unchanged frequencies.
Final Answer:
The systems that will have unchanged frequencies on the Moon are the Torsional Pendulum and the Spring-Mass System.
- Simple Pendulum
- Physical Pendulum
- Torsional Pendulum
- Spring-Mass System
The frequency f of a simple pendulum is given by:
f=1T=12π√gL
where g is the acceleration due to gravity and L is the length of the pendulum. Since g changes on the Moon, the frequency will change.
The frequency of a physical pendulum is also dependent on g:
f=1T=12π√gI/mh
where I is the moment of inertia, m is the mass, and h is the distance from the pivot to the center of mass. Since g changes, the frequency will also change.
The frequency of a torsional pendulum is given by:
f=1T=12π√kI
where k is the torsional constant and I is the moment of inertia. This system does not depend on g, so its frequency remains unchanged on the Moon.
The frequency of a spring-mass system is given by:
f=1T=12π√km
where k is the spring constant and m is the mass. This system also does not depend on g, so its frequency remains unchanged on the Moon.
The systems whose frequencies remain unchanged when taken to the Moon are the Torsional Pendulum and the Spring-Mass System. Therefore, the answer is that the Torsional Pendulum and Spring-Mass System will have unchanged frequencies.
The systems that will have unchanged frequencies on the Moon are the Torsional Pendulum and the Spring-Mass System.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - II
1 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - III
12 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II
36 Videos
- SEMICONDUCTORSBOOK - RESONANCE ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS
784 Videos
Similar Questions
Knowledge Check
A simple pendulum is oscillating in a stationery lift. When the lift falls freely, the frequency of oscillations of the pendulum is
AzeroBυC2υDinfiniteThe time period of a simple pendulum on the surface of the earth is 4s. Its time period on the surface of the moon is
A4sB8sC10sD12s
A simple pendulum completes 40 oscillations in one minute. Find its frequency
View SolutionWhat is a simple pendulum? Find an expression for the time period and frequency of a simple pendulum.
View SolutionThe time period of a simple pendulum is 2 s. What is its frequency?
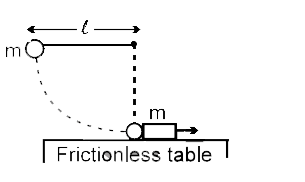
View SolutionThe bob of a simple pendulum of length l dropped from a horizontal position strikes a block of the same mass elastically, placed on a horizontal table (frictionless) as shown in the diagram, the block shall have kinetic energy-
View SolutionThe time period of a simple pendulum is 2 s. What is its frequency ? What name is given to such a pendulum ?
View SolutionA simple pendulum makes 100 oscillation in 25 s. What is the frequency of the pendulum correctto the significant figures?
View Solution
