Assuming all the surfaces to be smoth, if the time period of motion of the ball is N×10−1sec then find N. Neglect the small effect of bend near the bottom. (g=10m/s2)
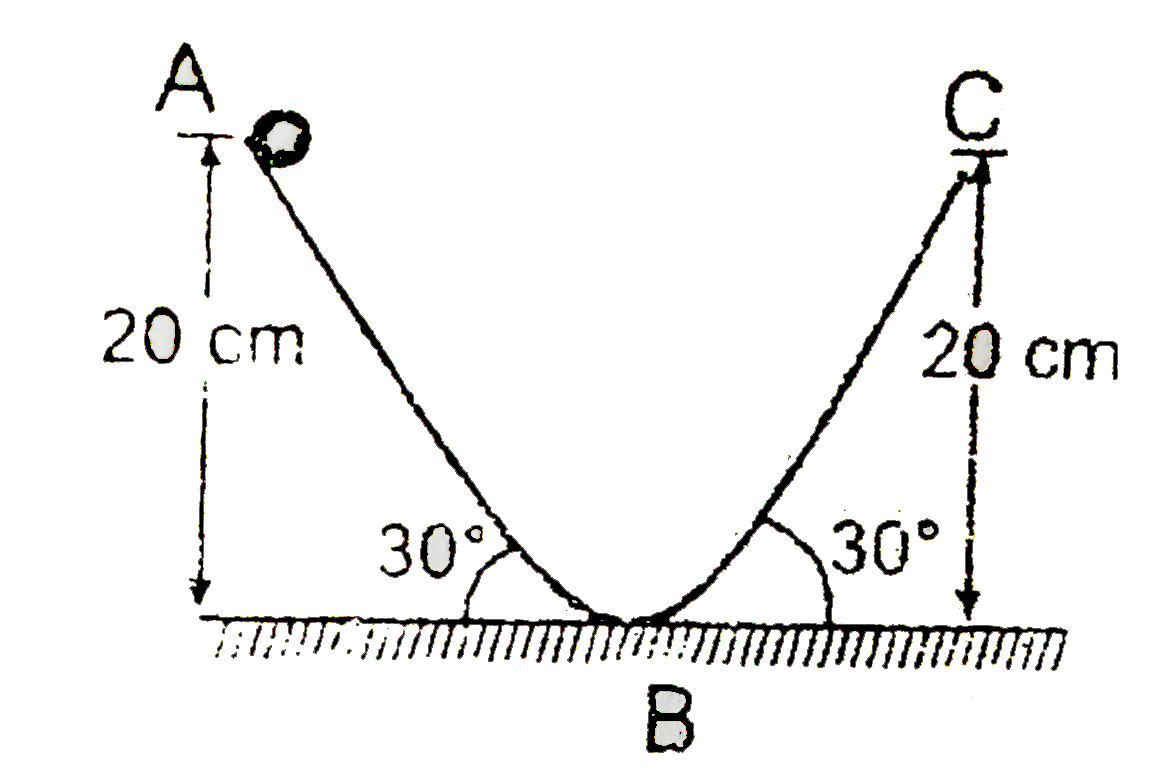
The correct Answer is:16
S=hsin30,a=gsin30,h=20cm,u=0
S=ut+12at2
⇒t=√2Sa=√2×(0.2×2)10×(0.5)=0.4
For BC max height reached by the particle will be same as h=20cm
(by energy conservation)
and for BA and BC,t=0.4
Total time period =0.4×4=1.6 second =16×10−1sec
∴N=16
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - II1videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - III12videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II36videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 388videos
SEMICONDUCTORS
- BOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS784videos
TEST PAPERS
Similar Questions
Find the time period of the motion of the particle shown in figure. Neglected small effect of the bend near the bottom.
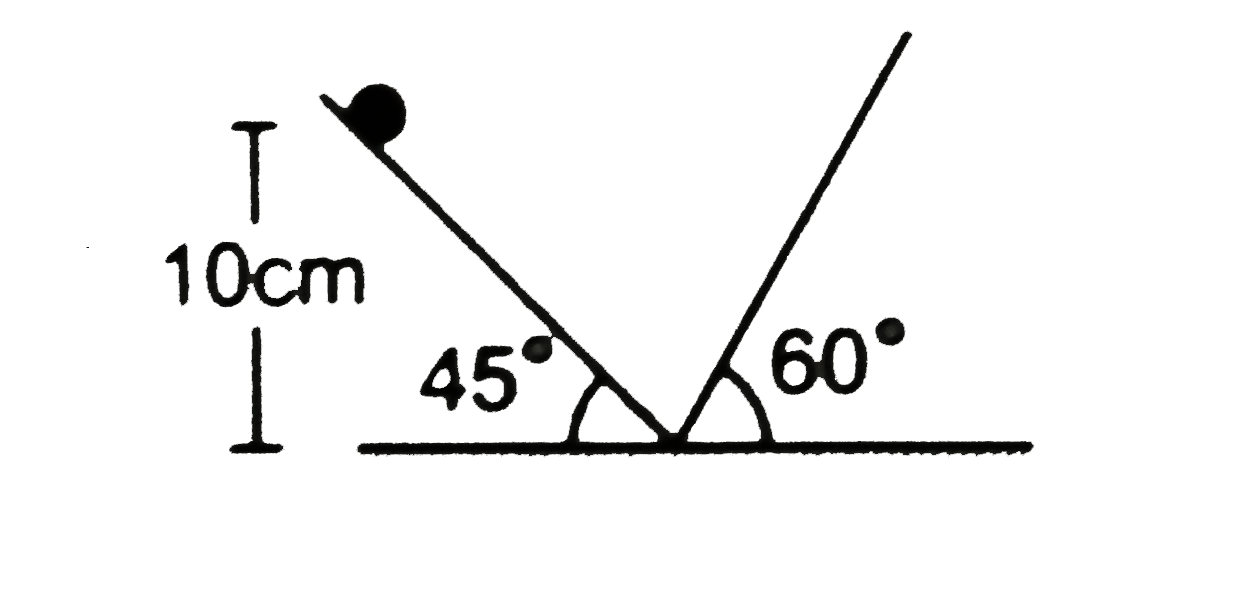View SolutionA pendulum suspended from the ceiling of the train has a time period of two seconds when the train is at rest, then the time period of the pendulum, if the train accelerates 10 m/s2 will be (g=10m/s2)
View SolutionAn electron in a hydrogen atom makes a transition from n1→n2. If the time period of electron in the initial state is eight times that in the final state then Find the ratio n1n2
View SolutionA H–atom in ground state has time period T=1.6×10–16 sec. find the frequency of electron in first excited state
View SolutionA H–atom in ground state has time period T=1.6×10–16 sec. find the frequency of electron in first excited state
View SolutionA stone is thrown horizontally with a velocity of 10m/sec. Find the radius of curvature of it’s trajectory at the end of 3s after motion began. (g=10m/s2)
View SolutionA stone is thrown horizontally with a velocity of 10m/sec. Find the radius of curvature of it’s trajectory at the end of 3s after motion began. (g=10m/s2)
View SolutionA small spherical ball (obeying Stoke's law for viscous force) is thrown up vertically with a speed 20ms−1 and is received back by the thrower at the point of projection with a speed 10ms−1. Neglecting the buoyant force on the ball, assuming the speed of the ball during its flight to be never equal to its terminal speed and taking the acceleration due to gravity g=10ms−2, find the time of flight of the ball in seconds.
View SolutionA small spherical ball (obeying Stoke's law for viscous force) is thrown up vertically with a speed 20ms−1 and is received back by the thrower at the point of projection with a speed 10ms−1. Neglecting the buoyant force on the ball, assuming the speed of the ball during its flight to be never equal to its terminal speed and taking the acceleration due to gravity g=10ms−2, find the time of flight of the ball in seconds.
View SolutionIf pressure at the half depth of a lake is equal to 34 times the pressure at its bottom, then find the depth of the lake. [Take g = 10 m/s2]
View Solution