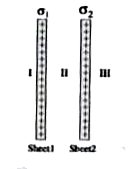
Figure shown two infinitely large conducting plates A and B. If electric field at C due to charge densities σ1,σ2,σ3 and σ40 is Eˆi, find σ2 and σ3 in terms of E. Derive a relation between σ1 and σ4.
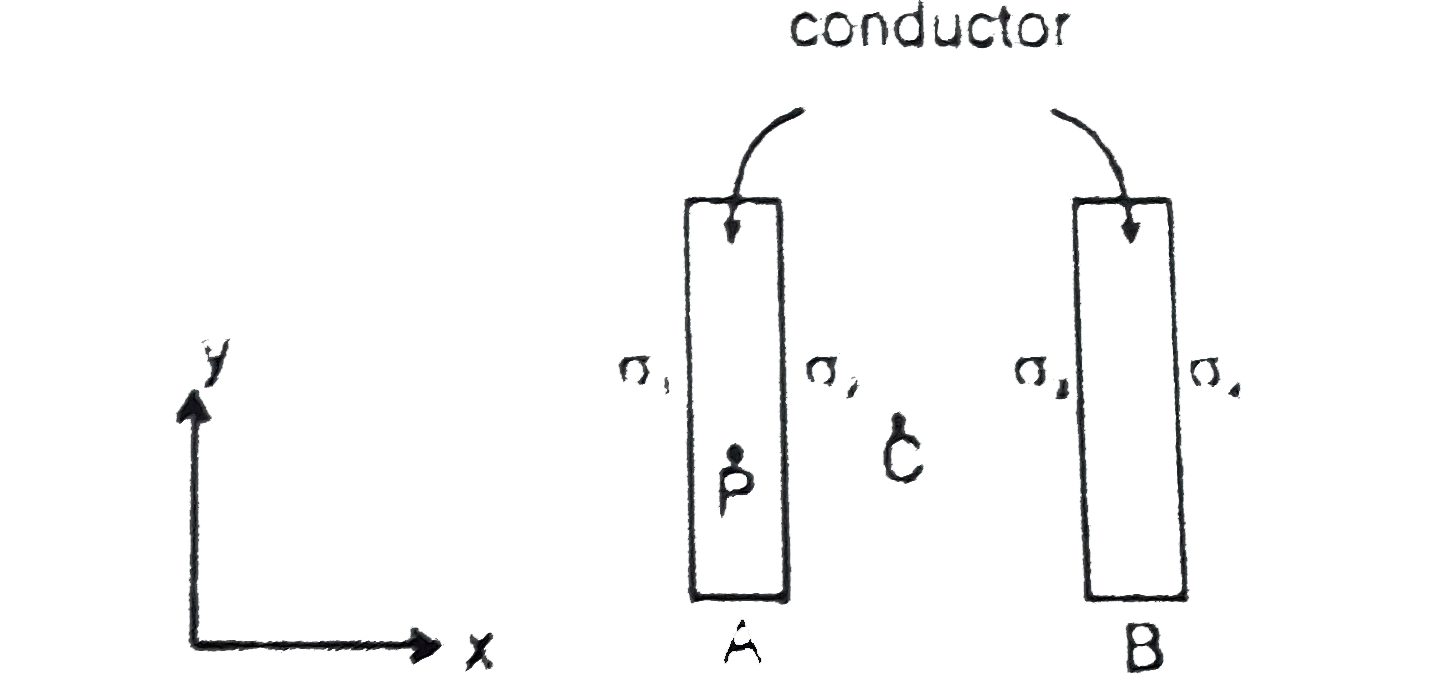
The correct Answer is:σ1=σ4,σ2=∈0E,σ3=−∈0E
Step by step video & image solution for Figure shown two infinitely large conducting plates A and B. If electric field at C due to charge densities sigma_(1), sigma_(2), sigma_(3) and sigma_(40 is E hat(i), find sigma_(2) and sigma_(3) in terms of E. Derive a relation between sigma_(1) and sigma_(4). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Part - II
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
Knowledge Check
If σx=3,σy=4, and bxy=13, then the value of r is
A14B94C49D4
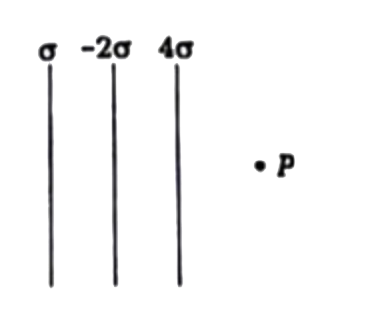
Three non-conducting large parallel plates have surface charge densities σ,−2σand4σ respectively as shown in the figure. The electric field at the point P is
View SolutionThe figure shows three infinite non-conducting plates of charge perpendicular to the plane of the paper with charge per unit area +σ,+2σ and −σ. Find the ratio of the net electric field at that point a to that at point B.
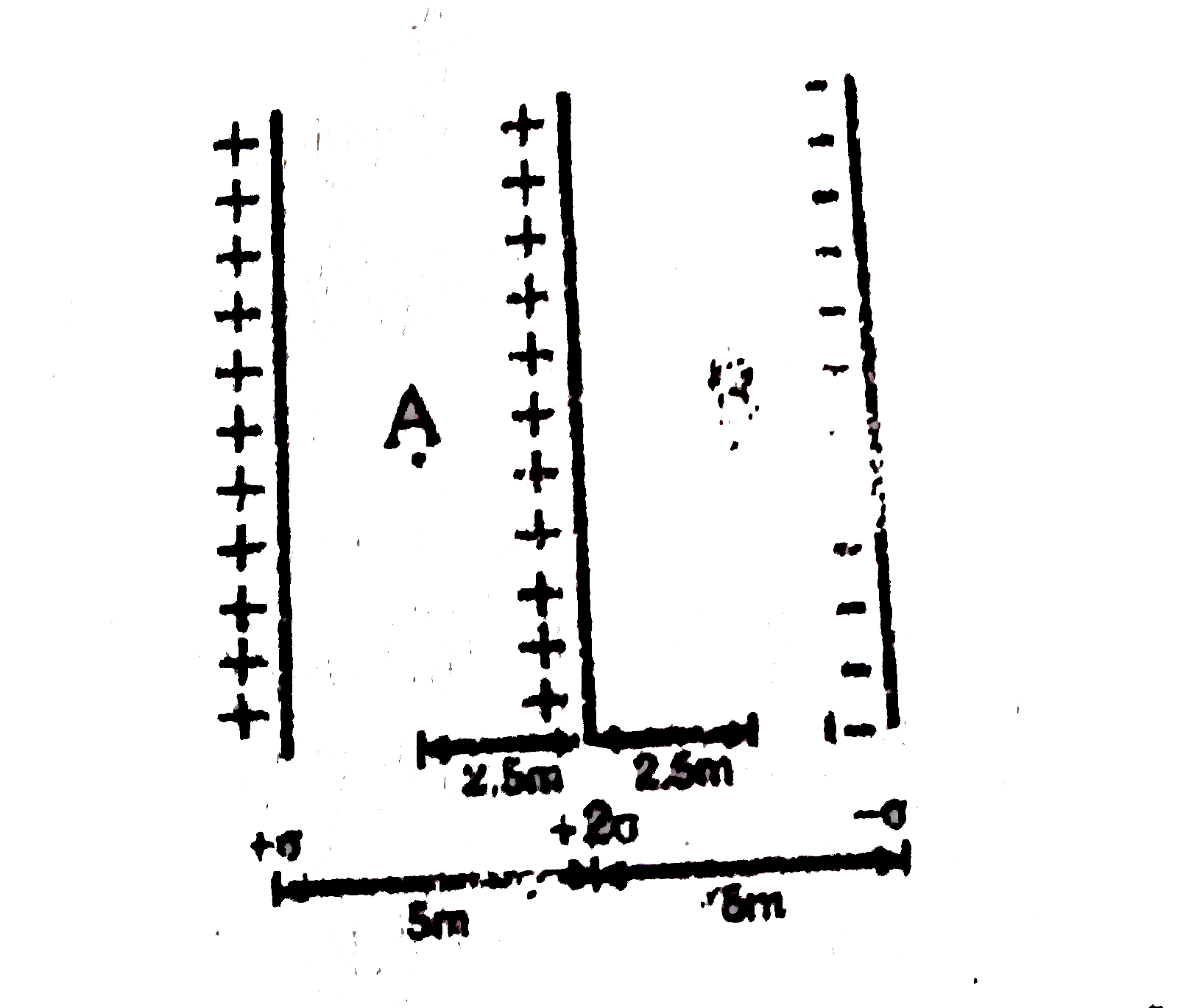
View SolutionIn the above question, the surface charge densities have the correct relation is-
A. σA>σB>σC>σD
B. σA=σB=σC=σD
C. σD>σC>σB>σA
D. σC<σB>σA>σD
View SolutionTwo teams A and B have the same mean and their coefficients of variance are 4, 2 respectively. If σA,σB are the standard deviations of teams A, B respectively then the relation between item is
View SolutionElectric field due to an infinite non-conducting sheet of surface charge density σ, at a distance r from it is
View SolutionTwo unequal blocks placed over each other of densities σ1 and σ2 are immersed in a fluid of density of σ. The block of density σ1 is fully submerged so that ratio of their masses is 12 and σ/σ1=2 and σ/σ2=0.5. Find the degree of submergence of the upper block of density σ2.
View SolutionFind the sum Σnj=1Σni=1I×3j
View Solution