A steel wire of length 1m, mass 0.1kg and uniform cross-sectional area 10−6m2 is rigidly fixed at both ends. The temperature of the wire is lowered by 20∘C. If transverse waves are set up by plucking the string in the middle.Calculate the frequency of the fundamental mode of vibration.
Given for steel Y=2×1011N/m2
α=1.21×10−5per∘C
The correct Answer is:11
To calculate the frequency of the fundamental mode of vibration of a steel wire that is fixed at both ends and has undergone a temperature change, we can follow these steps:
Step 1: Calculate the mass per unit length (μ) of the wire
The mass per unit length (μ) is given by the formula:
μ=mL
where:
- m=0.1kg (mass of the wire)
- L=1m (length of the wire)
Substituting the values:
μ=0.1kg1m=0.1kg/m
Step 2: Calculate the change in tension (T) in the wire due to temperature change
The tension in the wire can be calculated using Young's modulus (Y) and the change in length due to temperature change. The formula for tension is:
T=Y⋅strain
The strain can be calculated as:
strain=α⋅ΔT
where:
- α=1.21×10−5per ∘C (coefficient of linear expansion)
- ΔT=−20∘C (change in temperature)
Calculating strain:
strain=1.21×10−5⋅(−20)=−2.42×10−4
Now substituting into the tension formula:
T=Y⋅strain=(2×1011N/m2)⋅(−2.42×10−4)=−4.84×107N
(Note: The negative sign indicates a decrease in length, but tension is taken as a positive quantity.)
Step 3: Calculate the velocity (v) of the wave in the wire
The velocity of the wave is given by:
v=√Tμ
Substituting the values of T and μ:
v=√4.84×107N0.1kg/m=√4.84×108≈22040.3m/s
Step 4: Calculate the frequency (f) of the fundamental mode of vibration
The frequency of the fundamental mode of vibration is given by:
f=v2L
Substituting the values:
f=22040.3m/s2⋅1m=22040.32≈11020.15Hz
Final Result
The frequency of the fundamental mode of vibration is approximately:
f≈11020.15Hz
The mass per unit length (μ) is given by the formula:
μ=mL
where:
- m=0.1kg (mass of the wire)
- L=1m (length of the wire)
μ=0.1kg1m=0.1kg/m
The tension in the wire can be calculated using Young's modulus (Y) and the change in length due to temperature change. The formula for tension is:
T=Y⋅strain
The strain can be calculated as:
strain=α⋅ΔT
where:
- α=1.21×10−5per ∘C (coefficient of linear expansion)
- ΔT=−20∘C (change in temperature)
strain=1.21×10−5⋅(−20)=−2.42×10−4
T=Y⋅strain=(2×1011N/m2)⋅(−2.42×10−4)=−4.84×107N
(Note: The negative sign indicates a decrease in length, but tension is taken as a positive quantity.)
The velocity of the wave is given by:
v=√Tμ
Substituting the values of T and μ:
v=√4.84×107N0.1kg/m=√4.84×108≈22040.3m/s
The frequency of the fundamental mode of vibration is given by:
f=v2L
Substituting the values:
f=22040.3m/s2⋅1m=22040.32≈11020.15Hz
The frequency of the fundamental mode of vibration is approximately:
f≈11020.15Hz
Topper's Solved these Questions
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (FILL IN THE BLANKS)
17 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (MATRIX MATCH TYPE)
3 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
Similar Questions
A steel wire of length 20 cm and uniform cross-section 1mm2 is tied rigidly at both the ends. If the temperature of the wire is altered from 40∘C to 20∘C, the change in tension. [Given coefficient of linear expansion of steel is 1.1×105.∘C−1 and Young's modulus for steel is 2.0×1011Nm−2]
View SolutionDetermine the force required to double the length of the steel wire of area of cross-section 5×10−5m2. Give Y for steel =2×1011Nm−2.
View Solution
Knowledge Check
A steel wire of length 20 cm and uniform cross section 1 mm2 is tied rigidly at both the ends. The temperature of the wire is altered from 40∘ C to 20∘C Coefficient of linear expansion for steel α=1.1×10−5l∘C and Y for steel is 2.0×1011N/m2. The change in tension of the wire is
A2.2×106 newtonB16 newtonC8 newtonD44 newton
Calculate the speed of longitudinal wave in steel. Young's modulus for steel is 3×1010N/m2 and its density 1.2×103kg/m3
View SolutionCalculate the speed of longitudinal wave in steel. Young's modulus for steel is 3×1010N/m2 and its density 1.2×103kg/m3
View SolutionTo one end of a steel wire of unstretched length 3m and cross-section area 1mm2 a small ball of mass 150 gm is attached. The other end of the wire is fixed and the ball is allowed to revolved in a horizontal circle. If the ball makes 600 revolutions per minute find the radius of the uniform circular motion. Y for steel =2×1011Pa.
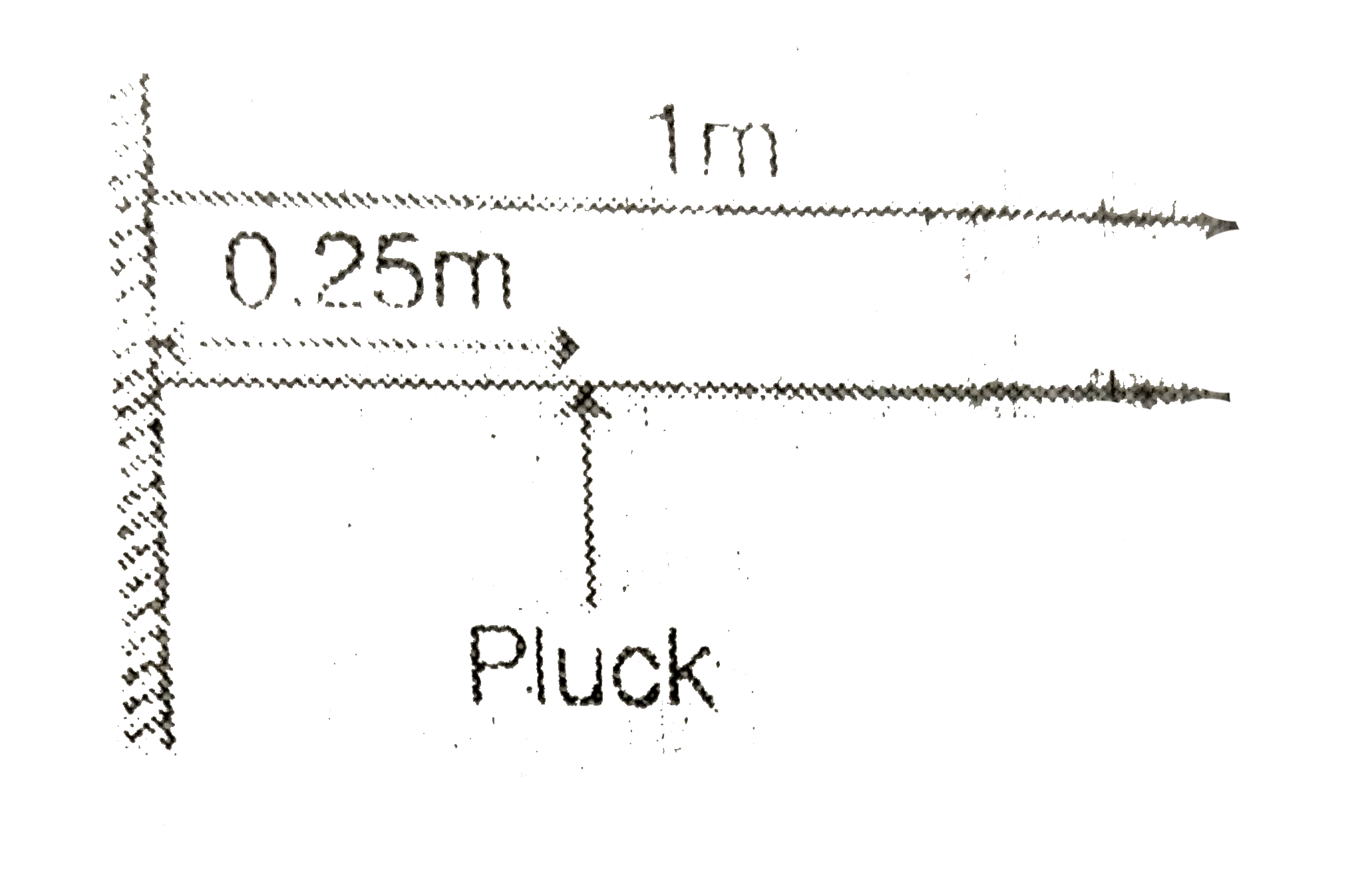
View SolutionA steel wire is rigidly fixed at both ends. Its length mass and cross-sectionl area are 1m, 0.1kg and 10−8m2 respectively. Tension in the wire is produced by lowering the temperature by 20∘C. If the transerverse waves are some up by plucking the wire at 0.25m from one end and assuming that the wire viberates with minimum number of loops possble for such a case. The frequency of viberation (in Hz) is found to be. 1.11. Find the value of K. Given α=1.21×10−5.∘C−1.Y−2×1011N/m2
View SolutionA steel wire of length 4.7m and cross-sectional area 3×10−6m2 stretches by the same amount as a copper wire of length 3.5m and cross-sectional area of 4×10−6m2 under a given load. The ratio of Young's modulus of steel to that of copper is
View SolutionA uniform wire of length 6m and a cross-sectional area 1.2 cm2 is stretched by a force of 600N . If the Young's modulus of the material of the wire 20×1010Nm−2 , calculate (i) stress (ii) strain and (iii) increase in length of the wire.
View SolutionA sonometer wire of length 1.5m is made of steel. The tension in it produces an elastic strain of 1%. What is the fundamental frequency of steel if density and elasticity of steel are 7.7×103kg/m3 and 2.2×1011N/m2 respectively ?
View Solution
