The axis X and Z in the plane of a disc are mutually perpendicular and Y-axis is perpendicular to the plane of the disc. If the moment of inertia of the body about X and Y axes is respectively 30 kg and 40 kg then M.I. about Z-axis in kg will be:
70
50
10
Zero
The correct Answer is:C
To find the moment of inertia about the Z-axis (I_z) of a disc given the moments of inertia about the X-axis (I_x) and Y-axis (I_y), we can use the perpendicular axis theorem.
1. Understand the Perpendicular Axis Theorem:
The perpendicular axis theorem states that for a planar body (like a disc), the moment of inertia about an axis perpendicular to the plane (I_z) is equal to the sum of the moments of inertia about two perpendicular axes (I_x and I_y) lying in the plane of the body. Mathematically, this can be expressed as:
Iz=Ix+Iy
2. Identify Given Values:
From the problem, we have:
- Moment of inertia about the X-axis (I_x) = 30 kg m²
- Moment of inertia about the Y-axis (I_y) = 40 kg m²
3. Apply the Perpendicular Axis Theorem:
Substitute the known values into the equation:
Iz=Ix+Iy
Iz=30kg m2+40kg m2
4. Calculate I_z:
Perform the addition:
Iz=30+40=70kg m2
5. Conclusion:
The moment of inertia about the Z-axis is:
Iz=70kg m2
Final Answer:
The moment of inertia about the Z-axis (I_z) is 70 kg m².
The perpendicular axis theorem states that for a planar body (like a disc), the moment of inertia about an axis perpendicular to the plane (I_z) is equal to the sum of the moments of inertia about two perpendicular axes (I_x and I_y) lying in the plane of the body. Mathematically, this can be expressed as:
Iz=Ix+Iy
From the problem, we have:
- Moment of inertia about the X-axis (I_x) = 30 kg m²
- Moment of inertia about the Y-axis (I_y) = 40 kg m²
Substitute the known values into the equation:
Iz=Ix+Iy
Iz=30kg m2+40kg m2
Perform the addition:
Iz=30+40=70kg m2
The moment of inertia about the Z-axis is:
Iz=70kg m2
The moment of inertia about the Z-axis (I_z) is 70 kg m².
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE
56 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ENABLE
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
The moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View SolutionThe M.I. of a ring of mass M and radius R about a tangential axis perpendicular to its plane is :
View SolutionA disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
View SolutionMoment of inertia of a disc about an axis which is tangent and parallel to its plane is I . Then the moment of inertia of disc about a tangent, but perpendicular to its plane will be
View SolutionA quarter disc of radius R and mass m is rotating about the axis OO' (perpendicular to the plane of the disc) as shown. Rotational kinetic energy of the quarter disc is

View SolutionFour point masses (each of mass m) are arranged it the X-Y plane the moment of inertia of this array of masses about Y-axis is
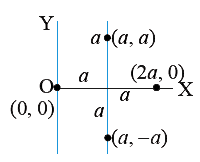
View SolutionA body having its centre of mass at the origin has three of its particles at (a,0,0),(0,a,0),(0,0,a). The moments of inertia of the body about the X and Y axes are 0.20 kg −m2 each. The moment of inertia about the Z-axis
View SolutionMoment of inertia of a disc about an axis parallel to diameter and at a distance x from the centre of the disc is same as the moment of inertia of the disc about its centre axis. The radius of disc is R. The value of x is
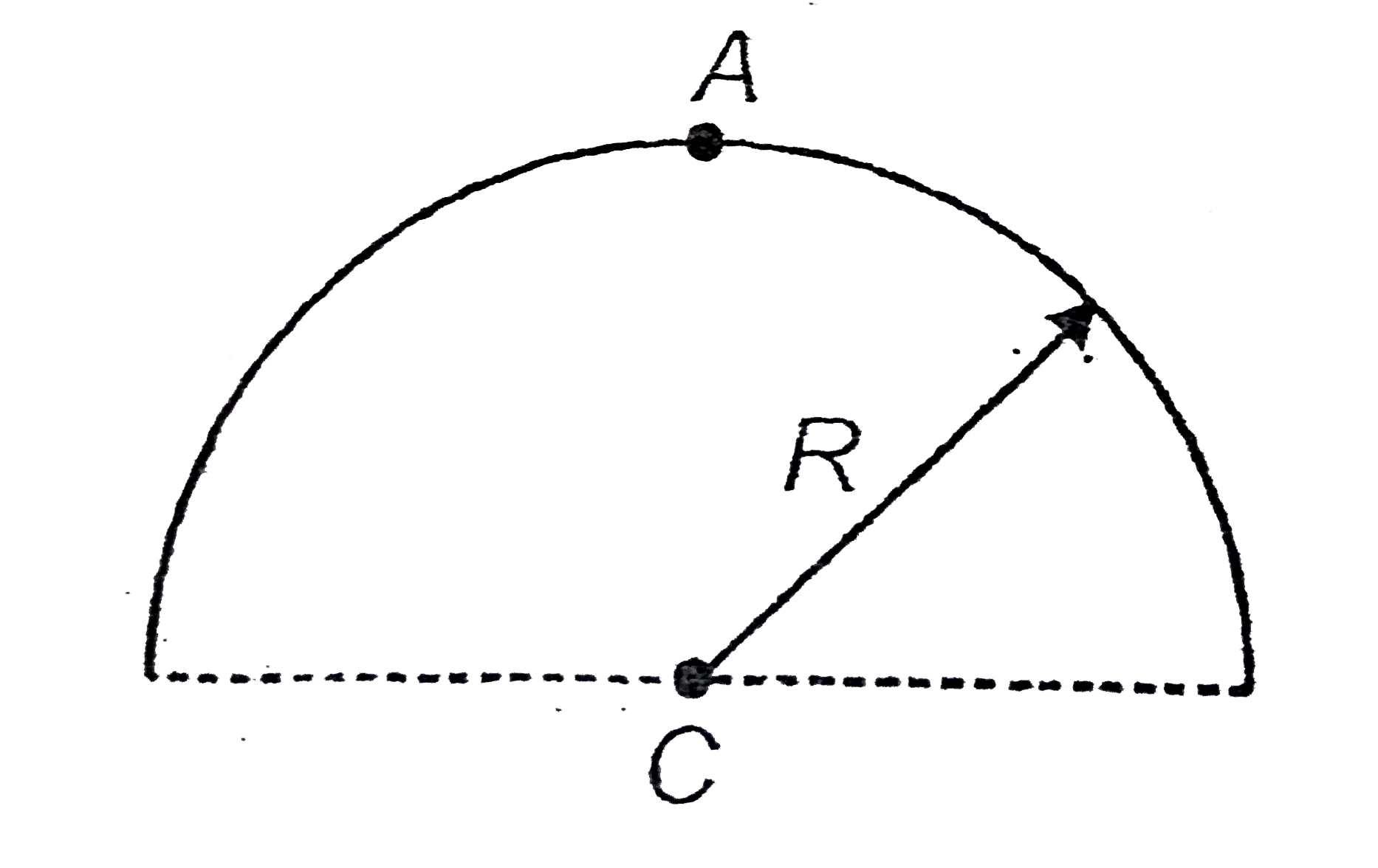
View SolutionMoment of inertia of a half ring of mass m and radius R about an axis passing through point A perpendicular to the plane of the paper is IA. If IC is the moment of inertia of the ring about an axis perpendicular to the plane of paper and passing through point C, then
View SolutionThree thin rods each of length Land mass M are placed along x, y and z-axes such that one end of each rod is at origin. The moment of inertia of this system about z-axis is
View Solution
