An LCR circuit behaves like a damped harmonic oscillator. Comparing it with a physical spring mass damped oscillator having damping constant b, the correct equivalence of b would be:
b↔R
b↔c
b↔1R
b↔1L
The correct Answer is:A
To solve the problem of finding the equivalence of the damping constant b in an LCR circuit compared to a physical spring-mass damped oscillator, we can follow these steps:
1. Understand the Damped Harmonic Oscillator:
- A damped harmonic oscillator can be described by the equation:
md2xdt2+bdxdt+kx=0
- Here, m is the mass, b is the damping constant, and k is the spring constant.
2. Write the Equation for the LCR Circuit:
- An LCR circuit consists of an inductor L, a capacitor C, and a resistor R. The equation governing the current I in the circuit is:
Ld2Qdt2+RdQdt+QC=0
- Here, Q is the charge on the capacitor.
3. Identify the Corresponding Terms:
- In the LCR circuit equation, the term RdQdt corresponds to the damping term in the mechanical oscillator equation. This is because both terms represent damping effects in their respective systems.
4. Establish the Equivalence:
- By comparing the coefficients of the first derivative terms in both equations, we can establish that:
b≡R
- This means that the damping constant b in the mechanical oscillator is equivalent to the resistance R in the LCR circuit.
5. Conclusion:
- Therefore, the correct equivalence of the damping constant b for the LCR circuit is:
b=R
- A damped harmonic oscillator can be described by the equation:
md2xdt2+bdxdt+kx=0
- Here, m is the mass, b is the damping constant, and k is the spring constant.
- An LCR circuit consists of an inductor L, a capacitor C, and a resistor R. The equation governing the current I in the circuit is:
Ld2Qdt2+RdQdt+QC=0
- Here, Q is the charge on the capacitor.
- In the LCR circuit equation, the term RdQdt corresponds to the damping term in the mechanical oscillator equation. This is because both terms represent damping effects in their respective systems.
- By comparing the coefficients of the first derivative terms in both equations, we can establish that:
b≡R
- This means that the damping constant b in the mechanical oscillator is equivalent to the resistance R in the LCR circuit.
- Therefore, the correct equivalence of the damping constant b for the LCR circuit is:
b=R
Topper's Solved these Questions
Similar Questions
An LCR series circuit behaves like a damped harmonic oscillator. Comparing it with a physical spring-mass damped oscillator having damping constant ‘b’, the correct equivalence would be (Take, i = current, V = voltage, x = displacement, q = charge, F = force, v = velocity)
View SolutionA LCR circuit behaves like a damped harmonic oscillator. Comparing it with a physical spring mass damped oscillator having damping constant ‘b’. If the amount of initial charge on the capacitor be Q0. then the amplitude of the amount of charge on the capacitor as a function of time t will be:
View SolutionA LCR circuit behaves like a damped harmonic oscillator. Comparing it with a physical spring mass damped oscillator having damping constant ‘b’. If the amount of initial charge on the capacitor be Q0. then the amplitude of the amount of charge on the capacitor as a function of time t will be:
View SolutionA LCR circuit be haves like a damped harmonic oscillator. The discharge of capacitor C, through an C□ inductor L having resistance R is oscillatory. Then which of following is correct _____.
View SolutionA LCR circuit be haves like a damped harmonic oscillator. The discharge of capacitor C, through an C□ inductor L having resistance R is oscillatory. Then which of following is correct _____.
View SolutionA rod of length l and mass m, pivoted at one end, is held by a spring at its mid - point and a spring at far end. The spring have spring constant k. Find the frequency of small oscillations about the equilibrium position.

View SolutionThere is a LCR circuit , If it is compared with a damped oscillation of mass m oscillating with force constant k and damping coefficient 'b'. Compare the terms of damped oscillation with the devices in LCR circuit.
View SolutionThe angular frequency of the damped oscillator is given by ω=√km−r24m2 ,where k is the spring constant, m is the mass of the oscillator and r is the damping constant. If the ratio r2/(mk) is 8% ,the change in the time period compared to the undamped oscillator
View SolutionYou are riding an automobile of mass 3000kg. Assuming that you are examining the oscillation characteristics of its suspension system. The suspension sags 15cm when the entire automobile is placed on it. Also, the amplitude of oscillation decreases by50% during one complete oscillation. Estimate the values of (a) the spring constant k and (b) damping constant b for the spring and shock absorber system of one wheel, assuming that each wheel supports 750kg.g=10m/s2.
View SolutionFind the time period of the oscillation of mass m in figure a,b,c what is the equivalent spring constant of the pair oif springs in each case?
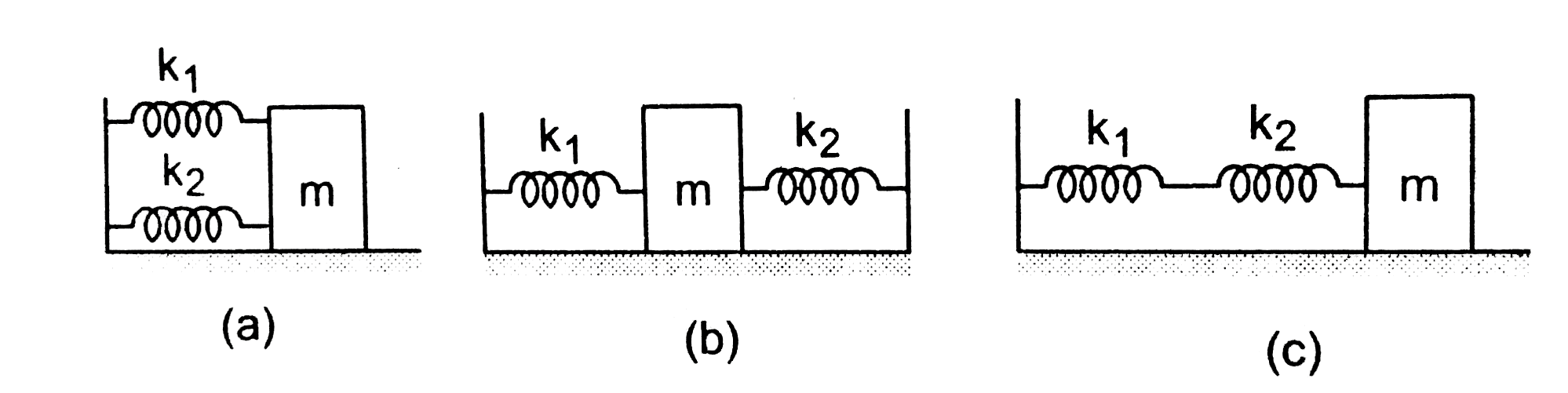
View Solution
