Question

A thin wire of radius ..r.. carries a charge q. Find the magnitude of the electric field strength on the axis of the ring as a function of distance L from the centre. Find the same for $L>>r$ Find maximum field strength and the corresponding distance L.

Explanation

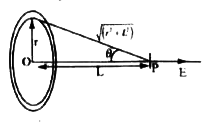
Due to a ring electric field strength at a distance ..L.. from its centre on it can be given as
`E= (qL)/(4pi epsi_(0)(L^(2) + r^(2))^(3//2)) rarr (1)`
For `L gt gt t` we have `E= (1)/(4pi in_(0))(q)/(L^(2))`
Thus the ring behaves like a point charge.
For `E_("Max") , (dE)/(dL) = 0`. From equation (1) we get
`(dE)/(dL) = (q)/(4pi epsi_(0)) [((r^(2) + L^(2))^(3//2)- (3)/(2) (r^(2) + L^(2))^(1//2)2L)/((r^(2) + L^(2))^(3))]=`
`(r^(2) + L^(2))^(3//2) = (3)/(2) (r^(2) + L^(2))^(1//2) xx 2L`
On solving we get `L = (r )/(sqrt2) rarr (2)`
Substituting the value of "L.. in equation (1) we get
`E= (1)/(4pi in_(0)) xx (q (r//sqrt2))/((r^(2) + r^(2)//2)^(3//2)) = (q)/(6 sqrt3 pi in_(0)r^(2))`