State whether the statements are true (T) or (F) false.
If diagonals of a quadrilateral bisect each other, it must be a parallelogram.
To determine whether the statement "If diagonals of a quadrilateral bisect each other, it must be a parallelogram" is true or false, we can analyze the properties of different types of quadrilaterals.
1. Understanding the Statement:
The statement claims that if the diagonals of any quadrilateral bisect each other, then that quadrilateral must be a parallelogram.
2. Definition of a Parallelogram:
A parallelogram is a quadrilateral where opposite sides are parallel and equal in length. One of the properties of parallelograms is that their diagonals bisect each other.
3. Exploring Other Quadrilaterals:
We need to consider whether there are any quadrilaterals other than parallelograms that also have diagonals that bisect each other.
4. Example of a Trapezium:
A trapezium (or trapezoid in some regions) is a quadrilateral with at least one pair of parallel sides. In certain cases, the diagonals of a trapezium can also bisect each other.
5. Conclusion:
Since we have found an example (the trapezium) where the diagonals bisect each other but the shape is not a parallelogram, we conclude that the original statement is false.
Final Answer:
The statement "If diagonals of a quadrilateral bisect each other, it must be a parallelogram" is False (F).
---
The statement claims that if the diagonals of any quadrilateral bisect each other, then that quadrilateral must be a parallelogram.
A parallelogram is a quadrilateral where opposite sides are parallel and equal in length. One of the properties of parallelograms is that their diagonals bisect each other.
We need to consider whether there are any quadrilaterals other than parallelograms that also have diagonals that bisect each other.
A trapezium (or trapezoid in some regions) is a quadrilateral with at least one pair of parallel sides. In certain cases, the diagonals of a trapezium can also bisect each other.
Since we have found an example (the trapezium) where the diagonals bisect each other but the shape is not a parallelogram, we conclude that the original statement is false.
The statement "If diagonals of a quadrilateral bisect each other, it must be a parallelogram" is False (F).
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
State whether the statements are true (T) or (F) false.
If diagonals of a quadrilateral are equal, it must be a rectangle.
View SolutionState whether the statements are true (T) or (F) false.
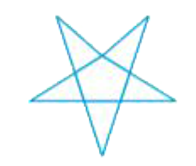 is a polygon.
is a polygon.
View SolutionState whether the statements are true (T) or (F) false.
If opposite angles of a quadrilateral are equal, it must be a parallelogram.
View SolutionState whether the statements are true (T) or (F) false.
A kite is not a convex quadrilateral.
View SolutionState whether the statements are true (T) or (F) false.
Diagonals of a rectangle are equal.
View SolutionState whether the statements are true (T) or (F) false.
Diagonals of rectangle bisect each other at right angles.
View SolutionState whether the statements are true (T) or (F) false.
Rectangle is a regular quadrilateral.
View SolutionIf the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
View SolutionState whether the statements are true (T) or (F) false.
Sum of all the angles of a quadrilateral is 180°.
View SolutionState whether the statements are true (T) or (F) false.
All kites are squares.
View Solution
