A line l is parallel to line m and a transversal p interesects them at X, Y respectively. Bisectors of interior angles at X and Y interesct at P and Q. Is PXQY a rectangle? Given reason.
To determine whether the quadrilateral PXQY is a rectangle, we need to analyze the properties of the angles formed by the parallel lines and the transversal. Here’s a step-by-step solution:
Step 1: Understand the Configuration
We have two parallel lines, L and M, and a transversal line P that intersects them at points X and Y respectively.
Hint: Draw two parallel lines and a transversal to visualize the situation.
Step 2: Identify the Angles
The angles formed at points X and Y are interior angles. Let’s denote the angles as follows:
- At point X: ∠DXY and ∠XYB
- At point Y: ∠CXY and ∠BYX
Hint: Label the angles clearly to avoid confusion.
Step 3: Use Angle Bisectors
The bisectors of the interior angles at X and Y intersect at points P and Q. This means:
- The angle bisector at X divides ∠DXY into two equal angles.
- The angle bisector at Y divides ∠CXY into two equal angles.
Hint: Remember that the angle bisector divides an angle into two equal parts.
Step 4: Establish Relationships Between Angles
Since lines L and M are parallel, the alternate interior angles are equal:
- ∠DXY = ∠BYX
- ∠CXY = ∠XYB
From the angle bisector properties, we can conclude:
- ∠PXY = ∠QYX (both are half of ∠DXY)
- ∠QXY = ∠PYX (both are half of ∠CXY)
Hint: Use the property of alternate interior angles to establish equality.
Step 5: Prove Parallel Sides
Since ∠PXY = ∠QYX and ∠QXY = ∠PYX, we can conclude that:
- Line PX is parallel to line QY (since they are cut by transversal XY).
- Line PY is parallel to line QX.
Hint: Parallel lines have equal corresponding angles.
Step 6: Check for Right Angles
Next, we need to show that all angles in quadrilateral PXQY are right angles. Since the sum of the angles at point X and Y is 180 degrees:
- ∠DXY + ∠XYB = 180°
Dividing this by 2 gives:
- (∠DXY/2) + (∠XYB/2) = 90°
Thus, ∠PXY + ∠PYX = 90° and ∠QXY + ∠QYX = 90°.
Hint: Use the property that adjacent angles on a straight line sum to 180 degrees.
Step 7: Conclusion
Since PX is parallel to QY and PY is parallel to QX, and all angles are right angles, we conclude that quadrilateral PXQY is a rectangle.
Final Answer: Yes, PXQY is a rectangle because opposite sides are parallel and all angles are right angles.
We have two parallel lines, L and M, and a transversal line P that intersects them at points X and Y respectively.
The angles formed at points X and Y are interior angles. Let’s denote the angles as follows:
- At point X: ∠DXY and ∠XYB
- At point Y: ∠CXY and ∠BYX
The bisectors of the interior angles at X and Y intersect at points P and Q. This means:
- The angle bisector at X divides ∠DXY into two equal angles.
- The angle bisector at Y divides ∠CXY into two equal angles.
Since lines L and M are parallel, the alternate interior angles are equal:
- ∠DXY = ∠BYX
- ∠CXY = ∠XYB
- ∠PXY = ∠QYX (both are half of ∠DXY)
- ∠QXY = ∠PYX (both are half of ∠CXY)
Since ∠PXY = ∠QYX and ∠QXY = ∠PYX, we can conclude that:
- Line PX is parallel to line QY (since they are cut by transversal XY).
- Line PY is parallel to line QX.
Next, we need to show that all angles in quadrilateral PXQY are right angles. Since the sum of the angles at point X and Y is 180 degrees:
- ∠DXY + ∠XYB = 180°
Dividing this by 2 gives:
- (∠DXY/2) + (∠XYB/2) = 90°
Since PX is parallel to QY and PY is parallel to QX, and all angles are right angles, we conclude that quadrilateral PXQY is a rectangle.
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
AB and CD are two parallel lines and a transversal 'l' intersects these lines at X and Y respectively Prove that the bisectors of interior angles from a parallelogram whose each angle is 90∘.
View SolutionIn Fig. 63, line l ∣∣ m and a transversal n cuts them at P and Q respectively. If ∠1=750, find all other angles.
View Solution
Knowledge Check
AB and CD are two parallel lines and a transversal PO intersects AB and CD at M and N respectively. The bisector of the interior angles form a quadrilateral :
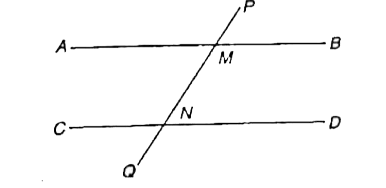 ArectangleBsquareCparallelogramDnone of these
ArectangleBsquareCparallelogramDnone of theseTwo parallel line l and m are intersected by a transversal p. The quadrilateral formed by the bisectors of interior angles is a
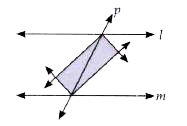 ATrapeziumBKiteCParallelogramDSquare
ATrapeziumBKiteCParallelogramDSquare
AB,CD are two parallel lines and a transversal l intersects AB at X and CD at Y Prove that the bisectors of the interior angles form a parallelogram, with all its angles right angles i.e., it is a rectangle.
View SolutionThe lines m and n are parallel and a transversal intersects them. Find the value of x,y and z
View SolutionIf two parallel lines are intersected by a transversal, prove that the bisectors of the two pairs of interior angles enclose a rectangle.
View SolutionIf two parallel lines intersected by a transversal; prove that the bisectors of the two pairs of interior angle encloses a rectangle.
View SolutionTwo parallel lines l and m are intersected by a transversal p (see Fig. 8.15). Show that the quadrilateral formed by the bisectors of interior angles is a rectangle.
View SolutionIf two parallel lines are intersected by a transversal then prove that the bisectors of any pair of alternate interior angles are parallel.
View Solution
