In △ABC ,∠A=90∘. If BL and CM are medians, then:
△ABC में, ∠A=90∘ है | यदि BL और CM मध्थिकाएं हैं, तो :
4(BL2+CM2)=3BC2
4(BL2+CM2)=5BC2
3(BL2+CM2)=4BC2
5(BL2+CM2)=4BC2
To solve the problem, we need to analyze triangle ABC where angle A is 90 degrees, and BL and CM are the medians from vertices B and C respectively. We will derive a relationship between the lengths of these medians and the sides of the triangle.
1. Identify the Triangle:
We have triangle ABC with angle A = 90°. Let's denote the lengths of the sides as follows:
- AB = c
- AC = b
- BC = a
2. Determine the Medians:
- The median BL from vertex B to side AC divides AC into two equal parts. Let L be the midpoint of AC.
- The median CM from vertex C to side AB divides AB into two equal parts. Let M be the midpoint of AB.
3. Use the Median Length Formula:
The length of a median in a triangle can be calculated using the formula:
ma=12√2b2+2c2−a2
where ma is the median from vertex A to side BC, and b and c are the lengths of the other two sides.
For our triangle:
- Median BL (from B to AC) is given by:
BL=12√2AB2+2BC2−AC2=12√2c2+2a2−b2
- Median CM (from C to AB) is given by:
CM=12√2AC2+2BC2−AB2=12√2b2+2a2−c2
4. Apply the Pythagorean Theorem:
Since triangle ABC is a right triangle, we can apply the Pythagorean theorem:
a2=b2+c2
5. Substitute and Simplify:
Substitute a2 in the median formulas:
- For BL:
BL2=(12√2c2+2a2−b2)2=14(2c2+2(b2+c2)−b2)=14(2c2+2b2+2c2−b2)=14(4c2+b2)
- For CM:
CM2=(12√2b2+2a2−c2)2=14(2b2+2(b2+c2)−c2)=14(2b2+2b2+2c2−c2)=14(4b2+c2)
6. Combine the Results:
Now we can express the relationship:
4BL2+4CM2=4(14(4c2+b2)+14(4b2+c2))=4c2+b2+4b2+c2=5(b2+c2)=5a2
7. Conclusion:
Therefore, we conclude that:
4BL2+4CM2=5BC2
Final Answer:
The correct relationship is 4BL2+4CM2=5BC2.
We have triangle ABC with angle A = 90°. Let's denote the lengths of the sides as follows:
- AB = c
- AC = b
- BC = a
- The median BL from vertex B to side AC divides AC into two equal parts. Let L be the midpoint of AC.
- The median CM from vertex C to side AB divides AB into two equal parts. Let M be the midpoint of AB.
The length of a median in a triangle can be calculated using the formula:
ma=12√2b2+2c2−a2
where ma is the median from vertex A to side BC, and b and c are the lengths of the other two sides.
- Median BL (from B to AC) is given by:
BL=12√2AB2+2BC2−AC2=12√2c2+2a2−b2
- Median CM (from C to AB) is given by:
CM=12√2AC2+2BC2−AB2=12√2b2+2a2−c2
Since triangle ABC is a right triangle, we can apply the Pythagorean theorem:
a2=b2+c2
Substitute a2 in the median formulas:
- For BL:
BL2=(12√2c2+2a2−b2)2=14(2c2+2(b2+c2)−b2)=14(2c2+2b2+2c2−b2)=14(4c2+b2)
- For CM:
CM2=(12√2b2+2a2−c2)2=14(2b2+2(b2+c2)−c2)=14(2b2+2b2+2c2−c2)=14(4b2+c2)
Now we can express the relationship:
4BL2+4CM2=4(14(4c2+b2)+14(4b2+c2))=4c2+b2+4b2+c2=5(b2+c2)=5a2
Therefore, we conclude that:
4BL2+4CM2=5BC2
The correct relationship is 4BL2+4CM2=5BC2.
Topper's Solved these Questions
Similar Questions
In a △ABC,∠A=90∘, if BM and CN are two medians, BM2+CN2BC2 is equal to:
△ABC में, ∠A=90∘, अगर BM और CN दो मध्यिका हैं, तो BM2+CN2BC2 बराबर है:
View SolutionIn △ABC, ∠ABC=90∘ and BD⊥AC. if AD=4 cm and CD=5 cm, then BD is equal to:
△ABC में, ∠ABC=90∘ और BD⊥AC है| यदि AD=4 cm और CD=5 cm तो BD किसके समान होगा?
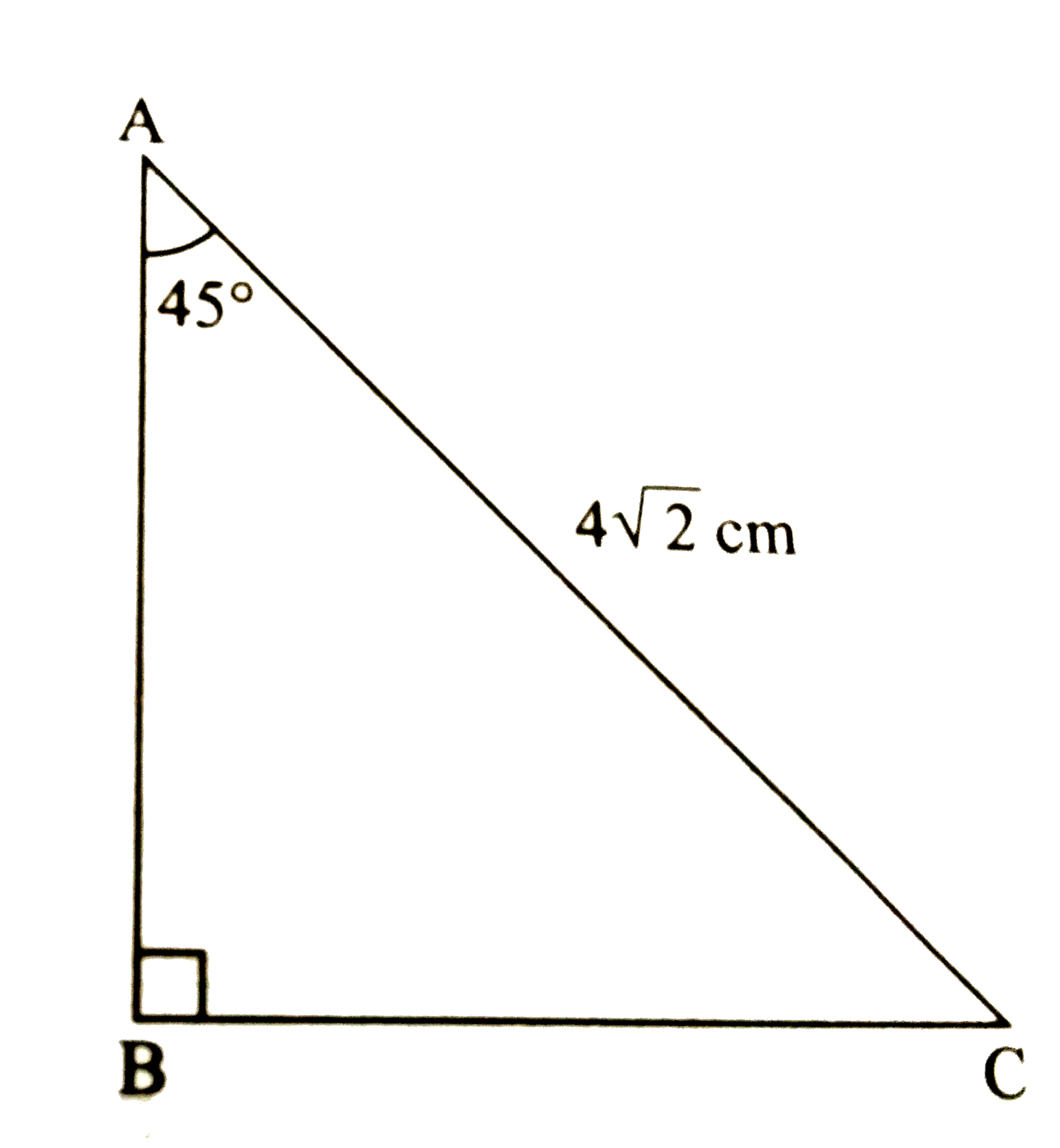
View SolutionIn △ABC,∠ABC=90∘
∠BAC=45∘ and AC =4√2cm,
then find AB.
View SolutionIna △ABC,∠BAC>90∘, then ∠ABC and ∠ACB must be :
View SolutionIn △ABC ,∠A=54∘. If I is the incentre of the triangle, then the measure of ∠BIC is:
त्रिभुज ABC में, ∠A=54∘ है |यदि I त्रिभुज का अंतःकेंद्र है, तो ∠BIC का माप है
View SolutionIn △ABC ,∠ABC=90∘ and BD⊥AC. If AD = 4 cm and CD =5 cm, then BD is equal to:
त्रिभुज ABC में, ∠ABC=90∘ तथा BD⊥AC है | यदि AD =4 सेमी और CD = 5सेमी है, तो BD किसके बराबर है ?
View SolutionIn △ABC ,∠A=90∘. M is the midpoint of BC and D is a point on BC such that AD⊥BC. If AB= 7 cm and AC = 24 cm, then AD:AM is equal to:
△ABC में, ∠A=90∘ है, M, BC का मध्य बिंदु है तथा D, BC पर स्थित ऐसा बिंदु है कि AD⊥BC है | यदि AB= 7 सेमी, AC = 24 सेमी है, तो AD:AM किसके बराबर है ?
View SolutionIn △ABC ,∠A=58∘. If I is the incentre of the triangle, then the measure of ∠BIC is:
/ त्रिभुज ABC में, ∠A=58∘है | यदि I त्रिभुज का अंतःकेंद्र है, तो कोण BIC का मान ज्ञात करें |
View SolutionBL and CM are medians of a triangle ABC right angled at A. Prove that 4(BL2+CM2)=5BC2
View SolutionIn △ABC, ∠A=90∘, AD is the bisector of ∠A meeting BC at D and DE⊥AC at E. If AB =10cm and AC = 15 cm, then the length of DE, in cm is:
△ABCमें ∠A=90∘, AD, ∠A का द्विभाजक है, जो BC से D पर मिलता है, और DE⊥AC से E पर मिलता है। यदि AB = 10 cm और AC= 15 cm है, तो DE की लंबाई(cm) है,
View Solution
